question_answer

If \[PQ\] is a double of the .hyperbola\[\frac{{{x}^{2}}}{{{a}^{2}}}-\frac{{{y}^{2}}}{{{b}^{2}}}=1\] such that \[OPQ\] is an equilateral triangle, \[O\] being the centre of the hyperbola, then the eccentricity \['e'\] of the hyperbola satisfies

A) \[1<e<\frac{2}{\sqrt{3}}\]

B) \[e=\frac{2}{\sqrt{3}}\]

C) \[e=\frac{\sqrt{3}}{2}\]

D) \[e>\frac{2}{\sqrt{3}}\]

Correct Answer: B

Solution :

Let the hyperbola be \[\frac{{{x}^{2}}}{{{a}^{2}}}-\frac{{{y}^{2}}}{{{b}^{2}}}=1\] and any double ordinate \[PO\] be\[(a\sec \theta ,\,\,b\tan \theta ),\,\,(a\sec \theta ,\,\,-b\tan \theta )\] and \[O\] is centre\[(0,\,\,0)\]. \[\Delta OPQ\]being equilateral.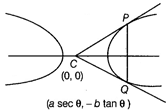 \[\Rightarrow \]               \[\tan {{30}^{o}}=\frac{b\tan \theta }{a\sec \theta }\] \[\Rightarrow \]               \[3\frac{{{b}^{2}}}{{{a}^{2}}}=\cos \text{e}{{\text{c}}^{2}}\theta \] \[\Rightarrow \]               \[3({{e}^{2}}-1)=\cos \text{e}{{\text{c}}^{2}}\theta \] Now,     \[\cos \text{e}{{\text{c}}^{2}}\theta \ge 1\] \[\Rightarrow \]               \[3({{e}^{2}}-1)\ge 1\] \[\Rightarrow \]               \[{{e}^{2}}\ge 4/3\] \[\Rightarrow \]               \[e>\frac{2}{\sqrt{3}}\]