Category : 11th Class
Pair and Straight Line
Key Points to Remember
A hemogenous of equation of second degree of the form \[a{{x}^{2}}+2hxy+b{{y}^{2}}=0\] represents the pair of straight lines which passes through the origin.
(a) If the lines are real and distinct then
\[{{h}^{2}}>ab\]
(b) If the lines are real and coincidents if
\[{{h}^{2}}=ab.\]
(c) If the lines are imaginary then \[{{h}^{2}}<ab.\]
Let \[y={{m}_{1}}x\to (1)\] and \[y={{m}_{2}}x\to (2)\] are two lines which are passing through the origin.
Then \[(y-{{m}_{1}}x)(y-{{m}_{2}}x)\equiv a{{x}^{2}}+2hxy+b{{y}^{2}}\]
\[{{y}^{2}}-({{m}_{1}}+{{m}_{2}})xy+{{m}_{1}}{{m}_{2}}{{x}^{2}}={{y}^{2}}+\frac{2h}{b}xy+\frac{a}{b}{{x}^{2}}\]
Equation the coefficient of same variable of the\[\frac{2h}{b}=({{m}_{1}}+{{m}_{2}})\]we have, \[({{m}_{1}}+{{m}_{2}})=\frac{2h}{b}\] & \[{{m}_{1}}.{{m}_{2}}=\frac{a}{b}\]
Let q be the angle between the two given pair of straight line \[a{{x}^{2}}+2hxy+b{{y}^{2}}=0,\] which are passing through origin is written as
\[\tan \theta =\pm \frac{2\sqrt{{{h}^{2}}}-ab}{a+b}\]
for acute angle,
\[\tan \theta =\left| \frac{2\sqrt{{{h}^{2}}}-ab}{a+b} \right|\]
In cosine form
\[\cos \theta =\frac{a+b}{\sqrt{{{(a+b)}^{2}}+4{{h}^{2}}}}\]
Note: If two lines are coincident
i.e. \[\theta =0{}^\circ \] or \[180{}^\circ \]
then \[\frac{2\sqrt{{{h}^{2}}-ab}}{a+b}=0\]
Hence \[{{h}^{2}}=ab\Rightarrow {{h}^{2}}=a\]
(b) If two lines are perpendicular
i.e. \[\theta =90{}^\circ ,\] i.e. \[\tan 90{}^\circ =\infty \]
then Loosly, it can be written
\[\frac{2\sqrt{{{h}^{2}}-ab}}{a+b}=\infty =\frac{1}{0}\]
\[a+b=0\] i.e.
\[\therefore \]Sum of the coefficient of \[{{x}^{2}}\] and \[{{y}^{2}}\] respectively is zero.
(c) The equation of pair of straight lines passing through the origin and perpendicular to the given equation of pair of straight lines\[/a{{x}^{2}}+2hxy+b{{y}^{2}}=0\] is written as \[b{{x}^{2}}-2hxy+a{{x}^{2}}=0\]
The general equation of second degree in x and y be
\[a{{x}^{2}}+b{{y}^{2}}+2hxy+2gx+2fy+c=0\] …….(i)
represents a pair of straight lines iff
\[abc+2fgx-a{{f}^{2}}-b{{g}^{2}}-c{{h}^{2}}=0\]
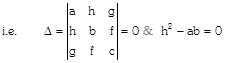
(a) If \[\Delta \ne 0\] & \[{{h}^{2}}-ab\ge 0\] then this general equation of second degree in x & y represents the equation of hyperbola.
(b) If \[\Delta \ne 0\] & \[{{h}^{2}}-ab\le 0\] then this pair of lines represent the equation ellipse.
(c) If \[\Delta \ne 0\] & \[{{h}^{2}}-ab=0\] then this pair of lines represent the equation of parabola
(d) If \[a=b=1\] & \[h=0\] then this represents the equation of circle.
(a) Angle between the lines:-
If \[\theta \] is the angle between the two lines:
\[a{{x}^{2}}+2hxy+b{{y}^{2}}+2gx+2fy+c=0\] …… (1)
then \[\tan \theta =\pm \left| \frac{2\sqrt{{{h}^{2}}-ab}}{a+b} \right|\]
\[\Rightarrow \,\,\,\theta ={{\tan }^{-1}}\left| \frac{2\sqrt{{{h}^{2}}-ab}}{a+b} \right|\]
Note: It is the same form which is obtained by the pair of straight lines passing through the origin.
(b) Point of intersection of lines:
The point of intersection of line (1) is obtained by the partially differentiation of
\[\text{f}\equiv a{{x}^{\text{2}}}+b{{y}^{2}}+2hxy+2gx+2\text{f}y+c=0\]
w.r.t x and y respective & making
\[\frac{\partial \text{f}}{\partial x}=0\] ….. (i)
& \[\frac{\partial \text{f}}{\partial y}=0\] ….. (ii)
Then solve these equations and hence, we will obtain the value ofx and y, which are written as
\[(x,y)=\left( \frac{bg-h}{{{h}^{2}}-ab},\frac{a\text{f-gh}}{{{h}^{2}}-ab} \right)\]
Here,
\[\frac{\partial \text{f}}{\partial x}=2ax+2hy+2g=0\] & \[\frac{\partial \text{f}}{\partial x}=2by+2hx+2\text{f}=0\]
If the two lines represented by equation (1) be parallel if \[{{h}^{2}}=ab\] & \[b{{g}^{2}}=a{{\text{f}}^{\text{2}}}\]
\[d=2\sqrt{\frac{{{g}^{2}}-ac}{a(a+b)}}\]
The equation to the pair of lines through the origin and forming an equiletral triangle with line
\[ax+by+c=0\]is given by \[{{(ax+by)}^{2}}-3{{(ay-bx)}^{2}}=0\]
Also, the area of equiletral triangle is
\[=\frac{{{c}^{2}}}{\sqrt{3}({{a}^{2}}+{{b}^{2}})}\]
The line \[a{{x}^{2}}+b{{y}^{2}}+2hxy=0\] & \[\ell \times +my+n\]
= 0 'form issosectes triangle if
\[\frac{{{\ell }^{2}}-{{m}^{2}}}{\ell m}=\frac{a-b}{h}\]
Area of the triangle = \[\left| \frac{{{n}^{2}}\sqrt{{{h}^{2}}-ab}}{a{{m}^{2}}-2h\ell m+b{{\ell }^{2}}} \right|\]
\[\frac{{{x}^{2}}-{{y}^{2}}}{a-b}=\frac{xy}{h}\]
Let the straight line is
\[y=mx+c\]
\[\Rightarrow y-mx=c\Rightarrow \frac{y-mx}{c}=1\]
& The equation of the curve is
\[a{{x}^{2}}+2hxy+b{{y}^{2}}+2gx+2\text{f}y+K=0\]
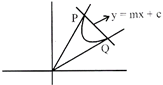
Let the line cuts the curve at point P & Q then the joint equation of OP & OQ be written as
\[a{{x}^{2}}+2hxy+b{{y}^{2}}+(2gx+2\text{f}y)+K{{\left( \frac{y-mx}{c} \right)}^{2}}=0\]
From this method we can make any pair of equation to be homogenous with the help of the equation of the line.
You need to login to perform this action.
You will be redirected in
3 sec
