Current Affairs 12th Class

Category : 12th Class

 

Integral Calculus

 

Integration is the inverse process of differentiation i.e. the process of finding out the integral of the integrand function is called integration.

e.g. If \[\frac{d}{dx}\{F(x)\}=f(x),\]then\[\int{f(x).dx=F(x)+C}\]

Here function f(x) is said to be integrand and value of\[\int{f(x).dx=F(x)}\] is said to be integral value the function, \[c=\] Integration constant

 

• Some Basic Properties of Indefinite Integration

 

Derivatives		Integrals:
1.	\[\frac{d}{dx}({{x}^{n}})=n.{{x}^{n-1}}\]	\[\int{{{x}^{n}}.dx=\frac{{{x}^{n}}+1}{n+1}+c}\] where \[c=\]integration constant.
2.	\[\frac{d}{dx}({{e}^{x}})={{e}^{x}}\]	\[\int{{{e}^{x}}.dx={{e}^{x}}+c}\]
3.	\[\frac{d}{dx}\{\log (x)\}=\frac{1}{x}\]	\[\int{\frac{1}{x}.dx=\log x+c}\]
4.	\[\frac{d}{dx}({{a}^{x}})={{a}^{x}}.\log a\]	\[\int{{{a}^{x}}.dx=\frac{{{a}^{x}}}{\log a}+c}\]
5.	\[\frac{d}{dx}(\sin x)=\cos x\]	\[\int{\cos x.dx=\sin x+c}\]
6.	\[\frac{d}{dx}(cosx)=-\sin x\]	\[\int{\sin xdx=-\cos x+c}\]
7.	\[\frac{d}{dx}(tanx)={{\sec }^{2}}x\]	\[\int{{{\sec }^{2}}xdx=\tan x+c}\]
8.	\[\frac{d}{dx}(cotx)=-\cos e{{c}^{2}}x\]	\[\int{\cos e{{c}^{2}}xdx=-\cot x+c}\]
9.	\[\frac{d}{dx}(secx)=\sec x.\tan x\]	\[\int{\sec x.\tan x.dx=\sec x+c}\]
10.	\[\frac{d}{dx}(cosecx)=-\cos ecx.\cot x\]	\[\int{\cos ecx.cotx.dx=-\cos ec\,x+c}\]
11.	\[\frac{d}{dx}(ta{{n}^{-1}}x)=\frac{1}{1+{{x}^{2}}}\]	\[\int{\frac{1}{1+{{x}^{2}}}.dx={{\tan }^{-1}}x+c}\]
12.	\[\frac{d}{dx}(si{{n}^{-1}}x)=\frac{1}{\sqrt{1-{{x}^{2}}}}\]	\[\int{\frac{1}{\sqrt{1-{{x}^{2}}}}.dx={{\sin }^{-1}}x+c}\]
13.	\[\frac{d}{dx}(co{{s}^{-1}}x)=\frac{-1}{\sqrt{1-{{x}^{2}}}};\]	\[\int{\frac{1}{\sqrt{1-{{x}^{2}}}}=-{{\cos }^{-1}}x+c}\]
14.	\[\frac{d}{dx}(ta{{n}^{-1}}x)=\frac{1}{1+{{x}^{2}}};\]	\[\int{\frac{dx}{1+{{x}^{2}}}={{\tan }^{-1}}x+c}\]
15.	\[\frac{d}{dx}(-co{{t}^{-1}}x)=\frac{1}{1+{{x}^{2}}};\]	\[\int{\frac{1}{1+{{x}^{2}}}dx=-{{\cot }^{-1}}x+c}\]
16.	\[\frac{d}{dx}(se{{c}^{-1}}x)=\frac{1}{x\sqrt{{{x}^{2}}-1}};\]	\[\int{\frac{1}{x\sqrt{{{x}^{2}}-1}}.dx={{\sec }^{-1}}x+c}\]
17.	\[\frac{d}{dx}(-cose{{c}^{-1}}x)=\frac{1}{x\sqrt{{{x}^{2}}-1}};\]	\[\int{\frac{dx}{x\sqrt{{{x}^{2}}-1}}=-\cos e{{c}^{-1}}x+c}\]

 

• Method of Integration: The different methods of doing integration are as follows:

1. Method of transformation
2. Method of substitution
3. Method of Integration by parts

 

• In method of transformation, we need to remember some basic trigonometry formulae that are being given below.

1. \[{{\sin }^{2}}x=\frac{1-\cos 2x}{2}\]
2. \[{{\cos }^{2}}x=\frac{1+\cos 2x}{2}\]
3. \[\sin 2x=2.\sin x\,\,\cos x\]
4. \[{{\sin }^{3}}x=\frac{3\sin x-\sin 3x}{4}\]
5. \[{{\cos }^{3}}x=\frac{3\cos x+\cos 3x}{4}\]
6. \[{{\tan }^{2}}x={{\sec }^{2}}x-1\]
7. \[{{\cot }^{2}}x=\cos e{{c}^{2}}x-1\]
8. \[2.\sin A.cosB=sin(A+B)+sin(A-B)\]
9. \[2.cosA.cosB=\cos (A+B)+\cos (A-B)\]
10. \[2.sinA.sinB=\cos (A-B)-\cos (A+B)\]

 

 

• Integration by substitution: The given integral \[\int{f(x).dx}\] can be transformed into another form by changing the independent variable x to t.

By substituting \[x=g(t).\]

\[\because \]   Let us consider, \[I=\int{f(x).dx}\]

Putting \[x=g(t)\]then\[dx=g'(t).dt\]\[\Rightarrow I=\int{f\{g(t)\}.g'(t).dt}\]

This change of variable formula is one of the important tools available to us for integrating various functions.

 

• Some other Important Formulae of Integration

1. \[\int{\tan x.dx=\log |\sec x|+c=-}\log (|\cos x|+\,\,c\]
2. \[\int{\cot x.dx=\log \sin x+c}\]
3. \[\int{\sec x.dx=\log |\sec x+\tan x|+c=\log \tan \left( \frac{x}{2}+\frac{\pi }{4} \right)}+c\]
4. \[\int{\cos ecx.dx=\log |cosecx-\cot x|+c=\log \tan \left( \tan \frac{x}{2} \right)}+c\]
5. \[\int{\frac{1}{f(x)}.f'(x).dx=\log f(x)+c=}\]
6. \[\int{{{e}^{x}}\{f'(x)}+f(x)\}.dx={{e}^{x}}.f(x)+c\]

 

• Solved Example

1. Find the value of \[\int{{{e}^{x}}(\tan x+\sec x+\sec x.\tan x+{{\sec }^{2}}x}).dx\,.\]

Sol. Let

\[I=\int{{{e}^{x}}(\tan x+\sec x+\sec x.\tan x+{{\sec }^{2}}x}).dx\]

Putting\[f(x)=\tan x+\sec x,\]we get

\[f'(x)={{\sec }^{2}}x+\sec x.\tan x\]

\[\therefore I=\int{{{e}^{x}}\{f(x)+f'(x)}\}dx={{e}^{x}}.f(x)+c={{e}^{x}}(\tan x+\sec x)+c\]

 

• Method of Partial Fraction for Rational Functions: Integral of the form\[\int{\frac{p(x)}{q(x)}.dx}\]can be integrated by revolving the integrand into partial fraction. We proceed as follows:

Check the degree of \[p(x)\] & \[q(x).\]

If the degree of \[p(x)>\] degree of \[q(x),\] then divide the \[p(x)\] by \[g(x)\] till its degree becomes less than the degree of \[g(x).\]

i.e. put in the form

\[\frac{p(x)}{q(x)}=r(x)+\frac{f(x)}{g(x)}\]

where degree of \[f(x)<\] degree of \[g(x)\]

Case-I: When the denominator contains non-repeated linear factors i.e., \[g(x)=(x-{{a}_{1}})(x-{{a}_{2}})......(x-{{a}_{n}})\]

Then\[\frac{f(x)}{g(x)}\]can be written as

\[\frac{f(x)}{g(x)}=\frac{{{A}_{1}}}{(x-{{a}_{1}})}+\frac{{{A}_{2}}}{(x-{{a}_{2}})}+.......+\frac{{{A}_{n}}}{(x-{{a}_{n}})}\]

Where\[{{A}_{1}}{{A}_{2}}.....{{A}_{n}}\] are constant to be determined by comparing the coefficient of various power of x on both side & taking L.C.M.

 

• Method of Integration by Parts: The process of finding out the integral of the product of two function is known as integration by parts.

e.g. Let u & v be two function of x.

Then \[\int{u.\text{v}.dx=u\int \text{v}\text{.}dx-\int \left[ \frac{du}{dx}.\int \text{v}.dx \right]}dx\]

i.e. integration of product of two functions= 1st function \[\times \] integral of 2nd function \[-\] integral of product of differentiation of 1st & integral of 2nd function.

For applying this formula, we may have to decide which function should be 1st & 2nd function. Keep remember the following tips and you will have no difficulties to choose the 1st & 2nd function.

To choose the 1st function use I LATE formula:

I for inverse function

L for Logarithmic function

A for Algebraic function

T for Trigonometric function

E for Exponential function

It means if we have two functions from which one is inverse trigonometric and other is logarithmic, then inverse trigonometric function will be the first function.

 

Solved Example

1. Find the value of \[\int{x.{{e}^{x}}.dx.}\]

            Sol. Let \[I=\int{x.{{e}^{x}}.dx.}\]

            Using ILate

A come first before E.

So, x will be the first function because it is algebric function & \[{{e}^{x}}\] be 2nd function because it is exponential term.

Now, \[I=\int{x.{{e}^{x}}.dx.}\]

\[=x.\int {{e}^{x}}.dx-\int \left\{ \left[ \frac{d}{dx}(x) \right].\int {{e}^{x}}.dx \right\}.dx=x.{{e}^{x}}-\int{1.{{e}^{x}}.dx=x.}\]\[{{e}^{x}}-{{e}^{x}}+c\]

\[={{e}^{x}}(x-1)+c\]

 

• Some Important Results

1. \[\int{\frac{2x}{{{x}^{2}}}.dx=\log {{x}^{2}}+c}\]
2. \[\int{{{e}^{x}}(\tan x+{{\sec }^{2}}x)dx={{e}^{x}}.\tan x+c}\]
3. \[I=\int{\frac{1}{1+\tan x}.dx}=\int{\frac{1}{1+\frac{\sin x}{\cos x}}.dx=\int{\frac{\cos x}{\sin x+\cos x}.dx}}\]

\[=\frac{1}{2}\int{\frac{\sin x+\cos x+(\cos x-\sin x)}{(\sin x+\cos x)}.dx}\]

Where \[f(x)=\sin x+\cos x=\frac{1}{2}[x+\log (\sin x+\cos x)]+c\]

4. \[I=\int{\frac{x}{{{e}^{{{x}^{2}}}}}.dx}\]\[I=\frac{1}{2}\int{\frac{2x}{{{e}^{{{x}^{2}}}}}.dx}\]

Now, putting \[{{x}^{2}}=z.\]

\[\therefore dz=2x.dx\]

\[I=\frac{1}{2}\int{\frac{dz}{{{e}^{z}}}=\frac{1}{2}\int{{{e}^{-z}}.dz=\frac{-{{e}^{-z}}}{2}+c}=\frac{-1}{2.{{e}^{+z}}}+c=\frac{-1}{2.{{e}^{x}}^{^{2}}}}+c\]

5. \[\int{\frac{dx}{\sqrt{{{x}^{2}}-{{a}^{2}}}}=\log (x+\sqrt{{{x}^{2}}-{{a}^{2}}})+c}\]
6. \[\int{\frac{dx}{\sqrt{{{x}^{2}}}+{{a}^{2}}}=\log (x+\sqrt{{{x}^{2}}+{{a}^{2}}})+c}\]
7. \[\int{\sqrt{{{a}^{2}}-{{x}^{2}}}.dx}=\frac{x}{2}.\sqrt{{{a}^{2}}-{{x}^{2}}}+\frac{{{a}^{2}}}{2}{{\sin }^{-1}}\left( \frac{x}{a} \right)+c\]
8. \[\int{\sqrt{{{x}^{2}}-{{a}^{2}}}.dx}=\frac{x}{2}.\sqrt{{{x}^{2}}-{{a}^{2}}}-\frac{{{a}^{2}}}{2}.\log \left| \begin{matrix}

   \left. (x+\sqrt{{{x}^{2}}-{{a}^{2}}}) \right|  \\

\end{matrix} \right.+c\]

9. \[\int{\sqrt{{{x}^{2}}+{{a}^{2}}}.dx}=\frac{x}{2}.\sqrt{{{x}^{2}}+{{a}^{2}}}+\frac{{{a}^{2}}}{2}.\log \left| \begin{matrix}

   \left. (x+\sqrt{{{x}^{2}}+{{a}^{2}}}) \right|  \\

\end{matrix} \right.+c\]

 

• Tips to find the integration of the form of

\[\int{\frac{dx}{a+b\cos x}}\]or \[\int{\frac{dx}{a+b\sin x}}\]or \[\int{\frac{dx}{a\cos x+b\sin x+c}}\]

Step 1. Put\[\cos x=\frac{1-{{\tan }^{2}}\frac{x}{2}}{1+{{\tan }^{2}}\frac{x}{2}}\]and \[\sin x=\frac{2\tan \frac{x}{2}}{1+{{\tan }^{2}}\frac{x}{2}}\]

Step 2. Then put\[\tan \frac{x}{2}=t\]\[\Rightarrow dt=\frac{1}{2}{{\sec }^{2}}\frac{x}{2}.dx.\]After finding the integral in the form of t, convert t into x, putting \[t=\tan \frac{x}{2}.\]Other technique can be adopted as:

1. \[\frac{p(x)+q}{(x-\alpha )(x-\beta )}=\frac{A}{x-\alpha }+\frac{B}{x-\beta }\]
2. \[\frac{p(x)+q}{{{(x-\alpha )}^{2}}}=\frac{A}{(x-\alpha )}+\frac{B}{{{(x-\alpha )}^{2}}}\]
3. \[\frac{p{{x}^{2}}+qx+r}{(x-\alpha )(x-\beta )(x-\gamma )}=\frac{A}{(x-\alpha )}+\frac{B}{(x-\beta )}+\frac{C}{(x-\gamma )}\]
4. \[\frac{p{{x}^{2}}+qx+r}{{{(x-\alpha )}^{2}}(x-\beta )}=\frac{A}{(x-\alpha )}+\frac{B}{{{(x-\alpha )}^{2}}}+\frac{C}{(x-\beta )}\]
5. \[\frac{P{{x}^{2}}+qx+r}{(x-\alpha )({{x}^{2}}+bx+c)}=\frac{A}{(x-\alpha )}+\frac{Bx+C}{{{x}^{2}}+bx+c}\]

where \[{{x}^{2}}+bx+c\] cannot be factorised further.

 

• Integral of some special type of functions:

1. \[\int{\frac{dx}{{{x}^{2}}+{{a}^{2}}}}=\frac{1}{a}{{\tan }^{-1}}\left( \frac{x}{a} \right)+c\]
2. \[\int{\frac{dx}{{{x}^{2}}-{{a}^{2}}}}=\frac{1}{2a}.\log \left| \left. \frac{x-a}{x+a} \right|+c \right.\]
3. \[\int{\frac{dx}{{{a}^{2}}-{{x}^{2}}}}=\frac{1}{2a}.\log \left| \left. \frac{x+a}{x-a} \right|+c \right.\]
4. \[\int{\frac{dx}{\sqrt{{{a}^{2}}-{{x}^{2}}}}}={{\sin }^{-1}}\left( \frac{x}{a} \right)+c\]

 

• Tips to find the integration type of functions:

\[\int{\frac{dx}{a+b{{\cos }^{2}}x}}\]or \[\int{\frac{dx}{a+b{{\sin }^{2}}x}}\]or \[\int{\frac{dx}{a{{\cos }^{2}}x+b{{\sin }^{2}}x+c.\sin x.\cos x}}\]

Step 1. Divide the numerator & denominator by \[{{\cos }^{2}}x.\]

Step 2. Replace \[{{\sec }^{2}}x\] by \[1+{{\tan }^{2}}x\] in denominator.

Step 3. Then put\[\tan x=t\]to get \[dt={{\sec }^{2}}x.dx.\]

Then integrate the function in form oft. After finding out the integral in t, convert t into x by putting \[t=\tan x.\]

 

Solved Example

1. Find the value of the \[\int{\frac{dx}{1-{{\cos }^{4}}x}.}\]

            Sol. Let \[I=\int{\frac{dx}{1-{{\cos }^{4}}x}}\]

Dividing numerator and denominator by\[{{\cos }^{2}}x,\]we have

\[\int{\frac{\frac{1}{{{\cos }^{2}}x}}{\frac{1}{{{\cos }^{2}}x}-\frac{{{\cos }^{4}}x}{{{\cos }^{2}}x}}.dx=\int{\frac{{{\sec }^{2}}x}{{{\sec }^{2}}x-co{{x}^{2}}x}.dx=\int{\frac{{{\sec }^{2}}x}{{{\sec }^{2}}x-\frac{1}{{{\sec }^{2}}x}}.dx}}}\]

\[=\int{\frac{{{\sec }^{2}}x}{(1+{{\tan }^{2}}x)-\frac{1}{(1+{{\tan }^{2}}x)}}.dx}\]

Putting\[\tan x=t\Rightarrow dt={{\sec }^{2}}x.dx\]

Now, \[I=\int{\frac{dt}{(1+{{t}^{2}})-\frac{1}{(1+{{t}^{2}})}}=\int{\frac{(1+{{t}^{2}})dt}{{{t}^{2}}({{t}^{2}}+2)}=\int{\frac{{{t}^{2}}}{{{t}^{2}}({{t}^{2}}+2)}dt+\int{\frac{1}{{{t}^{2}}({{t}^{2}}+2)}dt}}}}\]

\[=\int{\frac{1}{{{t}^{2}}+2)}dt+\int{\frac{1}{{{t}^{2}}({{t}^{2}}+2)}dt}}\]

Let \[(I)={{I}_{1}}+{{I}_{2}}\](say)

Now, \[{{I}_{1}}=\int{\frac{I}{({{t}^{2}}+2)}}dt=\frac{1}{\sqrt{2}}{{\tan }^{-1}}\left( \frac{t}{\sqrt{2}} \right)\] and \[{{I}_{2}}=\int{\frac{1}{{{t}^{2}}({{t}^{2}}+2)}dt}\]

To solve the \[{{I}_{2}},\] let \[\frac{1}{{{t}^{2}}({{t}^{2}}+2)}=\frac{A}{{{t}^{2}}}+\frac{B}{{{t}^{2}}+2}\]

\[\frac{1}{{{t}^{2}}(t+2)}=\frac{A{{t}^{2}}+2A+B{{t}^{2}}}{{{t}^{2}}({{t}^{2}}+2)}\]

On comparing the coefficients, we get

\[A+B=0\]and\[2A=1\]

\[\Rightarrow A=\frac{1}{2},\] \[B=\frac{-1}{2}\]

So \[{{I}_{2}}=\int{\left[ \frac{1}{2{{t}^{2}}}-\frac{1}{2({{t}^{2}}+2} \right]}dt=\int{\frac{1}{2{{t}^{2}}}dt-\frac{1}{2}\int{\frac{1}{{{t}^{2}}+2}dt}}\]

\[=\left( \frac{1}{2} \right)\frac{{{t}^{-1}}}{(-1)}-\frac{1}{2}\left[ \frac{t}{\sqrt{2}}{{\tan }^{-1}}\left( \frac{t}{\sqrt{2}} \right) \right]+C=\frac{-1}{2t}-\frac{1}{2\sqrt{2}}{{\tan }^{-1}}\frac{t}{\sqrt{2}}+C\]

So, \[I={{I}_{1}}+{{I}_{2}}=\frac{1}{\sqrt{2}}{{\tan }^{-1}}\left( \frac{t}{\sqrt{2}} \right)+\frac{-1}{2t}-\frac{1}{2\sqrt{2}}{{\tan }^{-1}}\frac{t}{\sqrt{2}}+C\]

\[=\frac{1}{2\sqrt{2}}{{\tan }^{-1}}\left( \frac{t}{\sqrt{2}} \right)-\frac{1}{2t}+C=\frac{1}{2\sqrt{2}}{{\tan }^{-1}}\left( \frac{\tan x}{\sqrt{2}} \right)\frac{1}{2\tan x}+C\]

 

• Tip to solve the Integral of the form\[\int{{{\sin }^{m}}x{{\sin }^{n}}x\,dx}\]

To choose\[t=\sin x\]or\[\cos x\]. You are independent, but you will have to adopt one of that tool either \[t=\sin x\]or\[t=\cos x\]. By substituting the function in the other variable. Otherwise use the reduction formulae.

        

• Tips to solve the Integral of the form \[\int{\frac{\alpha x+\beta }{a{{x}^{2}}+bx+c}.dx},\int{\frac{\alpha x+\beta }{\sqrt{a{{x}^{2}}+bx+c}}.dx}\]

or \[\int{(\alpha x+\beta ).\sqrt{a{{x}^{2}}+bx+c}}.dx\]

When \[f(x)=\frac{\alpha x+\beta }{a{{x}^{2}}+bx+c}\]

Putting \[\alpha x+\beta =\lambda \](differentiation of denominator)\[+m=\lambda (2ax+b)+m\]

Then comparing the coefficient of x & const. term on both sides, we have find the integral after substituting the constant term \[\lambda \]& m.

 

• Tips to solve the integral of the form\[\int{\frac{{{x}^{2}}+1}{{{x}^{4}}+\alpha {{x}^{2}}+1}.dx}\], where \[\alpha \] is constant term.

Step 1. Dividing numerator & denominator by \[{{x}^{2}}\]

Step 2. Then put\[x+\frac{1}{x}=t\]or\[x-\frac{1}{x}=t\]

 

Whichever substitution, on differentiation gives the numerator of resulting integrand.

Step 3. Evaluate the resulting integral in terms of z and then express the result in the terms of x.

Solved Example

1. Find the value of the\[\int{\frac{{{x}^{2}}+1}{{{x}^{4}}+1}dx}\].

            Sol. Let\[I=\int{\frac{{{x}^{2}}+1}{{{x}^{4}}+1}.dx}\]

On dividing numerator & denominator by\[{{x}^{2}}\], we get

\[\therefore \,\,\,\,\,\,I=\int{\frac{{{x}^{2}}+\frac{1}{{{x}^{2}}}}{\frac{{{x}^{4}}+1}{{{x}^{2}}}}}.dx\]

\[\therefore \,\,\,\,\,\,I=\int{\frac{1+\frac{1}{{{x}^{2}}}}{{{x}^{2}}+\frac{1}{{{x}^{2}}}}}.dx=\int{\frac{1+\frac{1}{{{x}^{2}}}}{({{x}^{2}})+{{\left( \frac{1}{x} \right)}^{2}}}.dx=\int{\frac{1+\frac{1}{{{x}^{2}}}}{{{\left( x-\frac{1}{x} \right)}^{2}}+2}.dx}}\]

Now put \[x-\frac{1}{x}=t\]\[\Rightarrow dt=\left( 1+\frac{1}{{{x}^{2}}} \right).dx\]

\[\therefore \,\,\,\,\,\,I=\int{\frac{dt}{{{t}^{2}}+2}=\int{\frac{dt}{{{t}^{2}}+{{(\sqrt{2})}^{2}}}}}\]\[=\frac{1}{\sqrt{2}}.{{\tan }^{-1}}\left( \frac{t}{\sqrt{2}} \right)+c\]

\[=\frac{1}{\sqrt{2}}.{{\tan }^{-1}}\frac{\left( x-\frac{1}{x} \right)}{\sqrt{2}}+c=\frac{1}{\sqrt{2}}{{\tan }^{-1}}\left( \frac{{{x}^{2}}-1}{\sqrt{2}x} \right)+c\]

 

• Definite Integration: Definite integral has unique value. It is denoted by \[\int_{a}^{b}{f(x).dx,}\] where a is called the lower limit of the integral and b is called the upper limit of the integral. It is defined a s

\[\int_{a}^{b}{f(x).dx=[F(x)]_{a}^{b}}=F(b)-F(a)\]

 

• Tips: To find the definite integral of the function first of all, you have to find the indefinite integral as you know about the method of finding out indefinite integral. Further hence, putting the upper limit in the result minus the lower limit in the result.

e.g. \[I=\int_{0}^{4}{(x+{{e}^{2x}}).dx}\]

\[\therefore \,\,\,\,\,\,I=\int_{0}^{4}{(x+{{e}^{2x}}).dx}=\frac{{{x}^{2}}}{2}+\frac{{{e}^{2x}}}{2}\]

\[\therefore \,\,\,\,\,\,I=\int\limits_{0}^{4}{(x+{{e}^{2x}}).dx}=\left( \frac{{{x}^{2}}}{2}-\frac{{{e}^{2x}}}{2} \right)_{0}^{4}=\frac{({{4}^{2}}-{{0}^{2}})-({{e}^{2\times 4}}-{{e}^{2\times 0}})}{2}\]

\[=\frac{4\times 4-{{e}^{8}}+1}{2}=\frac{17-{{e}^{8}}}{2}\]

 

 

• Some properties of definite integrals

1. \[\int\limits_{a}^{b}{f(x).dx=-}\int\limits_{b}^{a}{f(x)dx}\]
2. \[\int\limits_{a}^{b}{f(x).dx=-}\int\limits_{a}^{b}{f(t)dt}\]
3. \[\int\limits_{a}^{b}{f(x).dx}=\int\limits_{a}^{c}{f(x).dx+}\int\limits_{c}^{b}{f(x).dx,}\] where \[c\in (a,b)\]
4. \[\int\limits_{0}^{a}{f(x).dx=\int\limits_{0}^{a}{f(a-x).dx}}\]
5. (i) \[\int\limits_{0}^{a}{f(x).dx=2\int\limits_{0}^{a}{f(x).dx,}}\]when\[f(x)\]is even function i.e.\[f(x)=f(-x).\]

(ii) \[\int\limits_{-a}^{a}{f(x)dx=0,}\]when\[f(x)\]is odd function i.e.\[f(-x)=-f(x)\]

6. (i) \[\int\limits_{0}^{2a}{f(x).dx=}2\int\limits_{0}^{a}{f(x).dx;}\] If \[f(2a-x)=f(x)\]

(ii) \[\int\limits_{0}^{2a}{f(x)dx=}0\]; If \[f(2a-x)=-f(x)\]

7. \[\int\limits_{a}^{b}{f(x)dx=\int\limits_{a}^{b}{f(a+b-x).dx}}\]
8. \[\int\limits_{0}^{2a}{f(x).dx=\int\limits_{0}^{a}{f(x).dx+}}\int\limits_{0}^{a}{f(2a-x).dx}\]
9. \[\int\limits_{0}^{a}{\frac{f(x)}{f(x)+f(a-x)}.dx}=\frac{a}{2}\]

 

Solved Example

1. Find the value of\[\int{\sqrt{\frac{1-\sqrt{x}}{1+\sqrt{x}}}.dx.}\]

            Sol. On putting \[x={{\cos }^{2}}\theta ,\]\[dx=-2\cos \theta .\sin \theta \,d\,\theta .\]

            Now\[I=\int{\sqrt{\frac{1-\cos \theta }{1+\cos \theta }}}(-2\sin \theta .\cos \theta ).d\theta \]

            \[=\int{\tan \frac{\theta }{2}(-2).\cos \theta .2\sin \frac{\theta }{2}.cos\frac{\theta }{2}.d\theta }\]\[=-4\int{\frac{\sin \frac{\theta }{2}}{\cos \frac{\theta }{2}}.\cos \theta .\sin \frac{\theta }{2}.\cos \frac{\theta }{2}.d\theta }\]

            \[=4\int{\cos }\theta .{{\sin }^{2}}\frac{\theta }{2}.d\theta =4\int{\left( 1-2{{\sin }^{2}}\frac{\theta }{2} \right)}.{{\sin }^{2}}\frac{\theta }{2}.d\theta \]

            \[=4\int{\left( {{\sin }^{2}}\frac{\theta }{2}-2{{\sin }^{4}}\frac{\theta }{2} \right).d\theta =4\left[ \int{{{\sin }^{2}}\frac{\theta }{2}.d\theta -8}\int{{{\sin }^{4}}\frac{\theta }{2}.d\theta } \right]}\]

            \[=4\int{\left( \frac{1-\cos \theta }{2} \right)}d\theta -8\int{{{\left( \frac{1-\cos \theta }{2} \right)}^{2}}d\theta }\]

            \[=4\left( \frac{1}{2}\theta -\frac{\sin \theta }{2} \right)-8\left[ \int{\frac{1+{{\cos }^{2}}\theta -2\cos \theta }{4}}d\theta  \right]\]

            \[=2\left( \theta -\sin \theta  \right)-8\left[ \int{\left( \frac{1}{4}+\frac{1+\cos 2\theta }{8}-\frac{\cos \theta }{2} \right).}d\theta  \right]\]

            \[=2\theta -2\sin \theta -2\theta -\theta -\frac{\sin 2\theta }{2}+4\sin \theta =-\theta +2\sin \theta -\sin \theta \cos \theta \]

            \[=-{{\cos }^{-1}}\sqrt{x}+2\sqrt{1-x}-\sqrt{x}\sqrt{1-x}+c\]    

           

• Reduction Formulae

1. \[\int{{{\sin }^{n}}x.dx}=-\frac{{{\sin }^{n-1}}x.\cos x}{n}+\left( \frac{n-1}{n} \right).{{\text{I}}_{n-2}}\]
2. \[\int{{{\cos }^{n}}x.dx=\frac{{{\cos }^{n-1}}x.\sin x}{n}+\left( \frac{n-1}{n} \right).{{\text{I}}_{n-2}}}\]
3. \[\int\limits_{0}^{\frac{\pi }{2}}{{{\sin }^{n}}x.dx}=\int\limits_{0}^{\frac{\pi }{2}}{{{\cos }^{n}}x.dx}=\left( \frac{n-1}{n} \right).{{\text{I}}_{n-2}}\]

\[\therefore {{\text{I}}_{n}}=\int\limits_{0}^{\frac{\pi }{2}}{{{\sin }^{n}}x.dx=\left\{ \frac{(n-1)(n-3)(n-5)....6.4.2}{n(n-2)(n-4)....7.5.3.1} \right\}}\]when n is odd

\[{{\text{I}}_{n}}=\int\limits_{0}^{\frac{\pi }{2}}{{{\cos }^{n}}x.dx=}\left\{ \frac{(n-1)(n-3)(n-5)....5.3.1}{n(n-2)(n-4)....6.4.2}\times \frac{\pi }{2} \right.\]

When n is even

4. \[\int{{{\tan }^{n}}x.dx=\frac{{{\tan }^{n-1}}x}{n-1}-{{\text{I}}_{n-2}}}\]

\[{{\text{I}}_{n}}=\int\limits_{0}^{\frac{\pi }{4}}{{{\tan }^{n}}x.dx}=\frac{1}{n-1}-{{\text{I}}_{n-2}}\,\,{{\text{I}}_{n}}\text{+}{{\text{I}}_{n-2}}=\frac{1}{n-1}\]

5. Reduction formulae for\[{{\sec }^{n}}x\,\,dx\]

\[{{\text{I}}_{n}}=\int{{{\sec }^{n}}xdx=\frac{\tan x.{{\sec }^{n-2}}x}{n-1}+\frac{n-2}{n-1}.}{{\text{I}}_{n-2}}\]

\[\int\limits_{0}^{\pi /4}{{{\sec }^{n}}x.dx}=\frac{{{(\sqrt{2})}^{n-2}}-1}{n-1}+\left( \frac{n-2}{n-1} \right).{{\text{I}}_{n-2}}\]

6. \[\int{{{\cot }^{n}}x.dx}=\frac{-{{\cot }^{n-1}}x}{n-1}-{{I}_{n-2}}\]
7. \[\int{\cos e{{c}^{n}}x.dx=\frac{-\cot x.\cos e{{c}^{n-2}}x}{n-1}+\left( \frac{n-2}{n-1} \right).{{\text{I}}_{n-2}}}\]
8. \[\int{{{x}^{n}}.{{e}^{ax}}.dx\frac{{{x}^{n}}.{{e}^{ax}}}{a}-\frac{n}{a}\int{{{x}^{n-1}}.{{e}^{ax}}.dx}}\]

\[{{\text{I}}_{n}}=\frac{{{x}^{n}}{{e}^{ax}}}{a}-\frac{n}{a}.{{I}_{n-1}}\]

9. (i) \[\int{{{\sin }^{m}}x{{\cos }^{n}}x.dx}\]

\[=\frac{{{\sin }^{m+1}}x.{{\cos }^{n-1}}x}{m+n}+\frac{n-1}{m+n}.\int{{{\sin }^{m}}x.{{\cos }^{n-2}}x\,dx}\]

(ii) \[\int{{{\sin }^{m}}x.co{{s}^{n}}xdx}=-\frac{{{\sin }^{m-1}}x{{\cos }^{n+1}}x}{m+n}+\frac{m-1}{m+n}\int{{{\sin }^{m-2}}x.co{{s}^{n}}x\,dx}\]

10. (i) \[\int\limits_{0}^{\pi /2}{{{\sin }^{m}}x.{{\cos }^{n}}xdx}=\frac{n-1}{m+n}\,\,\int\limits_{0}^{\pi /2}{si{{m}^{m}}x{{\cos }^{n-2}}xdx}\]

(ii) \[\int\limits_{0}^{\pi /2}{{{\sin }^{m}}x.{{\cos }^{n}}xdx}=\frac{m-1}{m+n}\,\,\int\limits_{0}^{\pi /2}{si{{m}^{m-2}}x{{\cos }^{n}}xdx}\]

11. \[\int\limits_{a}^{b}{\frac{f(x)}{f(x)+f(b+a-x)}.dx=\frac{b-a}{2}}\]
12. if \[f(x)\ge 0\]on the interval\[[a,b],\]\[\int\limits_{a}^{b}{f(x).dx\ge 0}\]
13. \[\left| \left. \int\limits_{a}^{b}{f(x).dx} \right| \right.\le \int\limits_{a}^{b}{\left| \left. f(x) \right| \right.}.dx\]
14. If \[f(x)\le g(x)\]on the interval\[[a,b],\]\[\int\limits_{a}^{b}{f(x).dx\le }\int\limits_{a}^{b}{g(x).dx}\]
15. If \[f(x)\]is continuous on\[[a,b]\]and m is the least & M is the greatest value of\[f(x)\]on\[[a,b],\]then\[m(b-a)\le \int\limits_{a}^{b}{f(x).dx\le M(b-a)}\]
16. If\[f(x)\]is periodic function of the period a i.e. \[f(a+x)=f(x),\]then

(a) \[\int\limits_{a}^{ma}{f(x).dx}=m\int\limits_{0}^{a}{f(x).dx}\]

(b) \[\int\limits_{a}^{m.a}{f(x).dx}=(m-1).\int\limits_{0}^{a}{f(x).dx}\]

(c) \[\int\limits_{ma}^{m(a+b)}{f(x).dx}=\int\limits_{a}^{b}{f(x).dx}\](cancelled same term in the limit)

(d) \[\int\limits_{b}^{na+b}{f(x).dx}=0\](result independent of b)

 

• Tips for Integration of Modulus function

\[I=\int\limits_{a}^{b}{\left| \left. f(x) \right| \right.}.dx\]

First of all, solve the equation\[f(x)=0\]

Suppose value of\[x=\alpha \And \beta \]etc.

Then, partition the given limits such that i.e. \[(a<\alpha <\beta <b).\]

\[(a,\alpha ),(\alpha ,\beta ),(\beta ,b)\]etc.

Check the sign of \[f(x)\] by taking any point in each of these intervals

Lastly use the properties of integral.

\[\int\limits_{a}^{b}{f(x).dx=\int\limits_{a}^{\alpha }{f(x).dx}+\int\limits_{\alpha }^{\beta }{f(x).dx}+\int\limits_{\beta }^{b}{f(x).dx.}}\] e.g. \[I=\int\limits_{2}^{8}{\left| \left. x-5 \right| \right..dx}\]

\[\because \,\,\,\,\,f(x)=x-5=0\Rightarrow x=5\]

\[\because \,\,\,\,\,\int\limits_{2}^{5}{f(x).dx+\int\limits_{5}^{8}{f(x).dx=\int\limits_{2}^{5}{-(x-5)dx+\int\limits_{5}^{8}{(x+5).dx}}}}\]

\[=\left( -\frac{{{x}^{2}}}{2}+5x \right)_{2}^{5}+\left( \frac{{{x}^{2}}}{2}+5x \right)_{5}^{8}\]

\[=\left( \frac{-25}{2}+25 \right)-\left( \frac{-4}{2}+10 \right)+\left( \frac{64}{2}+40 \right)-\left( \frac{25}{2}+25 \right)\]\[=\frac{25}{2}-8+72-\frac{75}{2}\]

\[=\frac{-50}{2}+64=-25+64=39\]

 

• Application of Integrals

Area of Plane Region:

1. Area bounded by the curve \[y=f(x),\] x-axis and the ordinate \[x=a\] & \[x=b\] (where\[b>a\]) is given by

\[A=\int\limits_{a}^{b}{y\,dx}=\int\limits_{a}^{b}{f(x)dx}\]

 

2. The area bounded by the curve \[x=g(x),\]y-axis\[y=c\]&\[y=d(d>c)\]is given by

\[A=\int\limits_{c}^{d}{xdy}=\int\limits_{c}^{d}{g(y)dy}\]

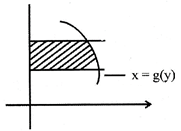
3. The area bounded by the curve\[y=h(x)\](which intersects the x-axis as shown below), x-axis & the two ordinate \[x=a\]and \[x=b\]is written as

 

 

 

If the two curves\[y=f(x)\]&\[y=g(x).\]Such that \[y=f(x)\]lies above the curve\[y=g(x)\]& both are the above the x-axis then bounded by them and the ordinate\[x=a\]& \[x=b\]\[(b>a)\]is written as

\[A=\int\limits_{a}^{b}{f(x).dx}-\int\limits_{a}^{b}{g(x).dx}\]

 

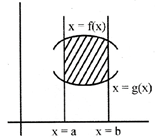
i.e. A=upper curve\[-\]lower curve

 

The area bounded by the curves \[y=f(x)\]& \[y=g(x)\](which intersect each other as shown in the figure) between the of ordinates\[x=a\] and\[x=b\]is written as

 

\[A=\int\limits_{a}^{c}{f(x).dx+\int\limits_{c}^{b}{g(x).dx}}\]where\[c=\]point of intersection of two curves.