Algebraic Expressions and Identities
- A combination of constants and variables connected by +, -, x and - is known as an algebraic expression.
e.g., \[2-3x+5{{x}^{-2}}{{y}^{-1}}+\frac{x}{3{{y}^{3}}}\]
An algebraic expression in which the variables involved have only non-negative integral powers is called a polynomial.
e.g., \[2-3x+5{{x}^{2}}{{y}^{-1}}-\frac{x}{3}x{{y}^{3}}\]
- Like terms: Terms formed from the same variables whose powers are same are called like
terms. The coefficients of like terms need not be the same.
- Unlike terms: Terms formed from different variables whose powers may be same or different are called unlike terms. The coefficients of unlike terms may or may not be the same.
In other words, terms with the same variables and which have the same exponent are called like or similar terms, otherwise they are called unlike (or) dissimilar terms.
e.g., (1)\[~3{{x}^{3}},\frac{1}{2}{{x}^{3}},- 9x3,.....\]
etc, are like terms.
(2) \[{{\operatorname{x}}^{2}}y,3x{{y}^{2}},-4{{x}^{3}}, .....\]
etc, are unlike terms.
- In case of a polynomial in one variable, the highest power of the variable is called the degree of the polynomial.
e.g.,
\[5{{x}^{3}}-7x+\frac{3}{2}\]
is a polynomial in\['x'\]of degree 3.
- In case of polynomial in more than one variable, the sum of the powers of the variables in each term is taken up and the highest sum so obtained is called the degree of the polynomial.
e.g.,
\[5{{x}^{3}}-2{{x}^{2}}{{y}^{2}}3{{x}^{2}}+9y\]
is a polynomial of degree 4 in \['x'\] and 'y'.
(i) Monomial: A polynomial containing 1 term is called a monomial.
(ii) Binomial: A polynomial containing 2 terms is called a binomial.
(iii) Trinomial: A polynomial containing 3 terms is called a trinomial.
- Multiplication of polynomials:
(i) A monomial multiplied by a monomial always gives a monomial.
(ii) While multiplying a polynomial by monomial, we multiply every term in the polynomial by the monomial.
(iii) In carrying out the multiplication of a polynomial by a binomial (or trinomial), we multiply term by term, i.e., every term of the polynomial is multiplied by every term in the binomial (or trinomial). Note that in such multiplication, we may get terms in the product which are like and have to be combined.
- An identity is an equality, which is true for all values of the variables in the equality.
- Some important identities:
(i) \[{{\left( a+b \right)}^{2}}={{a}^{2}}+2ab+{{b}^{2}}\]
(ii)\[{{\left( a-b \right)}^{2}}={{a}^{2}}-2ab+{{b}^{2}}\]
(iii) \[\left( a+b \right)\left( a-b \right)={{a}^{2}}-{{b}^{2}}\]
(iv) \[\left( x+a \right)\left( x+b \right)={{x}^{2}}+\left( a+b \right)x+ab\]
(v) These identities are useful in computing squares and products of algebraic expressions. They are alternative methods to calculate products of numbers too.
more...
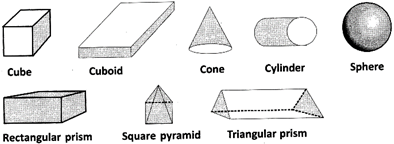
 Solid objects have three measurements – length, breadth and height or depth. So, they are called three – dimensional shapes. Also, Solids Occupy some Space.
e.g.,
Solid objects have three measurements – length, breadth and height or depth. So, they are called three – dimensional shapes. Also, Solids Occupy some Space.
e.g.,
 ·
·
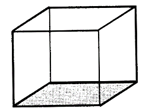 This polyhedron is regular. Its faces are congruent, regular polygons. Vertices are formed by the same number of faces.
This polyhedron is regular. Its faces are congruent, regular polygons. Vertices are formed by the same number of faces.
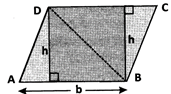 Then area of parallelogram = (base x height) sq. units
Then area of parallelogram = (base x height) sq. units