JEE Main & Advanced Chemistry GOC Geometrical Or Cis-Trans Isomerism

The compounds which have same molecular formula but differ in the relative spatial arrangement of atoms or groups in space are known as geometrical isomers and the phenomenon is known as geometrical isomerism. The isomer in which same groups or atoms are on the same side of the double bond is known as cis form and the isomer in which same groups or atoms are on the opposite side is called trans-isomer.            

Examples :

\[\underset{\text{Maleic acid }(cis)}{\mathop{\underset{H\,-\,\,C\,-\,COOH}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-COOH}}\,}}\,\]                           \[\underset{\text{Fumaric acid }(trans)}{\mathop{\underset{HOOC-C-H\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-COOH}}\,}}\,\]

\[\underset{\text{Citraconic acid (}cis\text{-isomer)}}{\mathop{\underset{\,\,\,\,\,\,H-C-COOH}{\mathop{{{H}_{3}}C-\underset{|\,|}{\mathop{C}}\,-COOH}}\,}}\,\]                              \[\underset{\text{Mesaconic}\,\text{acid}\,\text{(}trans-\text{isomer)}}{\mathop{\underset{HOOC-C-H}{\mathop{\,\,\,\,\,\,\,\,\,\,\,\,\,\,{{H}_{3}}C-\underset{|\,|}{\mathop{C}}\,-COOH}}\,}}\,\]

(1) Conditions for geometrical isomerism : Compound will show geometrical isomerism if it fulfils the following two conditions

(i) There should be frozen rotation about two adjacent atoms in the molecule.

(a) frozen rotation about carbon, carbon double bond in alkenes.              

(b) frozen rotation about carbon, carbon single bond in cycloalkanes.            

(c)     \[C=N-\] frozen rotation about carbon, nitrogen double bond in oxime and imine.            

(ii) Both substituents on each carbon should be different about which rotation is frozen.            

If these two conditions are fulfilled, then compound will show geometrical isomerism.       

• The compounds of the following type will not show geometrical isomerism.                                

\[\underset{\text{  }}{\mathop{\underset{x-C-y}{\mathop{a-\underset{||}{\mathop{C}}\,-a}}\,\,\,\,\underset{a-C-a}{\mathop{x-\underset{||}{\mathop{C}}\,-a}}\,\,\,\underset{x-C-x}{\mathop{a-\underset{||}{\mathop{C}}\,-a}}\,}}\,\]            

(2) Distinction between cis- and trans- isomers            

(i) By cyclization method : Generally, the cis-isomer (e.g. maleic acid) cyclises on heating to form the corresponding anhydride while the trans-isomer does not form its anhydride.        

• Note that the two reacting groups (–COOH) are near to each other.          

\[\underset{\text{Fumaric acid (trans)}}{\mathop{\underset{HOOC-C-H}{\mathop{\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,H-\underset{|\,|}{\mathop{C}}\,-COOH}}\,}}\,\begin{matrix}    {}  \\    \xrightarrow{\text{Heat}}\text{No anhydride}  \\ \end{matrix}\]   

• Note that the two reacting groups (–COOH)  are quite apart from each other, hence cyclisation is not possible.            

(ii) By hydroxylation (Oxidation by means of \[KMn{{O}_{4}},\,Os{{O}_{4}}\] or \[{{H}_{2}}{{O}_{2}}\] in presence of \[Os{{O}_{4}}\]) : Oxidation (Hydroxylation) of alkenes by means of these reagents proceeds in the cis-manner. Thus the two geometrical isomers of an alkene leads to different

products by these reagents. For example,

(iii) By studying their dipole moments : The cis-isomer of a symmetrical alkene (Alkenes in which both the carbon atoms have similar groups) has a definite dipole moment, while the trans-isomer has either zero dipole moment or less dipole moment than the cis-isomer. For example, 1,2-dichloroethylene and butene-2.

\[\underset{(\mu =1.9D)}{\mathop{\underset{cis-\,\text{Dichloroethylene}}{\mathop{\underset{H-C-Cl}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-Cl}}\,}}\,}}\,\]                              \[\underset{(\mu =0.0D)}{\mathop{\underset{trans-\,\text{Dichloroethylene}}{\mathop{\underset{Cl-C-H}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-Cl}}\,}}\,}}\,\]

\[\,\underset{cis-\,\text{Butene}-\text{2}}{\mathop{\underset{H-C-C{{H}_{3}}}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-C{{H}_{3}}}}\,}}\,\]                         \[\,\underset{(\mu =0.0D)}{\mathop{\underset{trans-\,\text{Butene}-\text{2}}{\mathop{\underset{C{{H}_{3}}-C-H}{\mathop{\,\,\,\,\,\,\,\,\,\,\,H-\underset{|\,|}{\mathop{C}}\,-C{{H}_{3}}}}\,}}\,}}\,\]

In trans-isomer of the symmetrical alkenes, the effect produced in one half of the molecule is cancelled by that in the other half of the molecule.

In case of unsymmetrical alkenes, the cis-isomer has higher dipole moment than the corresponding trans-isomer.

For Example,

\[\underset{\begin{smallmatrix}cis-2,3-\text{Dichloropentene}-2 \\\text{(High}\,\text{dipole}\,\text{moment)}\end{smallmatrix}}{\mathop{\underset{C{{H}_{3}}-C{{H}_{2}}-C-Cl\,\,\,\,\,\,\,\,\,\,\,\,\,\,}{\mathop{{{H}_{3}}C-\underset{||}{\mathop{C}}\,-Cl}}\,}}\,\]                                      \[\underset{\begin{smallmatrix}trans-2,3-Dichloropentene-2 \\(Less\,dipole\,moment)\end{smallmatrix}}{\mathop{\underset{\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,Cl-C-C{{H}_{2}}C{{H}_{3}}}{\mathop{C{{H}_{3}}-\underset{|\,|}{\mathop{C}}\,-Cl}}\,}}\,\]

Similar is the case with hexene-2.

(iv) By studying other physical properties: (a) The cis-isomer of a compound has higher boiling point due to higher polarity, higher density and higher refractive index than the corresponding trans-isomer (Auwers-skita rule).

\[\underset{\text{m}\text{.p}\text{.}\,\,\,\,\,\,\,\,\,\,\,\,\,\,-139{}^\circ C\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,}{\mathop{\underset{\text{b}\text{.p}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,4{}^\circ C\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,}{\mathop{\underset{cis-2-\text{Butene}}{\mathop{\underset{C{{H}_{3}}-\,\,C-H}{\mathop{C{{H}_{3}}-\underset{|\,|}{\mathop{C}}\,-H}}\,}}\,}}\,}}\,\]  \[\underset{-106{}^\circ C}{\mathop{\underset{1{}^\circ C}{\mathop{\underset{trans-2\text{Butene}}{\mathop{\underset{\,\,\,\,\,\,\,\,\,\,\,H-C-C{{H}_{3}}}{\mathop{C{{H}_{3}}-\underset{|\,|}{\mathop{C}}\,-H}}\,}}\,}}\,}}\,\]             \[\underset{-80{}^\circ C}{\mathop{\underset{60{}^\circ C}{\mathop{\underset{cis-1,2-Dichloroethene}{\mathop{\underset{H-C-Cl}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-Cl}}\,}}\,}}\,}}\,\]        \[\underset{-50{}^\circ C}{\mathop{\underset{48{}^\circ C}{\mathop{\underset{trans-1,2-Dichloroethene}{\mathop{\underset{Cl-C-H}{\mathop{H-\underset{|\,|}{\mathop{C}}\,-Cl}}\,}}\,}}\,}}\,\]           

(b) The trans-isomer has higher melting point than the cis-isomer due to symmetrical nature and more close packing of the trans-isomer.            

(v) Stability : Trans-isomer is more  stable than cis-isomer due to symmetrical structure.            

• Terminal alkenes such as propene, 1-butene and 2-methyl propene do not show geometrical isomerism.         
• Cis-trans isomers are configurational isomers but not mirror images, hence cis and trans isomers are always diastereomers.         
• Non-terminal alkenes with the same atoms or groups either on one or both the carbon atoms of the double bond such as 2-methyl-2-butene, 2,3-dimethyl -2- butene etc. do not show geometrical isomerism.            

(3) E and Z system of nomenclature : ‘Cis’ and ‘Trans’ designations cannot be used if four different atoms or groups are attached to the carbon atoms of a double bond.

In such cases, E and Z system of nomenclature is used. This system is based on a priority system developed by Cahn, Ingold and Prelog.

In this system, the two atoms or groups attached to each of the doubly bonded carbon are put in order of preference on the basis of sequence rules.

The symbol ‘E’ is assigned to an isomer in which the atoms or groups of higher preference are on the opposite side (E from German word Entgegen = across or opposite).

The symbol ‘Z’ is assigned to an isomer in which the atoms or groups of higher preference are on the same side (Z from German word, Zusammen = together).

• 1 signifies higher preference and 2 signifies lower preference. Preference in most of the cases ‘Z’ corresponds to cis-form and ‘E’ to trans-form. However, there are many exceptions.

The following rules are followed for deciding the precedence order of the atoms or groups;

(i) Higher priority is assigned to the atoms of higher atomic number. For example, the order of preference in the following atoms, \[H,Cl,I,Br\] is : I (at. no. 53)> Br (at. no. 35)>Cl (at. no. 17)>H (at. no. 1).

(ii) If isotopes of the same element are attached, the isotope with higher mass number is given higher order of preference. For example, deuterium \[\left( _{1}^{2}D \right)\] is assigned higher priority in comparison to hydrogen \[\left( _{1}^{1}H \right)\].

(iii) In the groups, the order of preference is also decided on the basis of atomic number of first atom of the group. For example, in the following set,

\[-Cl,-OH,-COOH,-NH-C{{H}_{3}},-S{{O}_{3}}H\].

The order of the precedence is :

\[\underset{\text{(}\,\,\,\,\text{at}\text{.no17)}}{\mathop{-\underset{\downarrow }{\mathop{Cl}}\,}}\,>\underset{\text{(at}\text{.no}\text{.16)}\,\,\,\,\,\,\,\,}{\mathop{-\underset{\downarrow }{\mathop{S}}\,{{O}_{3}}H}}\,>-\underset{\text{(at}\text{.no}\text{.8)}\,\,\,\,\,\,}{\mathop{\underset{\downarrow }{\mathop{O}}\,H}}\,>\underset{\text{(at}\text{.no}\text{.7)}\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,}{\mathop{-\underset{\downarrow }{\mathop{N}}\,HC{{H}_{3}}}}\,>\underset{\text{(at}\text{.no}\text{. 6)}\,\,\,\,\,\,\,\,\,\,\,\,}{\mathop{-\underset{\downarrow }{\mathop{C}}\,OOH}}\,\]

When the order of preference of the groups cannot be settled on the first atom, the second atom or the subsequent atoms in the groups are considered. For example, in the set \[-C{{H}_{2}}-C{{H}_{3}},-C{{H}_{3}},-COOH,\] the order cannot be decided on the basis of first atom as it is same in all the groups. However, in \[-C{{H}_{2}}-C{{H}_{3}},\] the second atom is carbon, in \[-C{{H}_{3}},\] the second atom is hydrogen while in \[-COOH,\] the second atom is oxygen. Hence, the order of preference is :

\[\underset{\text{(at}\text{.no}\text{.8)}}{\mathop{-C\underset{\downarrow }{\mathop{O}}\,OH}}\,>-C{{H}_{2}}\underset{\text{(at}\text{.no}\text{.6)}\,\,\,\,\,}{\mathop{-\underset{\downarrow }{\mathop{C}}\,{{H}_{3}}}}\,>\underset{\,\,\,\,\,\,\,\,\,\text{(at}\text{.no}\text{.1)}}{\mathop{-C\underset{\downarrow }{\mathop{{{H}_{3}}}}\,}}\,\]

(iv) A doubly or triply bonded atom is considered equivalent to two or three such atoms. For example,

The group is equal to  and the group \[-C\equiv N\] is equal to \[-\underset{N\,\,\,\,\,\,\,\,\,}{\overset{N\,\,\,\,\,\,\,\,}{\mathop{\underset{|}{\overset{|}{\mathop{C}}}\,-N}}}\,\].            

(4) Number of geometrical isomers in polyenes            

(i) When a compound has n double bonds and ends of a polyene are different, the number of geometrical isomers \[={{2}^{n}}\]

\[{{C}_{6}}{{H}_{5}}-CH=CH-CH=CH-CH=CH-CH=CH-Cl\]

The given compound has four double bonds and the two ends are different (One is \[{{C}_{6}}{{H}_{5}}\] and other is Cl). Therefore, number of geometrical isomers \[={{2}^{n}}={{2}^{4}}=16\].

(ii) When the ends of polyene are same.

Case I : When number of double bonds (=n) is even then the number of geometrical isomers \[={{2}^{n-1}}+{{2}^{[(n/2)-1]}}\]

\[\underset{n=4,\,\text{even}}{\mathop{Cl-CH=CH-CH=CH-CH=CH-CH=CH-Cl}}\,\]

Number of geometrical isomers \[={{2}^{n-1}}+{{2}^{(n/2)-1}}\] \[={{2}^{3}}+{{2}^{1}}\] \[=8+2=10\].

Case II : When number of double bonds (=n) is odd.

Number of geometrical isomers \[={{2}^{n-1}}+{{2}^{\left[ \frac{n+1}{2} \right]-1}}\]

\[{{C}_{6}}{{H}_{6}}-CH=CH-CH=\]\[\underset{(n=3,\,\text{ odd})}{\mathop{CH}}\,=CH-CH=CH-{{C}_{6}}{{H}_{5}}\]

Number of geometrical isomers \[={{2}^{2}}+{{2}^{2-1}}\] \[={{2}^{2}}+{{2}^{1}}\] \[=4+2=6\].

(5) Geometrical Isomerism in nitrogen compounds

(i) Geometrical isomerism due to  bond.            

The important class of compounds exhibiting geometrical isomerism due to   \[C=N-\]bond are oximes, nitrones, hydrazones and semicarbazones. But the most common compound is oxime.            

Oximes : In aldoxime, when hydrogen and hydroxyl groups are on the same side, the isomer is known as syn. (analogous to cis) and when these groups are on the opposite side, the isomer is known as anti (analogous to trans)

\[\underset{\text{Syn-benzaldoxime}}{\mathop{\underset{\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,N-OH}{\overset{{{C}_{6}}{{H}_{5}}-C-H}{\mathop{\,\,\,\,\,\,\,\,\,|\,|}}}\,}}\,\]        \[\underset{\text{Anti}-\text{benzaldoxime}}{\mathop{\underset{HO-N\,\,\,\,}{\overset{{{C}_{6}}{{H}_{5}}-C-H}{\mathop{\,\,\,\,\,\,\,\,\,\,\,\,\,|\,|}}}\,}}\,\]

In ketoximes the prefixes syn and anti indicate which group of ketoxime is syn or anti to hydroxyl group. For example:

\[\underset{\,\,\,\,\,\,\,\,\,\,N-OH}{\overset{C{{H}_{3}}-C-{{C}_{2}}{{H}_{5}}}{\mathop{||\,\,}}}\,\] this compound will be named as;

(a) Syn-ethyl methyl ketoxime \[\Rightarrow \,HO\] and \[{{C}_{2}}{{H}_{5}}\] are syn or

(b) Anti-methyl ethyl ketoxime \[\Rightarrow HO\] and \[{{C}_{2}}{{H}_{5}}\] are anti.

Similarly consider the following structure

\[\underset{\begin{smallmatrix}\text{Syn}-\text{methyl}\,\text{ethyl}\,\text{ketoxime } \\\text{or Anti}-\text{ethyl}\,\text{methyl}\,\text{ketoxime}\end{smallmatrix}}{\mathop{\underset{\,\,\,\,\,\,\,\,\,\,\,N-OH}{\overset{{{C}_{2}}{{H}_{5}}-C-C{{H}_{3}}}{\mathop{\,\,\,\,|\,|}}}\,}}\,\]

(ii) Geometrical isomerism due to N = N bond.

\[\underset{\text{Syn-azobenzene}}{\mathop{\underset{{{C}_{6}}{{H}_{5}}-N}{\mathop{{{C}_{6}}{{H}_{5}}-\underset{|\,|}{\mathop{N}}\,}}\,}}\,\]         \[\underset{\text{Anti}-\text{azobenzene}}{\mathop{\underset{\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,\,N-{{C}_{6}}{{H}_{5}}}{\mathop{{{C}_{6}}{{H}_{5}}-\underset{|\,|}{\mathop{N}}\,}}\,}}\,\]

(6) Geometrical isomerism show by cumulatrienes : Cumulatrienes (Trienes with three adjacent double bonds) show only geometric isomerism. This is because their molecule is planar, as such the terminal \[-C{{H}_{3}}\] groups and H- atoms lie in the same plane. Therefore, in this case their planar structure can exist in two diastereoisomeric forms, cis- and trans- but no enantiomeric forms are possible.

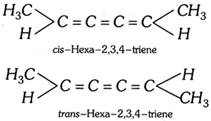
(7) Geometrical isomerism in cycloalkanes : Disubstituted cycloalkanes show geometrical isomerism.

• Certain compounds show geometrical as well optical isomerism. Such type of isomerism is known as geometrical enantiomerism.