-
question_answer1)
A horsed drinking through has the dimensions shown. How long will it take to fill the through if water flows it in 22 litters per minute?
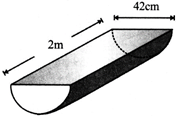
A)
6 m done
clear
B)
6 m 18 sec. done
clear
C)
6 m 42 sec done
clear
D)
6 m 22 sec done
clear
View Solution play_arrow
-
question_answer2)
An ice block with uniform cross section has the dimension shown alongside what is the volume of the ice block?
A)
36 \[c{{m}^{2}}\] done
clear
B)
40 \[c{{m}^{2}}\] done
clear
C)
44 \[c{{m}^{2}}\] done
clear
D)
50 \[c{{m}^{2}}\] done
clear
View Solution play_arrow
-
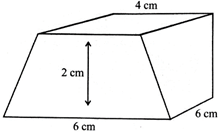
question_answer3)
What is the surface area of solid given along side?

A)
96 \[c{{m}^{2}}\] done
clear
B)
108 \[c{{m}^{2}}\] done
clear
C)
100 \[c{{m}^{2}}\] done
clear
D)
92 \[c{{m}^{2}}\] done
clear
View Solution play_arrow
-
question_answer4)
What is the volume of the solid shown alongside?
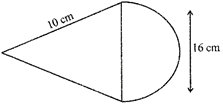
A)
265 \[c{{m}^{3}}\] done
clear
B)
255 \[c{{m}^{3}}\] done
clear
C)
359 \[c{{m}^{3}}\] done
clear
D)
569 \[c{{m}^{3}}\] done
clear
View Solution play_arrow
-
question_answer5)
What is the volume of the solid shown alongside?
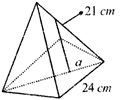
A)
4032 \[c{{m}^{3}}\] done
clear
B)
4023 \[c{{m}^{3}}\] done
clear
C)
3210 \[c{{m}^{3}}\] done
clear
D)
3040 \[c{{m}^{3}}\] done
clear
View Solution play_arrow
-
question_answer6)
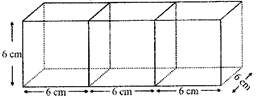
Three cubes each of side 6 cm are joined end-to-end as shown in the figure. Then what is the surface area of the resulting cuboid?
A)
252 \[c{{m}^{2}}\] done
clear
B)
504 \[c{{m}^{2}}\] done
clear
C)
540 \[c{{m}^{2}}\] done
clear
D)
450 \[c{{m}^{2}}\] done
clear
View Solution play_arrow
-
question_answer7)
10 cylindrical pillars of a building have to be painted. The radius of each pillar is 35 cm and the height is 2 m. what is the cost of painting at the rate of Rs 5 per sq m?
A)
Rs 200 done
clear
B)
Rs 220 done
clear
C)
Rs 280 done
clear
D)
Rs 500 done
clear
View Solution play_arrow
-
question_answer8)
A right triangle ABC with its sides 5 cm, 12 cm, and 13 cm is revolved about the side 12 cm. Find the volume of the solid so formed.
A)
\[59\text{ }\pi \,c{{m}^{2}}\] done
clear
B)
\[5\pi c{{m}^{2}}\] done
clear
C)
\[100\pi c{{m}^{2}}\] done
clear
D)
\[120\pi c{{m}^{2}}\] done
clear
View Solution play_arrow
-
question_answer9)
A cardboard sheet in the form of a circular sector of radius 20 cm and central angle\[\mathbf{10}{{\mathbf{8}}^{{}^\circ }}\] is folded to make a cone. What is the radius of the cone?
A)
6 cm done
clear
B)
18 cm done
clear
C)
21 cm done
clear
D)
4 cm done
clear
View Solution play_arrow
-
question_answer10)
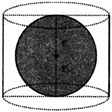
A right cylinder just encloses a sphere of radius r as shown in the given figure. What is the ratio of surface area of sphere and curved surface area of the cylinder?
A)
2 : 1 done
clear
B)
1 : 3 done
clear
C)
\[1:\sqrt{2}\] done
clear
D)
1 : 1 done
clear
View Solution play_arrow
-
question_answer11)
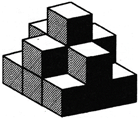
A child playing with building blocks which are of the shape of the cubes has build a structure as shown in the figure given below. If the each of the cube is 2 cm. what is the structure built by the cube?
A)
96 \[c{{m}^{3}}\] done
clear
B)
120 \[c{{m}^{3}}\] done
clear
C)
114 \[c{{m}^{3}}\] done
clear
D)
112 \[c{{m}^{3}}\] done
clear
View Solution play_arrow
-
question_answer12)
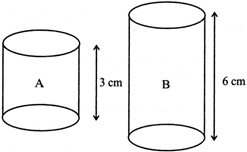
Cylinder A and B are with heights 2 cm and 4 cm respectively. If cylinder 4 has volume 20\[\mathbf{c}{{\mathbf{m}}^{\mathbf{3}}}\], then the volume of cylinder B is
A)
60\[c{{m}^{2}}\] done
clear
B)
70\[c{{m}^{3}}\] done
clear
C)
80\[c{{m}^{3}}\] done
clear
D)
160\[c{{m}^{3}}\] done
clear
View Solution play_arrow
-
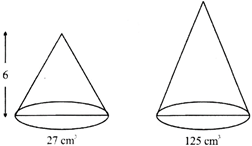
question_answer13)
A and B are similar cones with volumes 27\[\mathbf{c}{{\mathbf{m}}^{\mathbf{3}}}\]and 125\[\mathbf{c}{{\mathbf{m}}^{2}}\]respectively. If cone A is 6 cm high, then the height of cone B will be
A)
10 cm done
clear
B)
5 cm done
clear
C)
15 cm done
clear
D)
20 cm done
clear
View Solution play_arrow
-
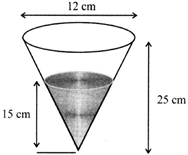
question_answer14)
A conical flask has height 25 cm. and base diameter 12 cm. Water is poured into the flask in a depth of 15 cm. The diameter of the surface of the water is
A)
6 cm done
clear
B)
7.2 cm done
clear
C)
8 cm done
clear
D)
9 cm done
clear
View Solution play_arrow
-
question_answer15)
What is the length of the uniform wire of radius 0.2 cm that can be drawn from a solid sphere of radius 3 cm?
A)
9 m done
clear
B)
24 m done
clear
C)
12 m done
clear
D)
60 m done
clear
View Solution play_arrow
-
question_answer16)
What will be the cost to plaster the inner surface of a well 28 m deep and 2 m in radius at the rate of 25 per sq m?
A)
Rs 4000 done
clear
B)
Rs 2200 done
clear
C)
Rs 8800 done
clear
D)
Rs 4400 done
clear
View Solution play_arrow
-
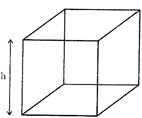
question_answer17)
In the given figure, volume of a square based box is 500\[\mathbf{c}{{\mathbf{m}}^{2}}\] and base length is 10 cm. The height h of the box is
A)
7 .5 cm done
clear
B)
10 cm done
clear
C)
5 cm done
clear
D)
2.5 cm done
clear
View Solution play_arrow
-
question_answer18)
The length of a cold storage is double its breadth. Its height is 3 metres. The area of its four walls (including doors) is 180 in."'. What is its volume?
A)
210 \[c{{m}^{3}}\] done
clear
B)
220 \[c{{m}^{3}}\] done
clear
C)
216\[c{{m}^{3}}\] done
clear
D)
250 \[c{{m}^{3}}\] done
clear
View Solution play_arrow
-
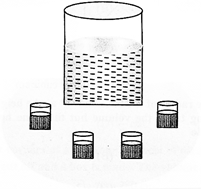
question_answer19)
At a Dusherra Mela, a stall keeper in one of the food stalls has a large cylindrical vessel of base radius 25 cm filled lap to height of 24 cm with orange juice. The juice is filled in small cylindrical glasses (See figure) of radius 5 cm up to a height of 6 cm, and sold for Rs. 10 each. How much money does the stall keeper receive by selling the juice completely?
A)
Rs 1000 done
clear
B)
Rs 800 done
clear
C)
Rs 1200 done
clear
D)
Rs 900 done
clear
View Solution play_arrow
-
question_answer20)
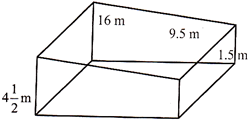
The Level of water of a swimming pool is rectangular of which length is 32 metres and width is 9.5 metres and the depth of water at one end is \[1\frac{1}{2}\]metres which Increases to \[4\frac{1}{2}\]metres at other end. Find the volume of water in the swimming pool.
A)
900 \[metr{{e}^{3}}\] done
clear
B)
456 \[metr{{e}^{3}}\] done
clear
C)
942 \[metr{{e}^{3}}\] done
clear
D)
924 \[metr{{e}^{3}}\] done
clear
View Solution play_arrow
-
question_answer21)
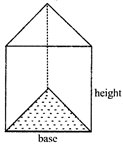
The base of a right prism is an equilateral triangle of side 6 cm and height of the prism is 20 cm. then the volume of the prism is
A)
\[320\sqrt{3}\]cubic cm done
clear
B)
\[180\sqrt{3}\]cubic cm done
clear
C)
\[150\sqrt{3}\]cubic cm done
clear
D)
\[300\sqrt{3}\]cubic cm done
clear
View Solution play_arrow
-
question_answer22)
A cylinder has 'r' as the radius of the base and 'V as the height. The radius of base of another cylinder, having triple the volume but the same height as that of the first cylinder must be equal to
A)
\[\frac{r}{\sqrt{2}}\] done
clear
B)
2r done
clear
C)
\[r\sqrt{3}\] done
clear
D)
\[\sqrt{2r}\] done
clear
View Solution play_arrow
-
question_answer23)
The height of the cone is 40 cm. A small cone is cut off at the top by a plane parallel to its base. If its volume is\[\frac{1}{64}\]of the volume of the cone. At what height, above the base, is the section made?
A)
6 cm done
clear
B)
8 cm done
clear
C)
10 cm done
clear
D)
20 cm done
clear
View Solution play_arrow
-
question_answer24)
If the ratio of the diameters of two spheres is 4:5, then what is the ratio of their surface areas?
A)
16 : 25 done
clear
B)
9 : 10 done
clear
C)
3 : 5 done
clear
D)
16 : 25 done
clear
View Solution play_arrow
-
question_answer25)
The radius of base of a right circular cone is 3.5 cm and slant height is 10 cm, then what is its lateral surface area?
A)
110 sq cm done
clear
B)
100 sq cm done
clear
C)
70 sq cm done
clear
D)
49 sq cm done
clear
View Solution play_arrow
-
question_answer26)
The base of a right pyramid is a square of side 30 cm long. If the volume of the pyramid is 4500 cm3 then its height is
A)
5 cm done
clear
B)
10cm done
clear
C)
15 cm done
clear
D)
20 cm done
clear
View Solution play_arrow
-
question_answer27)
The respective height and volume of a cylinder and a right circular Hemisphere are equal, then the ratio of their radii is
A)
\[\sqrt{2}:\sqrt{3}\] done
clear
B)
\[\sqrt{3}:1\] done
clear
C)
\[\sqrt{3}:\sqrt{2}\] done
clear
D)
\[2:\sqrt{3}\] done
clear
View Solution play_arrow
-
question_answer28)
The radii of the base of Cone and a Cylinder are in the ratio \[\sqrt{3}:\sqrt{2}\] are in the ratio \[\sqrt{2}:\sqrt{3}\]. Their volume are in the ratio of
A)
\[\sqrt{3}:\sqrt{2}\] done
clear
B)
\[3\sqrt{3}:\sqrt{2}\] done
clear
C)
\[\sqrt{3}:2\sqrt{2}\] done
clear
D)
\[\sqrt{2}:\sqrt{6}\] done
clear
View Solution play_arrow
-
question_answer29)
A rectangular water tank is\[\mathbf{160m}\times \mathbf{20m}\]. Water flows at the opening at a speed of 10 km/hr. The water level will rise in the tank in half an hour by
A)
\[\frac{3}{2}\]cm. done
clear
B)
\[\frac{4}{9}\]cm, done
clear
C)
\[\frac{5}{9}\]cm. done
clear
D)
\[\frac{5}{8}\]cm. done
clear
View Solution play_arrow
-
question_answer30)
From each of the four corners of a rectangular sheet of diamensions\[\mathbf{20cm}\times \mathbf{16cm}\], a square of side 3 cm is cut off and a box is made. The volume of the box is
A)
828 \[c{{m}^{3}}\] done
clear
B)
280 \[c{{m}^{3}}\] done
clear
C)
500 \[c{{m}^{3}}\] done
clear
D)
1000 \[c{{m}^{3}}\] done
clear
View Solution play_arrow


