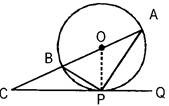
A) \[\text{a}{}^\circ +\text{b}{}^\circ =\text{18}0{}^\circ \]
B) \[\text{a}{}^\circ +\text{2b}{}^\circ =\text{9}0{}^\circ \]
C) \[\text{a}{}^\circ -\text{b}{}^\circ =\text{6}0{}^\circ \]
D) \[\text{2a}{}^\circ +\text{b}{}^\circ =\text{1}00{}^\circ \]
Correct Answer: B
Solution :
Given \[x=\frac{5040\times 12}{144}=420\]and \[219\times =657\]. Also, \[3\times =219\] (Angles in an isosceles triangle OAP, angle in deternate segment.) \[\text{1}0\text{32 }=\text{ 4}0\text{8 }\times \text{ 2 }+\text{ 216}\] \[\text{4}0\text{8 }=\text{ 216 }\times \text{ 1 }+\text{ 192}\] \[\text{216 }=\text{ 192 }\times \text{ 1 }+\text{ 24}\] In \[\text{192 }=\text{ 24 }\times \text{ 8 }+\text{ }0\] \[{{6}^{x}}\]\[{{6}^{x}}={{(2\times 3)}^{x}}={{2}^{x}}\times {{3}^{x}}\]You need to login to perform this action.
You will be redirected in
3 sec
