A) \[\sqrt{\frac{20}{3}}\]
B) \[\frac{2}{\sqrt{15}}\]
C) \[\sqrt{\frac{8}{15}}\]
D) \[\sqrt{\frac{15}{2}}\]
Correct Answer: A
Solution :
\[AD=\left| \,\frac{-2-2-1}{\sqrt{{{(2)}^{2}}+{{(-1)}^{2}}}}\, \right|=\left| \,\frac{-5}{\sqrt{5}}\, \right|=\sqrt{5}\] \[\because \] \[\tan {{60}^{o}}\] \[=\frac{AD}{BD}\Rightarrow \sqrt{3}=\frac{\sqrt{5}}{BD}\]Þ \[BD=\sqrt{\frac{5}{3}}\]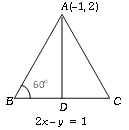 \[\therefore \] \[BC=2BD=2\sqrt{\frac{5}{3}}=\sqrt{\frac{20}{3}}\].
\[\therefore \] \[BC=2BD=2\sqrt{\frac{5}{3}}=\sqrt{\frac{20}{3}}\].
You need to login to perform this action.
You will be redirected in
3 sec
