A) \[\frac{{{u}^{2}}}{g}{{\cos }^{2}}\alpha \]
B) \[\frac{{{u}^{2}}}{g}\cos 2\alpha \]
C) \[\frac{2{{u}^{2}}}{g}{{\cos }^{2}}2\alpha \]
D) \[\frac{2{{u}^{2}}}{g}{{\cos }^{2}}\alpha \]
Correct Answer: D
Solution :
According to the figure, the length of latus rectum is \[2(SM)=2\times \frac{{{u}^{2}}}{2g}(1+\cos 2\alpha )=\frac{2{{u}^{2}}{{\cos }^{2}}\alpha }{g}\].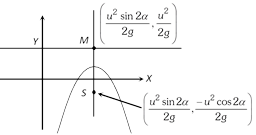
You need to login to perform this action.
You will be redirected in
3 sec
