question_answer

The area bounded by the curve \[y=x{{(3-x)}^{2}},\] the x-axis and the ordinates of the maximum and minimum points of the curve, is given by

A) 1 sq. unit

B) 2 sq. unit

C) 4 sq. unit          

D) none of these

Correct Answer: C

Solution :

clearly, the curve \[y=x{{(3-x)}^{2}}\] has maximum at \[x=1\] and minimum at \[x=3.\]

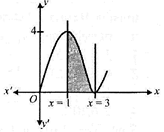\[\therefore \]Req. area \[=\int_{1}^{3}{x{{(3-x)}^{2}}dx}\]

\[=\int_{1}^{3}{({{x}^{3}}-6{{x}^{2}}+9x)dx}\]

\[=\left[ \frac{{{x}^{4}}}{4}-2{{x}^{3}}+\frac{9{{x}^{2}}}{2} \right]_{1}^{3}=4sq\,unit.\]