question_answer

Suppose we have a pavement all around the garden bed of which \[1\,m\] as shown below. The area of the pavement is:

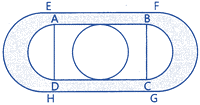A) \[(21+15\pi )\,{{m}^{2}}\]

B) \[(42+15\pi )\,{{m}^{2}}\]

C) \[(42-15\pi )\,m\]

D) \[(84+30\pi )\,{{m}^{2}}\]

Correct Answer: B

Solution :

We have \[FG=1+14+1=16m\]

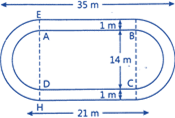Area of the pavement = Area of rectangle EFGH \[+2\times \] (Area of semi-circle with diameter \[16m\]) - (Area of rectangle \[ABCD+2\times \] (Area of semi-circle with diameter \[14m\])]

\[=EF\times FG+2\times \frac{1}{2}\pi {{\left( \frac{16}{2} \right)}^{2}}-\left[ AB\times BC+2\times \frac{1}{2}\pi {{\left( \frac{14}{2} \right)}^{2}} \right]\]\[=21\times 16+\pi \times {{(8)}^{2}}-[21\times 14+\pi +{{(7)}^{2}}]\]

\[=336+64\pi -294-49\pi =(42+15\pi ){{m}^{2}}\]

So, option [b] is correct.