question_answer

For a man, the angle of elevation of the highest point of the temple situated east of him is\[{{60}^{o}}\]. On walking 240 metres to north, the angle of elevation is reduced to\[{{30}^{o}}\], then the height of the temple is [MP PET 2003]

A) \[60\sqrt{6}m\]

B) \[60m\]

C) \[50\sqrt{3}m\]

D) \[30\sqrt{6}m\]

Correct Answer: A

Solution :

Total distance from temple = \[\sqrt{{{x}^{2}}+{{(240)}^{2}}}\] where \[x=\frac{h}{\tan {{60}^{o}}}\] = \[\frac{h}{\sqrt{3}}\]        So distance \[=\sqrt{\frac{{{h}^{2}}}{3}+{{(240)}^{2}}}\]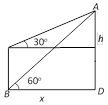 but \[\frac{h}{\sqrt{\frac{{{h}^{2}}}{3}+{{(240)}^{2}}}}=\frac{1}{\sqrt{3}}\] Þ \[\frac{{{h}^{2}}}{\frac{{{h}^{2}}}{3}+{{(240)}^{2}}}=\frac{1}{3}\] After solving, \[h=60\sqrt{6}\,\,m.\]