10th Class Mathematics Sample Paper

done Maths Olympiad Model Test Paper-7 Total Questions - 40    [Download]

• question_answer1)
  A rectangular courtyard 3.78 metre long and 5.25 metre wide is to be paved exactly with square tiles, all of the same size. What is the largest size of the tile which could be used for the purpose?
  A)
   14 cm             
  done clear
  B)
   21 cm
  done clear
  C)
   42 cm             
  done clear
  D)
   54 cm
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer2)
  The least number, which when divided by 48, 60, 72, 108 and 140 leaves 38, 50, 62, 98 and 130 as remainders respectively, is:
  A)
   11115             
  done clear
  B)
   15110
  done clear
  C)
   15120             
  done clear
  D)
   15210
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer3)
  If \[\alpha \] and \[\beta \] are the zeros of the polynomial \[f(x)={{x}^{2}}+ax-b,\] then the polynomial having zeros\[\frac{1}{\alpha }\]and\[\frac{1}{\beta }\].
  A)
   \[ab{{x}^{2}}+bx-a\]
  done clear
  B)
   \[b{{x}^{2}}-ax-1\]
  done clear
  C)
   \[ab{{x}^{2}}-bx+a\]
  done clear
  D)
   \[{{x}^{2}}+bx+a\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer4)
  If \[\alpha \] ,\[\beta \] and \[\gamma \] are the zeros of the polynomial \[2{{x}^{3}}-3{{x}^{2}}-23x+12,\] then the value of \[\frac{1}{\alpha }+\frac{1}{\beta }+\frac{1}{\gamma }\] is
  A)
   \[\frac{23}{12}\]          
  done clear
  B)
   \[-\,6\]
  done clear
  C)
   \[\frac{3}{2}\]                         
  done clear
  D)
   \[\frac{-\,23}{12}\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer5)
  If then nth term of an A.P. is 4n+1, then the common difference is:
  A)
   3                                
  done clear
  B)
   4
  done clear
  C)
   5                                
  done clear
  D)
   6
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer6)
  The condition for which the system of equations \[4x+ky+5=0\] and \[2x+3y+\text{7}=0\] has exactly one solution.
  A)
   \[k=6\]            
  done clear
  B)
   \[k\ne 6\]
  done clear
  C)
   \[k=3\]            
  done clear
  D)
   \[k\ne 3\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer7)
  Rs. 49 were divided among 150 children. Each girl got 50 paise and a boy 25 paise. How many boys were there?
  A)
   100                             
  done clear
  B)
   102
  done clear
  C)
   104     
  done clear
  D)
   105
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer8)
  The total cost of 4 soaps and 5 brushes is Rs. 123 and the total cost of 5 soaps and 4 brushes is Rs. 120. What is the cost of one soap?
  A)
   Rs. 10             
  done clear
  B)
   Rs. 12
  done clear
  C)
   Rs. 15             
  done clear
  D)
   Rs. 17
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer9)
  Solve: \[{{16.4}^{x+2}}-{{16.2}^{(x+1)}}+1=0\]
  A)
   \[x=5\]            
  done clear
  B)
   \[x=-\,5\]
  done clear
  C)
   \[x=4\]            
  done clear
  D)
   \[x=-\,4\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer10)
  If \[\left( x-3 \right)\] is the factor of \[3{{x}^{2}}-{{x}^{2}}+px+q,\]  then_______
  A)
   \[p+q=72\]                  
  done clear
  B)
   \[3p+q=72\]
  done clear
  C)
   \[3q+q=-\,72\]
  done clear
  D)
   \[q-3p=72\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer11)
  There are three consecutive positive integers such that the sum of the square of the first and the product of the other two is 277. Find the integers.
  A)
   12, 13, 14                    
  done clear
  B)
   13, 14, 15
  done clear
  C)
   11, 12, 13
  done clear
  D)
   9, 10, 13
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer12)
  Two medians AD and BE of \[\Delta \,ABC\] intersect at G at right angles. If AD = 9 cm and BE = 6 cm, then the length of BD, in cm, is
  A)
   10                               
  done clear
  B)
   6
  done clear
  C)
   5
  done clear
  D)
   3
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer13)
  A, B and C start at the same time in the same direction to run around a circular stadium. A completes a round in 252 seconds, B in 308 seconds and C in 198 seconds, all starting at the same point. After what time will they meet again at the starting point?
  A)
   26 minutes 18 seconds
  done clear
  B)
   42 minutes 36 seconds
  done clear
  C)
   45 minutes
  done clear
  D)
   46 minutes 12 seconds
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer14)
  If \[a+\frac{1}{a}+1=0,\] then find the value of\[{{a}^{4}}-\,a\].
  A)
   0        
  done clear
  B)
   1
  done clear
  C)
   2                                
  done clear
  D)
   \[-\,1\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer15)
  How many three digit numbers are divisible by 6?
  A)
   102                             
  done clear
  B)
   150
  done clear
  C)
   151                             
  done clear
  D)
   966
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer16)
  If the angle of depression from the top of a hill of two successive stones separated by a distance of 1 km are \[30{}^\circ \] and \[45{}^\circ \] respectively. The stones are at the east. Find the height of the hill?\[(take\,\,\sqrt{3}=1.732)\]
  A)
   916 m             
  done clear
  B)
   1366 m
  done clear
  C)
   812 m             
  done clear
  D)
   800 m
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer17)
  The angle of elevation of a pole from a point on the ground is \[15{}^\circ \]. Walking 10 meter towards the pole, the angle of elevation increases to\[30{}^\circ \]. What is the height of the pole (in meter)
  A)
   12 m               
  done clear
  B)
   10 m
  done clear
  C)
   5 m     
  done clear
  D)
   8 m
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer18)
  What should come next in the following letter series? AABABCABCDABCDEABCDEFABCDEF
  A)
   A                                
  done clear
  B)
   H
  done clear
  C)
   G        
  done clear
  D)
   B
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer19)
  Find the value of k so that the following system of linear equations has an infinite number of solutions: \[x+(k+2)y=4;\,\,(2k-1)x+25y=6k+2\]
  A)
   9                                
  done clear
  B)
   7
  done clear
  C)
   5                                
  done clear
  D)
   3
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer20)
  If \[{{\left( x+\frac{1}{x} \right)}^{2}}=3,\] then the value of,\[{{x}^{72}}+{{x}^{66}}+{{x}^{54}}+{{x}^{36}}+{{x}^{24}}+{{x}^{6}}+1.\]
  A)
   1        
  done clear
  B)
   2
  done clear
  C)
   3                                
  done clear
  D)
   4
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer21)
  The sum of 12 terms of an A.P., whose first term is 4, is 256. What is the last term?
  A)
   35                               
  done clear
  B)
   36
  done clear
  C)
   37                               
  done clear
  D)
   34
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer22)
  \[{{x}^{n}}-\,\,{{a}^{n}}\] is divisible by \[x-a\] for n is any ______
  A)
   Positive integer
  done clear
  B)
   Integer
  done clear
  C)
   Odd positive integer
  done clear
  D)
   Negative integer
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer23)
  If \[-\,5\] is a root of the quadratic equation \[2{{x}^{2}}+px-15=0\] and the quadratic equation \[p\,({{x}^{2}}+x)+k=0\] has equal roots, find the value of k.
  A)
   \[\frac{3}{5}\]                         
  done clear
  B)
   \[\frac{7}{4}\]
  done clear
  C)
   \[\frac{5}{3}\]                         
  done clear
  D)
   \[\frac{-\,7}{4}\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer24)
  Two dice are thrown simultaneously. What is the probability of getting two numbers whose product is even?
  A)
   \[\frac{1}{2}\]                         
  done clear
  B)
   \[\frac{3}{4}\]   
  done clear
  C)
   \[\frac{3}{8}\]                         
  done clear
  D)
   \[\frac{5}{16}\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer25)
  A bag contains 5 red balls and 8 blue balls. It also contains 4 green and 7 black balls. If a ball is drawn at random, then find the probability that it is not green.
  A)
   \[\frac{5}{6}\] 
  done clear
  B)
   \[\frac{1}{4}\]
  done clear
  C)
   \[\frac{1}{6}\]                         
  done clear
  D)
   \[\frac{7}{4}\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer26)
  A card is drawn from a packet of 100 cards numbered 1 to 100. The probability of drawing a number which is a square is:
  A)
   \[\frac{1}{10}\]
  done clear
  B)
   \[\frac{9}{100}\]
  done clear
  C)
   \[\frac{1}{100}\]                                  
  done clear
  D)
   \[\frac{2}{100}\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer27)
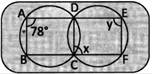
  In the given figure, find the value of the angles x and y.
  A)
   \[78{}^\circ ,\,\,102{}^\circ \] 
  done clear
  B)
   \[77{}^\circ ,\,\,103{}^\circ \]
  done clear
  C)
   \[70{}^\circ ,\,\,100{}^\circ \]             
  done clear
  D)
   \[75{}^\circ ,\,\,105{}^\circ \]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer28)
  In the adjoining figure. TAS is a tangent to the circle, with centre O, at the point A. If\[\angle OBA=32{}^\circ ,\] find the values of x and y.
  A)
   \[58{}^\circ ,\,\,58{}^\circ \]
  done clear
  B)
   \[64{}^\circ ,\,\,64{}^\circ \]
  done clear
  C)
   \[58{}^\circ ,\,\,64{}^\circ \]               
  done clear
  D)
   \[116{}^\circ ,\,\,64{}^\circ \]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer29)
  The slant height of a conical mountain is 2.5 km and the area of its base is \[1.54\text{ }k{{m}^{2}}\]. Find the height of the mountain.
  A)
   2.2 km            
  done clear
  B)
   2.4 km
  done clear
  C)
   3.05 km                       
  done clear
  D)
   3.11 km
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer30)
  Find the area of a triangle whose vertices are \[A\,\,\left( -\,8,-\,2 \right),\] \[B\,\,\left( -\,4,-\,6 \right)\] and \[C\,\,\left( -\,1,\,\,5 \right)\].
  A)
   56                               
  done clear
  B)
   48
  done clear
  C)
   36                               
  done clear
  D)
   28
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer31)
  For what value of ?m? the points \[A\,\,\left( m+1,\,\,1 \right),\]\[B\,\,\left( 2m\,\,+1,\,\,3 \right)\] and \[C\,\,\left( 2m+2,\,\,2m \right)\] are collinear.
  A)
   \[2\]                            
  done clear
  B)
   \[-\,\frac{1}{2}\]
  done clear
  C)
   \[\frac{3}{2}\]                         
  done clear
  D)
   Both A and B
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer32)
  Find the equation of a line, which whose portion intercepted between the coordinate axes is bisected at \[\left( 3,-\,2 \right)\].
  A)
   \[2x+y-6=0\]   
  done clear
  B)
   \[x+2y-6=0\]
  done clear
  C)
   \[2x-3y-12=0\]
  done clear
  D)
   \[2x+4y-12=0\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer33)
  If \[\sin \theta +\cos \theta =a\] and \[\sec \theta +\operatorname{cosec}\theta =b,\] then the value of \[b\,\,({{a}^{2}}-1)\] is equal to
  A)
   2a       
  done clear
  B)
   3a
  done clear
  C)
   0                                
  done clear
  D)
   2ab
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer34)
  A road which is 7 m wide surrounds a circular park whose circumference is 352 m. Find the area of the road.
  A)
   \[1246\,{{m}^{2}}\]               
  done clear
  B)
   \[2618\,{{m}^{2}}\]
  done clear
  C)
   \[3264\,{{m}^{2}}\]               
  done clear
  D)
   \[4460\,{{m}^{2}}\]
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer35)
  \[\frac{3+\cot 76{}^\circ +\cot 16{}^\circ }{\cot 76{}^\circ +\cot 16{}^\circ }\] is equal to
  A)
   \[\tan \,\,46{}^\circ \]               
  done clear
  B)
   \[\cot \,\,76{}^\circ \]
  done clear
  C)
   \[\cot \,\,44{}^\circ \]               
  done clear
  D)
   Both A and C
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer36)
  The height of a mountain is 90 meter. From the top of the mountain the angles of depression of top and foot of a tower are \[30{}^\circ \] and \[60{}^\circ \] respectively. Find the height of the tower? (in meter)
  A)
   45                               
  done clear
  B)
   60
  done clear
  C)
   75                               
  done clear
  D)
   30
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer37)
  In a row of girls, Shilpi is fifth from the left and Sukriti is sixth from the right. When the exchange their positions, then Shilpi becomes thirteenth from the left. What will be Sukriti's position from the right?
  A)
   7th                              
  done clear
  B)
   11th
  done clear
  C)
   14th    
  done clear
  D)
   18th
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer38)
  Find the missing number in the given figure.

  5	5	2
  2	4	1
  8	3	10
  40	3	?

  A)
   10                               
  done clear
  B)
   12
  done clear
  C)
   13                               
  done clear
  D)
   20
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer39)
  A person watches a car from a vertical observations tower and finds that the car is coming with the uniform speed towards the tower. If it takes 12 minutes to convert the angle of depression from \[30{}^\circ \]to \[45{}^\circ \]. Then what time it will take to reach the tower from initial place?
  A)
   26 minutes 23 seconds
  done clear
  B)
   28 minutes 23 seconds
  done clear
  C)
   24 minutes 35 seconds
  done clear
  D)
   25 minutes 49 seconds
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow

• question_answer40)
  The average of 5 consecutive odd numbers A, B, C, D and E is 55. What is the product of B & D?
  A)
   2915               
  done clear
  B)
   3127
  done clear
  C)
   3021   
  done clear
  D)
   2703
  done clear
  E)
   None of these
  done clear
  View Answer play_arrow