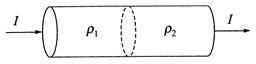
A) zero
B) \[\frac{({{\rho }_{1}}-{{\rho }_{2}})I{{\varepsilon }_{0}}}{2}\]
C) \[{{\varepsilon }_{0}}I|{{\rho }_{1}}-{{\rho }_{2}}|\]
D) \[{{\varepsilon }_{0}}I|{{\rho }_{1}}+{{\rho }_{2}}|\]
Correct Answer: C
Solution :
[c] Apply Gauss's law: \[\frac{{{q}_{in}}}{{{\varepsilon }_{0}}}~(outgoing\text{ }flux-incoming\text{ }flux)=\Delta (EA)\] \[V=IR=\frac{I\rho \ell }{A}\Rightarrow \frac{V}{\ell }A=I\rho \Rightarrow EA=I\rho \] \[\Rightarrow \,\,\,\frac{{{q}_{in}}}{{{\varepsilon }_{0}}}=I|{{\rho }_{1}}-{{\rho }_{2}}|\,\,\,\,\Rightarrow \,\,\,\,{{q}_{in}}=I{{\varepsilon }_{0}}|{{\rho }_{1}}-{{\rho }_{2}}|\]You need to login to perform this action.
You will be redirected in
3 sec
