A) 0
B) 1
C) 2
D) 4
Correct Answer: C
Solution :
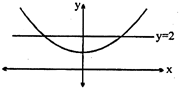 \[\frac{dy}{dx}=x+xy\Rightarrow \,\frac{dy}{dx}-xy=x\] Integrating factor \[={{e}^{\frac{-{{x}^{2}}}{2}}}\] \[\therefore \,\,y.{{e}^{\frac{-{{x}^{2}}}{2}}}\,=\int_{{}}^{{}}{x{{e}^{\frac{-{{x}^{2}}}{2}}}\,dx=-{{e}^{\frac{-{{x}^{2}}}{2}}}+C}\] \[\Rightarrow \,y=C.{{e}^{\frac{{{x}^{2}}}{2}}}\,-1\] At \[x=0,\,\,y=0\Rightarrow \,C=1\] \[\therefore \,\,f(x)={{e}^{\frac{{{x}^{2}}}{2}}}\,-1\] So, \[f(x)=1\Rightarrow \,{{e}^{\frac{{{x}^{2}}}{2}}}=2\] \[\Rightarrow \] Number of solution is 2.
\[\frac{dy}{dx}=x+xy\Rightarrow \,\frac{dy}{dx}-xy=x\] Integrating factor \[={{e}^{\frac{-{{x}^{2}}}{2}}}\] \[\therefore \,\,y.{{e}^{\frac{-{{x}^{2}}}{2}}}\,=\int_{{}}^{{}}{x{{e}^{\frac{-{{x}^{2}}}{2}}}\,dx=-{{e}^{\frac{-{{x}^{2}}}{2}}}+C}\] \[\Rightarrow \,y=C.{{e}^{\frac{{{x}^{2}}}{2}}}\,-1\] At \[x=0,\,\,y=0\Rightarrow \,C=1\] \[\therefore \,\,f(x)={{e}^{\frac{{{x}^{2}}}{2}}}\,-1\] So, \[f(x)=1\Rightarrow \,{{e}^{\frac{{{x}^{2}}}{2}}}=2\] \[\Rightarrow \] Number of solution is 2.
You need to login to perform this action.
You will be redirected in
3 sec
