question_answer

In a ball, a person receives direct sound waves from a source 120 m away. He also receives wave from the same source which reach him after being reflected from one 25 m high ceiling at a point half-way between them. The two waves interfere constructively for wavelengths (in metre) of

A)  \[10,5,\,\frac{5}{2},\,...\]                   

B)  \[20,\,\frac{20}{3},\,\frac{20}{5},\,...\]

C)  30, 20, 10, ?            

D)  35, 25, 15, ?

Correct Answer: B

Solution :

 As in \[\frac{Q}{4\pi {{R}^{2}}}=\frac{q}{4\pi {{r}^{2}}}=\sigma \]            \[\therefore \] \[V=\frac{1}{{{\varepsilon }_{0}}}\,[\sigma R+\sigma r]=\frac{\sigma }{{{\varepsilon }_{0}}}[R+r]\]             Path difference \[{{N}_{S}}=\left( \frac{\rho S}{\rho P} \right)\times {{N}_{P}}\] \[=\frac{(4.4\times {{10}^{3}})\,\times 100}{220}=2000\]          \[{{\rho }_{S}}\] \[{{\rho }_{P}}\]        \[-h=-u{{t}_{1}}+\frac{1}{2}gt_{1}^{2}\] But at A, the wave suffers reflection at the surface of rigid/fixed end or denser medium, hence the wave must suffer an additional path change of \[-h=-u{{t}_{2}}+\frac{1}{2}gt_{2}^{2}\] or a phase change of \[0=u({{t}_{2}}-{{t}_{1}})+\frac{1}{2}g(t_{1}^{2}-t_{2}^{2})\].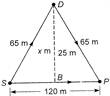 Net path difference \[u=\frac{1}{2}g({{t}_{1}}+{{t}_{2}})\] For maximum, net path difference \[h=\frac{g{{t}_{1}}{{t}_{2}}}{2}\]. \[\sqrt{2}\,mv\] \[\sqrt{2}\,mv'=(2m)\,v\] or         \[\Rightarrow \] \[v'\frac{v}{\sqrt{2}}\]            \[E=\frac{1}{2}mv{{'}^{2}}+\frac{1}{2}mv{{'}^{2}}+\frac{1}{2}(2m){{v}^{2}}\] \[=mv{{'}^{2}}+m{{v}^{2}}\]   \[\Rightarrow \]