question_answer

A  variable point \[P\]on the ellipse of eccentricity \[e\] is joined to the foci \[S\]and \[S'.\]if the locus of the incentre of the triangle \[PSS'\] is a conic of eccentricity \[{{e}_{1}},\]then\[\frac{2}{e_{1}^{2}}-\frac{1}{e}\]equals to

A) 5

B) \[2\]

C) 1

D) -1

Correct Answer: C

Solution :

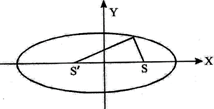Let the ellipse be\[\frac{{{x}^{2}}}{{{a}^{2}}}+\frac{{{y}^{2}}}{{{b}^{2}}}=1,\]

Foci are \[S\left( ae,0 \right)\] and \[S'\left( -ae,0 \right)\]

Let \[P(a\cos \theta ,b\sin \theta )\] be any point on the ellipse.

Then \[SP=a-e\left( a\cos \theta  \right)=a\left( 1-e\cos \theta  \right)\] and \[S'P=a+e(a\cos \theta )=a(1+e\cos \theta )\]

Also, \[SS'=2ae\]

Let \[(h,k)\] be the in Centre of the \[\Delta PSS',\]

Then \[(-ae.a(1-e\cos \theta )+ae.a(1+e\cos \theta )\]

\[h=\frac{+a\cos \theta .2ae)}{a(1-e\cos \theta )+a(1+e\cos \theta )+2ae}=ae\cos \theta .\]

\[k=\frac{b\sin \theta .2ae}{a(1-e\cos \theta )+a(1+e\cos \theta )+2ae}=\frac{b\sin \theta .e}{1+e}\]

\[\therefore \]\[\cos \theta =\frac{h}{ae},\sin \theta =\frac{(1+e)k}{eb}\]

\[\Rightarrow \]\[\frac{{{h}^{2}}}{{{a}^{2}}{{e}^{2}}}+\frac{{{(1+e)}^{2}}{{k}^{2}}}{{{e}^{2}}{{b}^{2}}}=1\]

Hence locus of \[\left( h,k \right)\] is \[\frac{{{x}^{2}}}{{{a}^{2}}{{e}^{2}}}+\frac{{{y}^{2}}}{{{e}^{2}}{{b}^{2}}/\left( 1+{{e}^{2}} \right)}=1,\]

Which is an ellipse,

Its eccentricity \[e_{1}^{2}=1-\frac{{{e}^{2}}{{b}^{2}}}{{{e}^{2}}{{a}^{2}}{{(1+e)}^{2}}}\] \[=1-\frac{{{a}^{2}}(1-{{e}^{2}})}{{{a}^{2}}{{(1+e)}^{2}}}=\frac{2e}{1+e}\Rightarrow \frac{2}{e_{1}^{2}}-\frac{1}{e}=1\]