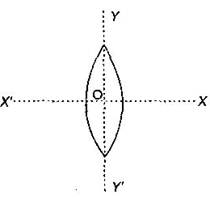
Choose the correct statement from the following:
A) \[f'=f,\,\,f''=f\]
B) \[f'=2f,\,\,f''=2f\]
C) \[f'=f,\,\,f''=2f\]
D) \[f'=2f,\,\,f''=f\]
Correct Answer: C
Solution :
Initially, the focal length of equiconvex lens is \[\frac{1}{f}=(\mu -1)\,\left( \frac{1}{{{R}_{1}}}-\frac{1}{{{R}_{2}}} \right)\] ....(i) \[\frac{1}{f}=(\mu -1)\,\left( \frac{1}{R}-\frac{1}{-R} \right)=\frac{2\,(\mu -1)}{R}\] Case I: When lens is cut along XOX?, then each half is again equiconvex with \[{{R}_{1}}=+R,\,{{R}_{2}}=-R\] Thus, \[\frac{1}{f}=(\mu -1)\,\left[ \frac{1}{R}-\frac{1}{(-R)} \right]\] \[=(\mu -1)\,\left[ \frac{1}{R}+\frac{1}{R} \right]\] \[=(\mu -1)\,\frac{2}{R}=\frac{1}{f}\] \[\Rightarrow f'=f\] Case II: When lens is cut along YOY?, then each half becomes plano-convex with \[{{R}_{1}}=+R,\,{{R}_{2}}=\infty \] Thus, \[\frac{1}{f''}=(\mu -1)\,\left( \frac{1}{{{R}_{1}}}+\frac{1}{{{R}_{2}}} \right)\] \[=(\mu -1)\,\left( \frac{1}{R}-\frac{1}{\infty } \right)\] \[=\frac{(\mu -1)}{R}=\frac{1}{2f}\] Hence, \[f'=f,\,f''=2f\]
You need to login to perform this action.
You will be redirected in
3 sec
