question_answer

The length of the longest size rectangle of maximum area that can be inscribed in a semicircle of radius 1, so that 2 vertices lie on the diameter, is:

A)  \[\sqrt{2}\]       

B)         \[2\]

C)  \[\sqrt{3}\]                       

D)         \[\frac{\sqrt{2}}{3}\]

E)  \[\frac{-2}{\sqrt{3}}\]

Correct Answer: A

Solution :

                Let the length and breadth of the rectangle be \[l\]and b. In\[\Delta OAB,\]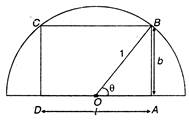                 \[b=\sin \theta \]             and        \[l=2\cos \theta \] \[\therefore \]  A = Area of rectangle \[=lb=2\text{ }sin\theta \text{ }cos\theta \] \[=sin2\theta \] On differentiating w.r.t.\[\theta \], we get \[\frac{dA}{d\theta }=2\cos 2\theta \] Put\[\frac{dA}{d\theta }=0\]for maxima or minima \[\Rightarrow \]               \[\cos 2\theta =0\] \[\Rightarrow \]               \[\theta =\frac{\pi }{4}\] \[\therefore \]  \[\frac{{{d}^{2}}A}{d{{\theta }^{2}}}=-4\sin 2\theta \]                 \[{{\left( \frac{{{d}^{2}}A}{d{{\theta }^{2}}} \right)}_{\theta =\frac{\pi }{4}}}<0\]               \[\therefore \]Function is maximum at\[\theta =\frac{\pi }{4}\]. \[\therefore \]Length of rectangle\[=2\cos \theta =2\cos \frac{\pi }{4}\]                 \[=2\frac{1}{\sqrt{2}}=\sqrt{2}\]