question_answer

A block of weight crests on a horizontal floor with coefficient of static friction \[\mu .\]It is desired to make the block move by applying minimum amount of force. The angle \[\theta \] from the horizontal at which the force should be applied and magnitude of the force F are respectively.     JEE Main  Online Paper (Held On 19  May  2012)

A) \[\theta ={{\tan }^{-1}}\left( \mu  \right),F=\frac{\mu W}{\sqrt{1+{{\mu }^{2}}}}\]

B)                        \[\theta ={{\tan }^{-1}}\left( \frac{1}{\mu } \right),F=\frac{\mu W}{\sqrt{1+{{\mu }^{2}}}}\]

C)                        \[\theta =0,F=\mu W\]

D)                        \[\theta ={{\tan }^{-1}}\left( \frac{\mu }{1+\mu } \right),F=\frac{\mu W}{1+\mu }\]

Correct Answer: A

Solution :

                Let the force F is applied at an angle \[\theta \] with the horizontal.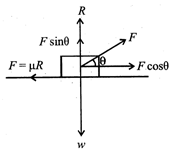 For horizontal equilibrium,\[F\cos \theta =\mu R\]?(i) For vertical equilibrium, \[R+F\sin \theta =mg\]or\[R=mg-F\sin \theta \]               ?(ii) Substituting this value of R in eq. (i), we get \[F\cos \theta =\mu (mg-F\sin \theta )\] \[=\mu \,mg-\mu \,F\sin \theta \] or,\[=F(\cos \theta +\mu \sin \theta )=\mu mg\] or,\[F=\frac{\mu mg}{\cos \theta +\mu \sin \theta }\]                                   ?(iii) For F to be minimum, the denominator \[(\cos \theta +\mu \sin \theta )\] should be maximum. \[\therefore \]\[\frac{d}{d\theta }(\cos \theta +\mu \sin \theta )=0\] or\[-\sin \theta +\mu \cos \theta =0\]or\[\tan \theta =\mu \] or\[\theta ={{\tan }^{-1}}(\mu )\] Then, \[\sin \theta =\frac{\mu }{\sqrt{1+{{\mu }^{2}}}}\] and\[\cos \theta =\frac{1}{\sqrt{1+{{\mu }^{2}}}}\] Hence, \[=\frac{\mu }{\frac{1}{\sqrt{1+{{\mu }^{2}}}}+\frac{1}{\sqrt{1+{{\mu }^{2}}}}}=\frac{\mu w}{\frac{1}{\sqrt{1+{{\mu }^{2}}}}}\]