2nd Class Mathematics Division

Division

Synopsis  

Division:

• Division is the reverse process of multiplication. It means grouping or sharing. It is also called repeated subtraction. - is the symbol for division.
• Equal sharing or grouping means to divide the things equally without anything being left over or remaining.
• The number which is divided is called the dividend. The number which divides the dividend is called the The number of times the divisor divides the dividend is called the quotient. The number of the dividend left undivided is called the remainder.

e.g.,    In 20 \[\div \] 5 = 4, 20 is the dividend, 5 is the divisor and 4 is quotient when 20 is divided exactly by 5. So, remainder is 0.

• 35 \[\div \] 3 can be written as \[\frac{35}{3}\].
• Dividing 0 by a number gives 0. g., \[\frac{0}{5}=0\]
• A number cannot be divided by 0. g., \[\frac{3}{0}\to \]cannot be done.
• Dividing a number by 1 gives the same number as quotient, g., \[\frac{7}{1}=7\]
• Dividing a number by itself gives 1 as quotient, g., \[\frac{6}{6}=1\]
• Division of a 1 - digit number by another 1 - digit number, e.g., 9 \[\div \] 3 = 3.
• Division of a 2 -digit number by a 1 - digit number, e.g., Divide 67 by 4.

Step-1: 67 \[\div \] 4

Write the given division as shown.  

Divisor \[\leftarrow \] 

Step - 2:  Starting from the left most digit, find the number of times the divisor is in the digit.

There is one 4 in 6.

Put this 1 in quotient and 4 below 6 as shown.

Quotient

Step - 3: Now, subtract 4 from 6 in the dividend and write the difference 2 below the

Separator line

Step - 4: Bring down the next digit (ie., 7) beside 2 as shown.   

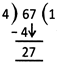
Find the number of 4's in the new dividend 27.

4 \[\times \] 6 = 24 and 4 \[\times \]7 = 28

So, there are 6 fours in the dividend 27. Write 6 beside 1 in the quotient and 24 below 27 as shown. Subtract 24 from 27 and write the difference 3 below the

separator line shown.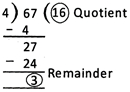  

Step - 5: The number 3 < 4 and 4 cannot divide 3. So, 3 remains undivided and is called the remainder. Stop the division when the remainder is less than the divisor.  

Therefore, in 67 \[\div \] 4:

67 is dividend; 4 is divisor; 16 is quotient and 3 is remainder.

• 44 \[\div \] 5 = 8 R 4 is a division statement.
• For every division fact, there are two multiplication facts.

40 \[\div \] 5 = 8 \[\to \] a) 40 = 5 \[\times \] 8   b) 40 = 8 \[\times \]5

• For every multiplication fact there are two division facts.

5 \[\times \]8 = 40 \[\to \] a) 40 \[\div \] 5 = 8   b) 40 \[\div \]8 = 5