9th Class Mathematics Number System and its Operations Number Systems

Number Systems

• Rational numbers (Q): The numbers of the form,\[\frac{p}{q}\] where 'p' and 'q' are integers and are called rational     number A numfaer of the form r is a fraction. So all fractions are rational numbers.

            Note: A number of the form \[\frac{\mathbf{a}}{\mathbf{b}}\] is a fraction. So all are rational             numbers. in the fraction, ‘a’     is called the numbers and ‘b’ is called the denominator. e.g.             \[\frac{\mathbf{1}}{\mathbf{2}}\mathbf{-            }\frac{\mathbf{2}}{\mathbf{3}}\mathbf{,}\frac{\mathbf{7}}{\mathbf{6}}\mathbf{,}\frac{\mat            hbf{6}}{\mathbf{11}}\mathbf{,-}\frac{\mathbf{2}}{\mathbf{9}}\mathbf{,}....\]

            (i) Zero is a rational number.

            Note: 0 by 0 is undefined.

            (ii) Every integer is a rational number.

            (iii) A rational number, may or may not be an integer.

            (iv) To write W distinct rational numbers between any two rational numbers 'a' and \['b'\],

            we write\[a=\frac{{{P}_{1}}}{q}\] and\[b=\frac{{{P}_{2}}}{q}\] such that \[\left( {{P}_{2}}\text{ }-            \text{ }{{P}_{1}} \right)\]is a positive integer greater than \['n'\],

            (Here a < b); \[{{P}_{1}},{{P}_{2}}\]and q are integers\[(q\ne 0)\].

            p- +1 p. + 2     Pi + n

            A set of n rational numbers can be written as

            \[\frac{{{P}_{1}}+1}{q},\frac{{{P}_{2}}+2}{q},......,\frac{{{P}_{1}}+n}{q}\]

            i.e.,\[a=\frac{{{p}_{1}}}{q}<\frac{{{p}_{1}}+1}{q}<\frac{{{p}_{1}}+2}{q}.....<\frac{{{p}_{1}}+            n}{q}<\frac{{{p}_{2}}}{q}=b\]

            Between two given rational numbers a and b, there are infinitely many rational numbers.

• Properties of rational number

            (i) If \[\frac{p}{q}\], is a rational number and \['m'\] is a non-zero integer, then \[\frac{p}{q}=\frac{p\times             m}{q\times m}\]                                            

• (ii) If q is a rational number and \['m'\] is a common divisor of p and q, then \[\frac{p}{q}=\frac{p\div m}{q\div m}\]

            (iii) Two rational numbers are equivalent only when the product of the numerator of the first rational             number and the denominator of the second is equal to the product of the denominator of the first and the             numerator of the second.

• Thus,\[\frac{p}{q}=\frac{r}{s}\operatorname{only}\,if\,p\times s=q\times r\]

            Note \[\frac{\mathbf{-p}}{\mathbf{q}}\mathbf{=}\frac{\mathbf{p}}{\mathbf{-q}}\mathbf{=-            }\frac{\mathbf{p}}{\mathbf{q}}\]

• Representation of rational numbers on a number line:

            (i) Rational numbers of the form \[\frac{m}{n}\] where m < n are represented on the number line as     shown below.

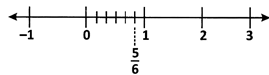
(ii) Rational numbers of the form \[\frac{m}{n}\] where m > n are represented on the number line as    shown below.

• Irrational numbers (Q'): Any number which cannot be expressed in the form of. (Which is neither terminating nor repeating decimal) where p and q are integers and \[q\ne 0\]is said to be an irrational            

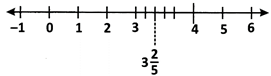
            Note:\[\pi \] is an irrational number.

• Representation of an irrational number on a number line:

• In the above figure, Q represents \[\sqrt{3}\] on the number line.

• Important properties of rational & irrational numbers:

            (i) The sum of two rational numbers is a rational number.

            (ii) The sum of two irrational numbers may or may not be irrational.

            (ii) The product of two rational numbers is a rational number,

            (iv) The product of two irrational numbers may or may not be irrational.

            (v) The difference of two rational numbers is a rational number.

            (vi) The sum of a rational number and an irrational number is an irrational number.

            (vii) The difference of a rational number and an irrational number is an irrational number.

            (viii) The product of a rational number and an irrational number is an irrational number.

            (ix) The quotient of two rational numbers is a rational number.

            \[\operatorname{Exception}:\frac{\operatorname{Any} rational number}{0}=\infty \]                                                   (x) The quotient of two irrational numbers may or may not be irrational.

            (xi) The quotient of a rational number and an irrational number is an irrational number.

            \[\operatorname{Exception}:\frac{0}{\operatorname{Any} rational number}=\infty \]

• Real numbers (R): The union of all rational and irrational numbers is called the set of real numbers.

            Definition: A number whose square is non-negative is called a real number.

            Note:    (i) Every point on the number line, represents either a rational number or an             irrational number i.e., a real number.

            (ii) Corresponding to every point on the number line. There is a unique real number. And             Corresponding to every real number, there is a unique point on the number line.

• Decimal expansion of real numbers:

            (i) Every rational number\[\frac{p}{q}\left( p,q\in Z\,\operatorname{and}\,q\ne 0 \right)\] can be             expressed in the form of terminating or non-           terminating recurring decimal.

            (ii) Every irrational number can be expressed as a non-terminating non-recurring decimal.

            Square root of a given positive real number: Let \['a'\] be any positive real number. We can express             \[\sqrt{a=b}\]    if and only if b > 0 and \[{{b}^{2}}\]= a. The value of \['b'\]is called the positive square             root of the positive real number 'a'.

• Some results on square roots:

            (i) \[{{\left( \sqrt{\operatorname{x}} \right)}^{2}}=\operatorname{x}\]) Where x is a positive real             number.

            (ii)\[\sqrt{\operatorname{x}}\times \sqrt{\operatorname{y}}=\sqrt{\operatorname{xy}}\] Where x and y             are positive real numbers.

            (iii)             \[\frac{\sqrt{\operatorname{x}}}{\sqrt{\operatorname{y}}}=\sqrt{\frac{\operatorname{x}}{\operatorna            me{y}}}\]where x and y are positive real numbers.

• (iv)\[\left( \sqrt{\operatorname{x}}+\sqrt{\operatorname{y}} \right)\times \left( \sqrt{\operatorname{x}}- \sqrt{\operatorname{y}} \right)=\operatorname{x}-y\] where x and y are positive real numbers.

            (v)\[{{\left( \sqrt{\operatorname{x}}+\sqrt{\operatorname{y}}             \right)}^{2}}=\operatorname{x}+y+2\sqrt{\operatorname{xy}}\,\operatorname{and}{{\left(             \sqrt{\operatorname{x}}-\sqrt{\operatorname{y}} \right)}^{2}}=x+y-2\sqrt{xy}\]  Where x and y are             positive real numbers.

            (vi)

            \[(\sqrt{a}+\sqrt{b})\times             (\sqrt{\operatorname{c}}+\sqrt{d})=\sqrt{ac}+\sqrt{ad}+\sqrt{bc}+\sqrt{bd}\]where a, b, c and d are             positive real numbers.

            where 'a' is any real number and 'b' is a positive real number.

• Rationalizing factor: In expressions like \[\frac{a}{\sqrt{x}},\frac{1}{\operatorname{a}+b\sqrt{x}},\frac{1}{\sqrt{x}+\sqrt{y}},\operatorname{a            nd}\frac{1}{a\sqrt{\operatorname{x}}\times b\sqrt{\operatorname{y}}}\]make the denominators       free             from square roots            such as             \[\sqrt{\operatorname{x}}\,\operatorname{and}\,\sqrt{\operatorname{y}}\]we multiply the    Numerator             and denominator by a suitable      This factor is called the rationalizing factor.

• Rationalizing the denominator: The process of converting the denominator of an expression containing a term with a square root to an equivalent expression where denominator is a rational number is called             rationalizing the denominator.

• Rationalizing factors:

            (i) The rationalizing factor             of\[\frac{a}{\sqrt{\operatorname{x}}}\operatorname{is}\sqrt{\operatorname{x}}\].

            (ii) The rationalizing factor of\[\frac{1}{a+b\sqrt{x}}\,\operatorname{is}\,\,\operatorname{a}-b\sqrt{x}\].

            (iii) The rationalizing factor of             \[\frac{1}{a\sqrt{\operatorname{x}}+b\sqrt{\operatorname{y}}}\,\operatorname{is}\,\,\operatorname{a            }\sqrt{x}-b\sqrt{\operatorname{y}}\]

            (iv)The rationalizing factor of\[\frac{1}{a-b\sqrt{\operatorname{x}}}\operatorname{is}\left(             a+b\sqrt{\operatorname{x}} \right)\].

            (v) The rationalizing factor of\[\frac{1}{a\sqrt{\operatorname{x}}-            b\sqrt{\operatorname{y}}}\operatorname{is}\,\operatorname{a}\sqrt{x}+b\sqrt{y}\].

• Laws of exponents for real numbers: If 'a' is any real number and W is a natural number

            (positive integer), then\[{{a}^{n}}=a\times a\times ........\times a\](\['n'\] factors). Here, (\[{{a}^{n}}\])             is called the \[{{n}^{th}}\]power of a. The real     number 'a' is called the base and positive integer 'n' is             called the exponent.

            For any rational base a; and any integers 'm' and 'n', we have

            (i)\[{{a}^{m}}\times {{a}^{n}}={{a}^{m+n}}\]                   (ii) \[{{\left( {{a}^{m}}             \right)}^{n}}={{a}^{mn}}\]

            (iii) \[\frac{{{a}^{m}}}{{{a}^{n}}}={{a}^{m-n}};m>n\]      (iv) \[{{a}^{n}}\times             {{b}^{n}}={{\left( a\times b \right)}^{n}}\]

            (v) \[\frac{{{a}^{n}}}{{{b}^{n}}}=\left( \frac{{{a}^{n}}}{{{b}^{n}}} \right);b\ne 0\]           (vi)             \[\frac{{{a}^{n}}}{{{b}^{n}}}=\left( \frac{{{a}^{n}}}{{{b}^{n}}} \right);b\ne 0\]

            (vii) \[{{a}^{o}}=1\]                (viii) \[\frac{1}{{{a}^{n}}}={{a}^{-n}}\]

            (ix) \[{{a}^{-n}}=\frac{1}{{{a}^{n}}}={{\left( \frac{1}{a} \right)}^{n}}\]

• \[{{n}^{th}}\]root of a positive real number: For any natural number n > 2, we define \[\sqrt[n]{a}=b\]as the root of      positive real number 'a' if\[{{b}^{n}}\]\[=a,\,\,and\,b>0\]
• Laws of rational exponents of positive real numbers: For a positive real number 'a'; and any positive integers 'm' and 'n' \[\left( \ne 0 \right)\], such that 'm' and 'n' have no common factor other than 1,we have

            Note: For any positive real number a

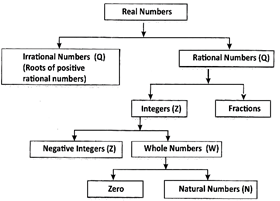
            Overall view of the number system: