Notes - Syllogism
Category : 12th Class
Syllogism
Introduction: Items based on Logical Reasoning are indispensable feature of all competitive examinations these days to test a candidate’s basic intelligence and aptitude. Syllogism is an important section of logical reasoning and hence a working knowledge of its rules is required on the part of the candidate. The term syllogism means inference or deduction drawn from the given statements.
The questions of syllogism can be solved with the help of Venn-diagrams and some rule devised with the help of analytical ability. Some people are of the opinion that Venn-diagram can be of great use for solving questions of syllogism. No doubt a few questions can be solved with the help of Venn-diagrams but van-diagrams alone do not help the students to solve variety of questions of syllogism. Even common sense also will not be much help in working out certain working out certain difficult type of questions. Only the basic concepts and rules, which have a bearing on reasoning faculty could alone help. We have discussed these rule with illustrations throughout the chapter. To proceed further for the study of rules, we must first know some terminology used in syllogism.
Proposition
Proposition, also know a premises, is a grammatical sentence which comprises a subject, a Predicate and a copula. A subject is that which affirms or denies a fact. Predicate is a terms which states something about a subject and copula establishes relationship between the subject and the predicate.
Example: I. All ![]()
II. No ![]()
III. Some ![]()
Classification of Propositions
A proposition can mainly be divided into three categories:
(a) Categorical Proposition: In categorical propositions, there exists a relationship between the subject and the predicate without any condition. It means predicate is either affirmation or denial of the subject unconditionally.
Examples: I. All cups are plates.
II. No Girl is boy.
(b) Hypothetical Proposition: In a hypothetical proposition, relationship between subject and predicate is asserted conditionally.
Examples: I. If it rains, he will not come
II. If he comes, I will accompany him.
(c) Disjunctive Proposition: In a disjunctive proposition, the assertion is of alteration.
Examples: I. Either he is honest or he is loyal.
II. Either he is educated or he is illiterate.
Keeping in view the existing pattern of syllogism in competitive examinations we are concerned only with the categorical type of proposition.
Types of Categorical Proposition
Categorical propositions have been classified on the basis of quality and quantity of the propositions. Quality denotes whether the proposition is affirmative or negative. Quality denotes whether the proposition is affirmative or negative. Quantity represents whether the propositions is universal or particular.
The four-fold classification of categorical propositions can be summarized as under:
|
Symbol |
Proposition |
Quantity |
Quality |
|
A |
All A and B |
Universal |
Affirmative |
|
E |
No A is B |
Universal |
Negative |
|
I |
Some A are B |
Particular |
Affirmative |
|
O |
Some A are not B |
Particular |
Negative |
To draw valid inferences from the statement the candidate is required to have a clear understanding of A, E, I, O relationships.
Venn-Diagram Representation of Four Propositions
1. Universal Affirmative (a): All S are P
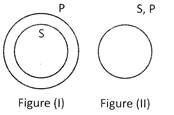
There are two possibilities to represent the relation between S and P given by universal affirmative proposition "All S are P". This can also be understood with the help of set theory.
Case I. \[S=\left\{ a,\text{ }b,\text{ }c \right\},~~~~~P=\left\{ a,\text{ }b,\text{ }c,\text{ }d \right\}\]
Case II. \[S=\left\{ a,\text{ }b,\text{ }c \right\},~~~~~P=\left\{ a,\text{ }b,\text{ }c \right\}\]
Case I is represented by Figure (I) and Case II is represented by Figure (18). In both these cases, we see that every element of set S is also the element of set P. Hence, we can definitely say that the above two figures shows "All S are P".
2. Universal Negative (e): No S are P
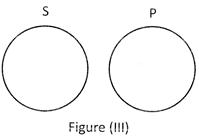
There is only one possibility of drawing the relationship between S and P.
\[S=\left\{ a,\text{ }b,\text{ }c \right\},\text{ }P=\left\{ d,\text{ }e,\text{ }f \right\}\]
From the above two sets it is clear that none of the elements of S is the element of set P.
3. Particular Affirmative (I): Some S are P

There are three possible representations given by figure (IV), figure (V) and figure (VI) depicting particular affirmative proposition “Some S are P”. This can be supported with the help of following sets:
Case I. \[S\text{ }\left\{ a,\text{ }b,\text{ }c,\text{ }d \right\},~\] \[P=\left\{ c,\text{ }d,\text{ }e,f \right\}\]
Case II. \[S=\left\{ a,\text{ }b \right\},\] \[P=\left\{ a,\text{ }b,\text{ }c,\text{ }d \right\}\]
Case III. \[S=\left\{ a,\text{ }b,\text{ }c,\text{ }d \right\},~\] \[P=\left\{ a,\text{ }b \right\}\]
In all the three cases we find that some of the elements of S are also the elements of set P.
4. Particular Negative (O): Same S are not P
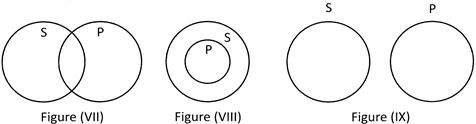
The particular negative proposition “Some S are P” are be represented with the help of three possible Figure given in (VII), (VIII) and (IX).
Venn-diagram representations of the above propositions can be supported by way of following sets:
Case I. \[S=\left\{ a,\text{ }b,\text{ }c \right\}\] \[P=\left\{ c,\text{ }d,\text{ }e \right\}\]
Case II \[S=\left\{ a,\text{ }b,\text{ }c,\text{ }d \right\}\] \[P=\left\{ c,\text{ }d \right\}\]
Case III. \[S=\left\{ a,\text{ }b,\text{ }c \right\}\] \[P=\left\{ d,\text{ }e,\text{ }f \right\}\]
In all the three cases, we find that there are some elements in set S which are not elements of set P. Hence, all the cases along with the respective figures support the proposition “Some S are not P”.
Hidden Proposition
The type of proposition we have discussed so far are of standard nature. But there are propositions which do not appear in standard format, and yet can be classified under any of the four types. Let us only discuss the type of such propositions.
I A - type Propositions:
(i) All positive propositions beginning with ‘every’, ‘each’ ‘any’ are W type propositions
Examples:
(a) Every cot is mat
\[\Rightarrow \] All cots are mats.
(b) Each of students of class 12th has passed.
\[\Rightarrow \] All students of class 12th have passed
(c) Anyone can do this job
\[\Rightarrow \] All (men) can do this job
(ii) A positive sentence with a particular person as its subject is always an A type proposition.
Examples:
(a) He can qualify the SSC written test
(b) Mahatma Gandhi is known as the father of the nation.
II E - Type Propositions:
(i) All negative sentences beginning with ‘no one’, ‘none’, ‘not a single’, etc. are E-type propositions.
Examples:
(a) Not a single student could answer the question.
(b) None can cross the English channel.
(ii) A negative sentence with a very definite exception is also of E-type propositions.
Example:
Not student except Ram has failed.
(iii) When a interrogative sentence is used to make an assertion, this could be reduced to an E-type proposition.
Example: Is there any person who can scale Mount Everest?
\[\Rightarrow \] None can climb Mount Everest.
(III) I- type Propositions:
(i) Positive propositions beginning with words such as ‘most’, ‘a few’, ‘mostly’ ‘generally’, ‘almost’, ‘frequently’, often’ are to be reduced to the I-type.
Examples:
(a) Almost all the fruits have been sold.
\[\Rightarrow \] Some fruits have been sold
(b) Most of the student will qualify in the examination.
\[\Rightarrow \] Some of the student will qualify in the examination.
(c) Girls are frequently physically weak.
\[\Rightarrow \] Some girls are physically weak.
(ii) Negative propositions beginning with words such as ‘few’, ‘seldom’, ‘hardly’, ‘scarcely’, ‘rarely’, ‘little’ etc. are to be reduced to I-type.
Examples:
(a) Seldom players do not take rest
\[\Rightarrow \] Some players take rest.
(b) Few priests do not tell a lie.
\[\Rightarrow \] Some priests tell a lie.
(c) Rarely IT professional do not get a good job
\[\Rightarrow \] Some IT professionals get a good job
(iii) A positive sentence with an exception which is not definite, is reduced to an I-type proposition.
Examples:
(a) All students except three have passed.
\[\Rightarrow \] Some student have passed.
(b) All innocents except a few are guilty.
\[\Rightarrow \] Some innocents are guilty.
VI O-type Propositions:
(i) All negative propositions beginning with words such as ‘all’, ‘every’, ‘any’, ‘each’ etc. are to be reduced to O-type propositions.
Examples:
(a) All innocent are not guilty.
\[\Rightarrow \] Some innocents are not guilty
(b) All that glitters is not gold.
\[\Rightarrow \] Some glittering objects are not
(c) Everyone is not present.
\[\Rightarrow \] Some are not present.
(ii) Negative propositions with words as ‘most’, ‘a few’, ‘mostly’, ‘generally’, ‘almost’, ‘frequently’ are to be reduced to the O-type propositions.
Examples:
(a) Girls are usually not physically weak.
\[\Rightarrow \] Some girls are not physically weak.
(b) Priests are not frequently thiefs.
\[\Rightarrow \] Some priests are not thiefs,
(c) Almost all the questions can’t be solved.
\[\Rightarrow \] Some questions can’t be solved.
(iii) Positive propositions with starting words such as ‘few’, ‘seldom’, ‘hardly’, ‘scarcely’, ‘rarely’, ‘little’, etc. are to be reduced to the O-type.
Examples:
(a) Few girls are intelligent.
\[\Rightarrow \] Some girls are not intelligent.
(b) Seldom are innocents guilty.
\[\Rightarrow \] Some innocent are not guilty.
(iv) A negative sentence with an exception, which is not definite, is to reduced to the O-type.
Examples:
(a) No student except Ram has passed.
\[\Rightarrow \] Some students have not passed.
(b) Non girls except a few are intelligent.
\[\Rightarrow \] Some girls are not intelligent.
Types of Inference
Inference drawn from statements can be of two types:
Example: Statement: All Books are Pages.
Conclusion: Some Pages are Books.
In the above example, a conclusion is drawn from a single statement and does not require the second statement to be referred, hence the inference is called an immediate inference.
Example: Statement: All Dogs are Cats
All Cats are Black
Conclusion: All Dogs are Black
In the above example, conclusion is drawn from the two statements or in other words, both the statements are required to draw the conclusion. Hence, the above conclusion is known as mediate inference.
Methods to Drawn Inference
(I) Immediate Inference: There are various methods to draw immediate inference like conversion, observation, contraposition, etc. Keeping in view the nature of questions asked in various competitive examinations, we are required to study two methods-implications and conversion.
(a) Implications (of a given proposition): Below we shall discuss the implications of all the four types of propositions.
While drawing a conclusion through implications, subject remains the subject and predicate remains the predicate.
A-Type: All boys are blue. From the above A type proposition, it is very clear that if all boys are blue, then some boys will definitely be blue because some is a part of all. Hence, from A type proposition, we can draw I type conclusion (through implication).
E-Type: No cars are buses. If no cars are buses, it clearly means that some cars are not buses.
Hence, from E-type proposition, O-type conclusion (through implication) can be drawn.
I-Type: Some chairs are tables. From the above 1-type propositions, we cannot draw any valid conclusion (through implication) From the above O-type proposition, we cannot drawn any valid inference (through implication). On first look, it appears that if some A are not B, then conclusion that some A are B must be true but the possibility of this conclusion being true can overruled with the help of following example:
Case I: \[A=\left\{ a,\text{ }b,\text{ }c \right\},\] \[B=\left\{ d,\text{ }e,\text{ }f \right\}\]
Case II: \[~A\text{ }=\text{ }\left\{ a,\text{ }b,\text{ }c \right\},\] \[B=\left\{ b,\text{ }c,\text{ }d \right\}\]
The above two cases show the relationship between A and B given by O-type proposition “Some A are not B”.
Now, in case I, none of the element of set A is the element of set B. Hence, conclusion “Some A are B” can’t be valid. However, in case (II) elements b, c are common to both sets A and B. Hence, here conclusion “Some A are B” is valid. But for any conclusion to be true, it should be true for all the cases. Hence/ conclusion “Some A are B” is not a valid conclusion drawn from an O-type proposition.
All the results derived for immediate inference through implication can be presented in the table as below:
|
Types of Proposition |
Proposition |
Types of Inference |
Inference |
|
A |
All S are P |
I |
Some S are P |
|
E |
No S is P |
O |
Some S are not P |
|
I |
Some S are P |
- |
- |
|
O |
Some S are not P |
- |
- |
(b) Conversion: In conversion, while drawing inference, subject and predicate of a proposition are interchanged, i.e. subject becomes the predicate and predicate becomes the subject but the quality of the proposition remains the same i.e., the affirmative remains affirmative and the negative remains negative.
In conversion, A-type proposition is converted into I-type E-type can be converted into E-type, I-type converted into I-type and O-type proposition can’t be converted.
Examples:
|
(I) |
Statement: All lamps are mangoes. |
(A-type) |
|
|
Conclusion: Some mangoes are lamps. |
(I-type) |
|
(II) |
Statement: No men are wise. |
(E-type) |
|
|
Conclusion: No wise are men. |
(E-type) |
|
(III) |
Statement: Some chairs are tables. |
(I-type) |
|
|
Conclusion: Some tables are chairs. |
(I-type) |
For making the things clear, we combining and presenting the results of implication and conversion in the following table. This will help the candidates, by and large, in drawing immediate inferences from a given proposition quickly.
|
Type of Preposition |
Preposition |
Valid Inferences |
Type of Inference |
Method |
|
A |
All S and P |
Some S are P Some P are S |
I I |
Implication |
|
E |
No S are P |
Some P are not S No P are S |
O E |
Conversion |
|
I |
Some A are B |
Some B are A |
I |
Conversion |
|
O |
Some A are not B |
No valid inference |
- |
- |
It is important to note here that only valid inference given in the above table can be drawn from the four types of propositions. Hence, the candidate are advised to make, themselves familiar with types of proposition (A, E, I, O) and to remember the results of immediate inferences as given above for solving question quickly.
Three-Statement Syllogism
In these types of questions, three statements are given in place of two statements. Though the principle involved in solving these questions are same yet a slightly different approach is adopted. Follow examples will help to understand the pattern of these questions and methods to solve them.
Example: 1
Statement: 1. All bags are caps.
2. Some pens are bags.
3. No caps are desks.
Conclusions: I. Some pens are caps.
II. No desks are bags.
III. Some pens are desks.
Solution: The first step in solving these questions is to select two statements for each of the conclusions in such a way that subject and predicate of the conclusion should be present in these statement. And, importantly, these statement should be linked with “common term”.
Conclusion I: For conclusion (I) “ Some pens are caps”, we can take statement (1) and (2) because these two statement have subject “pens” and predicate “caps” of the statement and are linked with the common term “bags”. Now to draw mediate inference these two statements have to be aligned in such a way that predicate of the first statement is the subject of the second statement. Hence, these two statement can be aligned as follows:
Some pens are bags. (I type)

All bags are caps. (A type)
From the table of mediate inference, we know that \[I+A=L\] Therefore, conclusion will be ‘Some pens are caps.” Hence, conclusion I is valid.
Conclusion II: Statement (1) and (3) are relevant for conclusion II “No desks are bags” These two statement are already aligned.
All bags are caps. (A type)
No caps are desks. (E type)
We know that \[A+E=E\]. Therefore, from these two statements, conclusion “No bags are desks” are valid. But our conclusion is “No desks are bags” We know that E type proposition can be converted into E type proposition, i.e., “No bags desks” \[\Rightarrow \] No desks are bags” Hence, conclusion (II) valid.
Conclusion III: For this conclusion, we cannot choose any two statements because out of three, no two statement containing subject and predicate of the conclusion are linked with the common term. Therefore, all the three statements are relevant to test this inference. For this, we should write the three statements in such a way that the predicate of the first is the subject of second and predicate for second is the subject of the third.
|
Some pens are bags. |
(I type) |
|
II bags are caps. |
(A type) |
|
No caps are desks. |
(E type) |
From the combination of all these statements, we find that \[I+A+E=(I+A)+E=I+E=O\]
Thus, a valid conclusion would be of O-type, i.e., “Some pens are not desk” Hence, conclusion ill “Some pens desks” is not valid conclusion.
Conclusion IV: Conclusion IV “Some caps are bags” is immediate inference drawn from statement (1). From the rule of conversion, we know “All bags are caps”
\[\Rightarrow \] “Some caps are bags” Hence, conclusion (IV) is valid.
Example: 2
Statement: 1. All boys are jokers.
2. Some men are boys.
3. All jokers are caps.
Conclusion: I. Some caps are boys.
II. Some men are caps.
III. All boys are caps.
Solution: For conclusion (I), (III) and (IV), subject and predicate “caps” “boys”. And for these conclusions, relevant statements would be (1) and (3) because these two statements have “caps” and “boys” and are linked with the common term “jokers”.
Now, as per the rule of mediate inference, we know that
All boys are jokers. (A type)
All jokers are caps. (A type)
|
All boys are jokers. |
(A type) |
|
All jokers are caps. |
(A type) |
\[A+A=A\]. Therefore, conclusion “All boys are caps” is valid. From the rule of conversion we know that “All boys are caps” “Some caps are boys.” Hence, conclusion (I) and (III) are valid and conclusion (IV) is invalid.
For conclusion (II), subject is “men” and predicate is “caps”. These two terms are available in Statement (2) and (3) but these statements are not linked with common term. Therefore, all the three statements are relevant. Now, after aligning all the three statements, we get

All boys are jokers. (A type)

All jokers are caps. (A type)
\[I+A+A~~\Rightarrow ~~\left( I+A \right)+A~~~\Rightarrow ~~~~I+A=I.\]
Thus, conclusion ?Some men are caps?.
Hence, in the above examples, conclusion (i), (ii) and (iii) are valid.
Snap Test
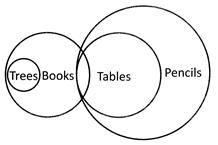
Some books are tables.
All tables are pencils.
Conclusions:
I. Some Pencil are tabl
II. Some books are trees
III. Some tables are trees
(a) Only I and II follow (b) Only II and III follow
(c) Only III follow (d) All follow
(e) None of these
Explanation: Option (a) is correct.
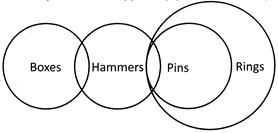
2. Statement: Some boxes are hammers.
Some hammers are pins.
All pins are rings.
Conclusions: I. Some rings are hammers.
II. Some hammers are boxes
III. Some rings are boxes.
(a) All follow (b) Only I and II follow
(c) Only II and III follow (d) Only III follow
(e) None of these
Explanation: So only conclusion (I) and (II) are correct. Option (b) is correct.
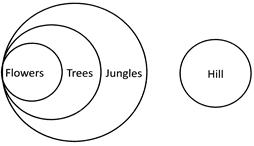
3. Statement: All flowers are trees
All trees are jungles
No jungles are flowers
Conclusions: I. No flowers is hill
II. No free is hill
III. Some jungles are flowers.
(a) All follow (b) Only I and II follow
(c) Only II and III follow (d) Only III follow
(e) None of these
Explanation: Option (a) is correct.
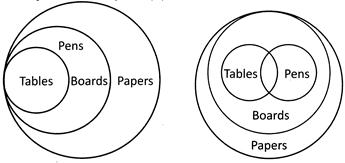
Statement: All tables are boards
All pens are boards
All boards are papers
Conclusions: I. Some pens are tables
II. Some papers are pens
III. No pen is table
(a) All follow (b) Only I and II follow
(c) Only II and III follow (d) Only III follow
(e) None of these
Explanation: Option (c) is correct.
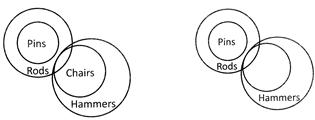
5. Statements: All pins are rods.
Some rods are chairs
All chairs are hammers
Conclusion: I. Some pins are hammers
II. Some hammers are rods
III. No pin is hammer
(a) All follow (b) Only I and II follow
(c) Only II and III Follow (d) Only III follow
(e) None of these
Explanation: Option (b) is correct.
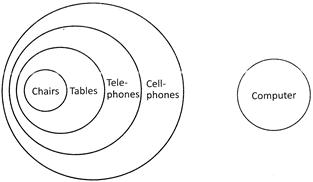
6. Statements: All chairs are table.
All tables are telephones.
All telephones are cellphones.
No cellphone is computer.
Conclusion: I. All cellphones are tables.
II. Some chairs are computers
III. No computer is chairs
(a) All follow (b) Only I and II follow
(c) Only II and III follow (d) Only III follow
(e) None of these
Explanation: Option (d) is correct.
You need to login to perform this action.
You will be redirected in
3 sec
