JEE Main & Advanced Physics Semiconducting Devices Constants of Triode Valve

(1) Plate or dynamic resistance \[({{r}_{P}})\]

(i) The slope of plate characteristic curve is equal to \[\frac{1}{\text{plate}\,\text{resistance}}\] or It is the ratio of small change in plate voltage to the change in plate current produced by it, the grid voltage remaining constant. That is, \[{{r}_{p}}=\frac{\Delta {{V}_{\text{p}}}}{\Delta {{i}_{p}}},{{V}_{G}}=\text{constant}\].

(ii) It is expressed in kilo ohms \[(K\Omega )\]. Typically, it ranges from \[8K\Omega \] to\[40K\Omega \]. The \[{{r}_{P}}\] can be determined from plate characteristics. It represents the reciprocal of the slope of the plate characteristic curve. (iii) If the distance between plate and cathode is increased the \[{{r}_{P}}\] increases. The value of \[{{r}_{P}}\] is infinity in the state of cut off bias or saturation state.

(2) Mutual conductance (or trans conductance) \[({{g}_{m}})\]

(i) It is defined as the ratio of small change in plate current \[(\Delta {{i}_{p}})\] to the corresponding small change in grid potential \[(\Delta {{V}_{g}})\] when plate potential \[{{V}_{p}}\] is kept constant i.e. \[{{g}_{m}}={{\left( \frac{\Delta {{i}_{p}}}{\Delta {{V}_{g}}} \right)}_{{{V}_{p\,}}\text{is}\,\text{constant}}}\]

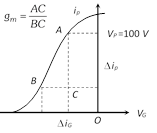
(ii) The value of \[{{g}_{m}}\] is equal to the slope of mutual characteristics of triode.

(iii) The value of \[{{g}_{m}}\] depends upon the separation between grid and cathode. The smaller is this separation, the larger is the value of \[{{g}_{m}}\] and vice versa. (iv) In the saturation state, the value of \[\Delta {{i}_{p}}=0\], \[{{g}_{m}}=0\]

(3) Amplification factor \[(\mu )\] : It is defined as the ratio of change in plate potential \[(\Delta {{V}_{p}})\] to produce certain change in plate current \[(\Delta {{i}_{p}})\] to the change in grid potential \[(\Delta {{V}_{g}})\] for the same change in plate current \[(\Delta {{i}_{p}})\]i.e. \[\mu =-\,{{\left( \frac{\Delta {{V}_{p}}}{\Delta {{V}_{g}}} \right)}_{\Delta {{I}_{p}}=\,\text{a constant }}}\]; negative sign indicates that \[{{V}_{p}}\] and \[{{V}_{g}}\] are in opposite phase. (i) Amplification factor depends upon the distance between plate and cathode \[({{d}_{pk}}),\] plate and grid \[{{d}_{pg}}\] and grid and cathode \[({{d}_{gk}})\]. i.e. \[\mu \,\propto \,{{d}_{pg}}\propto \,{{d}_{pk}}\propto \frac{1}{{{d}_{gk}}}\] (ii) The value of \[\mu \] is greater than one. (iii) Amplification factor is unitless and dimensionless.

(4) Relation between triode constants : The triode constants are not independent of each other. They are related by the relation \[\mu ={{r}_{p}}\times {{g}_{m}}\] The \[{{r}_{p}}\] and \[{{g}_{m}}\] depends on \[{{i}_{p}}\] in the following manner \[{{r}_{p}}\propto {{i}_{p}}^{-1/3}\], \[{{g}_{m}}\propto {{i}_{p}}^{1/3}\], \[\mu \] does not depend on \[{{i}_{p}}\].

Above three constants may be determined from any one set of characteristic curves.

\[{{r}_{p}}=\frac{{{V}_{P1}}-{{V}_{P2}}}{{{I}_{PA}}-{{I}_{PB}}}\], \[{{g}_{m}}=\frac{{{I}_{PA}}-{{I}_{PB}}}{{{V}_{G1}}-{{V}_{G2}}}\], \[\mu =-\frac{{{V}_{P1}}-{{V}_{P2}}}{{{V}_{G2}}-{{V}_{G1}}}\]