Solved papers for JEE Main & Advanced Physics Thermodynamical Processes JEE PYQ-Thermodynamical Processes

done JEE PYQ-Thermodynamical Processes Total Questions - 83

• question_answer1) Even Carnot engine cannot give 100% efficiency because we cannot             [AIEEE 2002]
  A)
   prevent radiation           
  done clear
  B)
   find ideal sources
  done clear
  C)
   reach absolute zero temperature
  done clear
  D)
   eliminate friction
  done clear
  View Answer play_arrow

• question_answer2) “Heat cannot be itself flow from a body at lower temperature to a body at higher temperature” is a statement or consequence of                                                                                        [AIEEE 2003]
  A)
   second law of thermodynamics
  done clear
  B)
   conservation of momentum
  done clear
  C)
                                           conservation of mass
  done clear
  D)
   first law of thermodynamics
  done clear
  View Answer play_arrow

• question_answer3) During an adiabatic process, the pressure of a gas is found to be proportional to the cube of its absolute temperature. The ratio, \[{{C}_{p}}/{{C}_{V}}\] for the gas is                                                                             [AIEEE 2003]
  A)
   4/3
  done clear
  B)
                    2    
  done clear
  C)
   5/3      
  done clear
  D)
        3/2
  done clear
  View Answer play_arrow

• question_answer4) A Carnot engine takes \[3\times {{10}^{6}}\] cal of heat from a reservoir at \[{{627}^{o}}C\] and gives it to a sink at \[{{27}^{o}}C\]. The work done by the engine is                                                              [AIEEE 2003]
  A)
   \[42\times {{10}^{6}}\]J       
  done clear
  B)
        \[8.4\times {{10}^{6}}\]J
  done clear
  C)
   \[16.8\times {{10}^{6}}\]      
  done clear
  D)
        Zero
  done clear
  View Answer play_arrow

• question_answer5) Which of the following statements is correct for any thermodynamic system?                           [AIEEE 2004]
  A)
   The internal energy changes in all processes
  done clear
  B)
   Internal energy and entropy are state functions
  done clear
  C)
   The change in entropy can never be zero
  done clear
  D)
   The work done in an adiabatic process is always zero
  done clear
  View Answer play_arrow

• question_answer6) Which of the following is incorrect regarding the first law of thermodynamics?      [AIEEE 2005]
  A)
   It is not applicable to any cyclic process
  done clear
  B)
   It is a restatement of the principle of conservation of energy
  done clear
  C)
   It introduces the concept of the internal energy
  done clear
  D)
  None of these
  done clear
  View Answer play_arrow

• question_answer7) The temperature-entropy diagram of a reversible engine cycle is given in the figure. Its efficiency is         [AIEEE 2005]
  A)
   1/2      
  done clear
  B)
        1/4    
  done clear
  C)
   1/3
  done clear
  D)
        2/3
  done clear
  View Answer play_arrow

• question_answer8) 

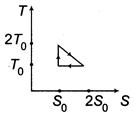
  A system goes from A to B via two processes and II as shown in figure. Ifandare the changes in internal energies in the processes I and II respectively, then              [AIEEE 2005]

  A)
   \[\Delta {{U}_{1}}=\Delta {{U}_{2}}\]
  done clear
  B)
   relation between\[\Delta {{U}_{1}}\]and\[\Delta {{U}_{2}}\]cannot be determined
  done clear
  C)
   \[\Delta {{U}_{2}}>\Delta {{U}_{1}}\]
  done clear
  D)
   \[\Delta {{U}_{2}}<\Delta {{U}_{1}}\]
  done clear
  View Answer play_arrow

• question_answer9) Two rigid boxes containing different ideal gases are placed on a table. Box A contains one mole of nitrogen at temperature to, while box B contains one mole of helium at temperature (7/3)\[{{T}_{0}}\]. The boxes are then put into thermal contact with each other and heat flows between them until the gases reach a common final temperature (ignore the heat capacity of boxes). Then, the final temperature of the gases,\[{{T}_{f}}\]in terms of\[{{T}_{0}}\]is                                                        [AIEEE 2006]
  A)
   \[{{T}_{f}}=\frac{3}{7}{{T}_{0}}\]   
  done clear
  B)
        \[{{T}_{f}}=\frac{7}{3}{{T}_{0}}\]
  done clear
  C)
   \[{{T}_{f}}=\frac{3}{2}{{T}_{0}}\]   
  done clear
  D)
        \[{{T}_{f}}=\frac{5}{2}{{T}_{0}}\]
  done clear
  View Answer play_arrow

• question_answer10) The work of 146 kJ is performed in order to compress one kilo mole of a gas adiabatically and in this process, the temperature of the gas increases   by\[7{}^\circ C\].   The   gas   is \[(R=8.3\,J\,mo{{l}^{-1}}{{K}^{-1}})\]                   [AIEEE 2006]
  A)
   Diatomic
  done clear
  B)
   triatomic
  done clear
  C)
   a mixture of monatomic and diatomic
  done clear
  D)
   monatomic
  done clear
  View Answer play_arrow

• question_answer11) A Carnot engine, having an efficiency of\[(-\pi /2,\pi /2)\]1/10 as heat engine, is used as a refrigerator. If the work done on the system is 10 J, the amount of energy absorbed from the reservoir at lower temperature is                                 [AIEEE 2007]
  A)
   99 J    
  done clear
  B)
       90   
  done clear
  C)
   1 J      
  done clear
  D)
        100 J
  done clear
  View Answer play_arrow

• question_answer12) If\[min\{x+1,|~x|+1\}\]and\[f\{x\}\ge 1\]denote the specific heats of nitrogen per unit mass at constant pressure and constant volume respectively, then                         [AIEEE 2007]
  A)
   \[x\in R\]
  done clear
  B)
        \[f(x)\]
  done clear
  C)
   \[x=1\]
  done clear
  D)
        \[f(x)\]
  done clear
  View Answer play_arrow

• question_answer13) 

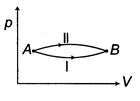  When a system is taken from state\[E={{E}_{0}}\]to state f along the path iaf, it is found that\[sin\text{ }\omega t\] and\[I={{I}_{0}}\sin \left( \omega t-\frac{\pi }{2} \right)\]. Along the path ibf, \[p=\frac{{{E}_{0}}{{I}_{0}}}{\sqrt{2}}\]cal. W along the path ibf is              [AIEEE 2007]

  A)
   6 cal               
  done clear
  B)
        16 cal
  done clear
  C)
   66 cal           
  done clear
  D)
        14 cal
  done clear
  View Answer play_arrow

• question_answer14) 

  Directions: Question numbers 14 - 16 are based on the following paragraph.

  Two moles of helium gas are taken over the cycle ABCDA, as shown in the P-T diagram.

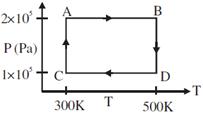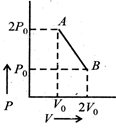
  Assuming the gas to be ideal the work done on the gas in taking it from A to B is:                    [AIEEE 2009]

  A)
   200 R  
  done clear
  B)
        300 R
  done clear
  C)
   400 R  
  done clear
  D)
        500 R
  done clear
  View Answer play_arrow

• question_answer15) The work done on the gas is taking it from D to A is                                    [AIEEE 2009]
  A)
   - 414 R
  done clear
  B)
        + 414 R
  done clear
  C)
   - 690 R            
  done clear
  D)
        + 690 R
  done clear
  View Answer play_arrow

• question_answer16) The net work done on the gas in the cycle ABCDA is                                  [AIEEE 2009]
  A)
   zero                 
  done clear
  B)
        276 R
  done clear
  C)
   1076 R            
  done clear
  D)
        1904 R
  done clear
  View Answer play_arrow

• question_answer17) A diatomic ideal gas is used in a Carnot engine as the working substance. If during the adiabatic expansion part of the cycle the volume of the gas increases from V to 32 V, the efficiency of the engine is-                                       [AIEEE 2010]
  A)
   0.25    
  done clear
  B)
        0.5
  done clear
  C)
  0.75                       
  done clear
  D)
        0.99
  done clear
  View Answer play_arrow

• question_answer18) A carnot engine operation between temperatures \[{{T}_{1}}\] and \[{{T}_{2}}\] has efficiency\[\frac{1}{6}\]. When \[{{T}_{2}}\] is lowered by 62 K, its efficiency increases to \[\frac{1}{3}\]. Then \[{{T}_{1}}\] and \[{{T}_{2}}\] are respectively                                                        [AIEEE 2011]
  A)
   310 K and 248 K
  done clear
  B)
        372 K and 310 K
  done clear
  C)
   372 K and 330 K
  done clear
  D)
        330 K and 268 K
  done clear
  View Answer play_arrow

• question_answer19) A thermally insulated vessel contains an ideal gas of molecular mass M and ratio of specific heats\[\gamma \]. It is moving with speed v and is suddenly brought to rest. Assuming no heat is lost to the surroundings, its temperature increases by[AIEEE 2011]
  A)
   \[\frac{(\gamma -1)}{2R}M{{\upsilon }^{2}}K\]
  done clear
  B)
        \[\frac{(\gamma -1)}{2(\gamma +1)R}M{{\upsilon }^{2}}K\]
  done clear
  C)
   \[\frac{(\gamma -1)}{2\gamma R}M{{\upsilon }^{2}}K\]
  done clear
  D)
        \[\frac{\gamma M{{\upsilon }^{2}}}{2R}K\]
  done clear
  View Answer play_arrow

• question_answer20)  

  The specific heat capacity of a metal at low temperature (T) is given as :

  \[{{C}_{p}}(kj{{K}^{-1}}k{{g}^{-1}})=32{{\left( \frac{T}{400} \right)}^{3}}\]

  A 100 gram vessel of this metal is to be cooled from 20°K to 4°K by a special refrigerator operating at room temperature (27°C). The amount of work required to cool the vessel is:                 [AIEEE 11-05-2011]

  A)
   greater than 0.148 kJ     
  done clear
  B)
                    between 0.148 kJ and 0.028 kJ
  done clear
  C)
   less than 0.028 kJ
  done clear
  D)
                             equal to 0.002 kJ
  done clear
  View Answer play_arrow

• question_answer21) A container with insulating walls is divided into equal parts by a partition fitted with a valve. One part is filled with an ideal gas at a pressure P and temperature T, whereas the other part is completely evacuated. If the valve is suddenly opened, the pressure and temperature of the gas will be:                                    [AIEEE 11-05-2011]
  A)
  \[\frac{P}{2},\frac{T}{2}\]                    
  done clear
  B)
  \[P,T\]
  done clear
  C)
  \[P,\frac{T}{2}\]                       
  done clear
  D)
       \[\frac{P}{2},T\]
  done clear
  View Answer play_arrow

• question_answer22) 

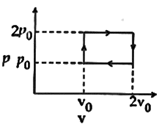
  Helium gas goes through a cycle ABCDA (consisting of two isochoric and isobaric lines) as shown in figure. Efficiency of this cycle is nearly:                                                                                                          [AIEEE 2012]

  (Assume the gas to be close to ideal gas)

  A)
   15.4%
  done clear
  B)
        9.1%
  done clear
  C)
   10.5%
  done clear
  D)
        12.5%
  done clear
  View Answer play_arrow

• question_answer23) A Carnot engine, whose efficiency is 40%, takes in heat from a source maintained at a temperature of 500K. It is desired to have an engine of efficiency 60%. Then, the intake temperature for the same exhaust (sink) temperature must be:                  [AIEEE 2012]
  A)
   efficiency of carnot engine cannot be made larger than 50%
  done clear
  B)
   1200 K
  done clear
  C)
   750 K  
  done clear
  D)
        600 K
  done clear
  View Answer play_arrow

• question_answer24) 

  This question has Statement 1 and Statement 2.                 [JEE ONLINE 07-05-2012]

  Of the four choices given after the Statements, choose the one that best describes the two Statements.

  Statement 1: In an adiabatic process, change in internal energy of a gas is equal to work done on/ by the gas in the process.

  Statement 2: The temperature of a gas remains constant in an adiabatic process.

  A)
   Statement 1 is true. Statement 2 is true, Statement 2 is a correct explanation of Statement 1.
  done clear
  B)
   Statement 1 is true. Statement 2 is false.
  done clear
  C)
    Statement 1 is false, Statement 2 is true.
  done clear
  D)
   Statement 1 is false. Statement 2 is true, Statement 2 is not a correct explanation of Statement 1.
  done clear
  View Answer play_arrow

• question_answer25) A given ideal gas with \[\gamma =\frac{{{C}_{p}}}{{{C}_{v}}}=1.5\] at a temperature T. If the gas is compressed adiabatically to one-fourth of its initial volume, the final temperature will be                                                               [JEE ONLINE 12-05-2012]
  A)
  \[2\sqrt{2}T\]    
  done clear
  B)
        4 T
  done clear
  C)
   2 T     
  done clear
  D)
        8 T
  done clear
  View Answer play_arrow

• question_answer26) 

  n moles of an ideal gas undergo a process \[A\to B\]as shown in the figure. Maximum temperature of the gas during the process is                                                                                               [JEE ONLINE 12-05-2012]

  A)
  \[\frac{8{{P}_{0}}{{V}_{0}}}{nR}\]    
  done clear
  B)
       \[\frac{3{{P}_{0}}{{V}_{0}}}{2nR}\]
  done clear
  C)
  \[\frac{9{{P}_{0}}{{V}_{0}}}{2nR}\]  
  done clear
  D)
       \[\frac{9{{P}_{0}}{{V}_{0}}}{4nR}\]
  done clear
  View Answer play_arrow

• question_answer27) 

  This question has Statement 1 and Statement 2.

  Of the four choices given after the Statements, choose the one that best describes the two Statements.

  Statement 1: An inventor claims to have constructed an engine that has an efficiency of 30% when operated between the boiling and freezing points of water. This is not possible.

  Statement 2: The efficiency of a real engine is always less than the efficiency of a Carnot engine operating between the same two temperatures.                                                                         [JEE ONLINE 19-05-2012]

  A)
         Statement 1 is true, Statement 2 is true, and Statement 2 is not the correct explanation of Statement 1.
  done clear
  B)
        Statement 1 is true. Statement 2 is false.
  done clear
  C)
     Statement 1 is false, Statement 2 is true.
  done clear
  D)
        Statement 1 is true. Statement 2 is true, Statement 2 is the correct explanation of Statement 1.
  done clear
  View Answer play_arrow

• question_answer28) The pressure of an ideal gas varies with volume as \[P=\alpha V,\] where a is a constant. One mole of the gas is allowed to undergo expansion such that its volume becomes 'm times its initial volume. The work done by the gas in the process is                                                                                                                                     [JEE ONLINE 19-05-2012]
  A)
  \[\frac{\alpha V}{2}\left( {{m}^{2}}-1 \right)\]
  done clear
  B)
                   \[\frac{{{\alpha }^{2}}{{V}^{2}}}{2}\left( {{m}^{2}}-1 \right)\]
  done clear
  C)
  \[\frac{\alpha }{2}\left( {{m}^{2}}-1 \right)\]
  done clear
  D)
                   \[\frac{\alpha {{V}^{2}}}{2}\left( {{m}^{2}}-1 \right)\]
  done clear
  View Answer play_arrow

• question_answer29) The door of a working refrigerator is left open in a well-insulated room. The temperature of air in the room will       [JEE ONLINE 26-05-2012]
  A)
   decrease
  done clear
  B)
   increase in winters and decrease in summers
  done clear
  C)
   remain the same           
  done clear
  D)
   Increase
  done clear
  View Answer play_arrow

• question_answer30) An ideal monatomic gas with pressure P, volume F and temperature T is expanded isothermally to a volume 2V and a final pressure \[{{P}_{i}}.\] If the same gas is expanded adiabatically to a volume 2V, the P final pressure is \[{{P}_{a}}.\]The ratio \[\frac{{{P}_{a}}}{{{P}_{i}}}\]is                                                                                                                                  [JEE ONLINE 26-05-2012]
  A)
  \[{{2}^{-1/3}}\]                      
  done clear
  B)
       \[{{2}^{1/3}}\]
  done clear
  C)
  \[{{2}^{2/3}}\]                       
  done clear
  D)
       \[{{2}^{-2/3}}\]
  done clear
  View Answer play_arrow

• question_answer31) 

  The above \[p-v\] diagram represents the thermodynamic cycle of an engine, operating with an ideal monoatomic gas. The amount of heat, extracted from the source in a single cycle is:                                  [JEE MAIN 2013]

  A)
   \[{{p}_{0}}{{v}_{0}}\]           
  done clear
  B)
        \[\left( \frac{13}{2} \right){{p}_{0}}{{v}_{0}}\]
  done clear
  C)
   \[\left( \frac{11}{2} \right){{p}_{0}}{{v}_{0}}\]
  done clear
  D)
        \[4{{p}_{0}}{{v}_{0}}\]
  done clear
  View Answer play_arrow

• question_answer32) 

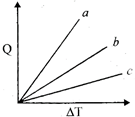
  Figure shows the variation in temperature \[(\Delta T)\]with the amount of heat supplied \[(Q)\] in an isobaric process corresponding to a monatomic (M), diatomic  and a polyatomic (P) gas. The initial state of all the gases are the same and the scales for the two axes coincide, ignoring vibration degrees of freedom, the lines a, b and c respectively correspond to: [JEE ONLINE 09-04-2013]

  A)
         P, M and D                  
  done clear
  B)
  M, D and P
  done clear
  C)
   P, D and M      
  done clear
  D)
        D, M and P
  done clear
  View Answer play_arrow

• question_answer33)            

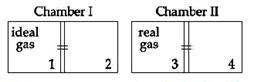  There are two identical chambers, completely thermally insulated from surroundings. Both chambers have a partition wall dividing the chambers in two compartments. Compartment 1 is filled with an ideal gas and compartment 3 id filled with a real gas. Compartments 2 and 4 are vacuum. A small hole (orifice) is made in the partition walls and the gases are allowed to expand in vacuum.

  Statement-1: No change in the temperature of the gas takes place when ideal as expands in vacuum. However, the temperature of real gas goes down (cooling) when it expands it expands in vacuum.

  Statement-2: The internal energy of an ideal gas is only kinetic. The internal energy of a real gas is kinetic as well as potential.                                                                                    [JEE ONLINE 09-04-2013]

  A)
         Statement 1 is false and Statement 2is true
  done clear
  B)
     Statement 1 and Statement 2 bath are true. Statement 2 is the correct explanation of Statement 1.
  done clear
  C)
        Statement 1 is true and Statement 2 if false.
  done clear
  D)
  Statement 1 and Statement 2 both are true. Statement 2 is not the correct explanation of Statement 1.
  done clear
  View Answer play_arrow

• question_answer34) An ideal gas at atmospheric pressure is adiabatically compressed so that its density becomes 32 times of its initial value. If the final pressure of gas is 128 atmospheres, the value of \['\gamma '\] the gas is:  [JEE ONLINE 22-04-2013]
  A)
   1.5                  
  done clear
  B)
       1.4
  done clear
  C)
   1.3      
  done clear
  D)
                   1.6
  done clear
  View Answer play_arrow

• question_answer35) A certain amount of gas is taken through a cyclic process (A B C D A) that has two isobars, one isochore and one isothermal. The cycle can be represented on a P-V indicator diagram as:                        [JEE ONLINE 22-04-2013]
  A)
   
  done clear
  B)
        
  done clear
  C)
   
  done clear
  D)
      
  done clear
  View Answer play_arrow

• question_answer36) Given that 1g of water in liquid phase has volume \[\text{1c}{{\text{m}}^{3}}\]and in vopour phase \[\text{1671}\,\,\text{c}{{\text{m}}^{3}}\] at atmospheric pressure and the latent heat of vaporization of water is 2256 J/g; the change in the internal energy in Joules for 1 g of water at 373 K when it changes from liquid phase to vapour phase at the same temperature is:                   [JEE ONLINE 22-04-2013]
  A)
         2256   
  done clear
  B)
       167
  done clear
  C)
   2089   
  done clear
  D)
                   1  
  done clear
  View Answer play_arrow

• question_answer37) 

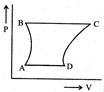
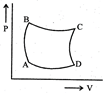
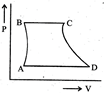
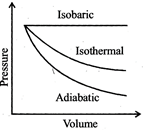
  A sample of gas expands from \[{{\operatorname{V}}_{1}}\] to \[{{\operatorname{V}}_{2}}\]. In which of the following, the work dine will be greatest?

  [JEE ONLINE 25-04-2013]

  A)
          Same in all processes
  done clear
  B)
        Isobaric process
  done clear
  C)
  Isothermal process
  done clear
  D)
     A diabetic process  
  done clear
  View Answer play_arrow

• question_answer38) In the isothermal expansion of 10g of gas from volume V to 2V the work done by the gas is 575J. What is the root mean square speed of the molecules of the gas at that temperature?                                 [JEE ONLINE 25-04-2013]
  A)
         398 m/s                       
  done clear
  B)
  520 m/s
  done clear
  C)
  499 m/s
  done clear
  D)
                    532 m/s
  done clear
  View Answer play_arrow

• question_answer39) 

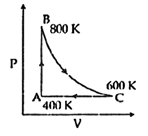
  One mole of diatomic ideal gas undergoes a cyclic process ABC as shown in figure. The process BC is adiabatic. The temperatures at A, B and C are 400 K, 800 K and 600 K respectively. Choose the correct statement:   [JEE MAIN 2014]

  A)
   The change in internal energy in the process AB is − 350 R.
  done clear
  B)
   The change in internal energy in the process BC is − 500 R.
  done clear
  C)
   The change in internal energy in whole cyclic process is 250 R.
  done clear
  D)
   The change in internal energy in the process CA is 700 R.
  done clear
  View Answer play_arrow

• question_answer40) The equation of state for a gas is given by PV = nRT + aV, where n is the number of moles and a is a positive constant. The initial temperature and pressure of one mole of the gas contained in a cylinder are \[{{T}_{o}}\]and \[{{P}_{o}}\]respectively. The work done by the gas when its temperature doubles isobarically will be:                            [JEE ONLINE 09-04-2014]
  A)
  \[\frac{{{P}_{o}}{{T}_{o}}R}{{{P}_{o}}-\alpha }\]     
  done clear
  B)
       \[\frac{{{P}_{o}}{{T}_{o}}R}{{{P}_{o}}+\alpha }\]
  done clear
  C)
  \[{{P}_{o}}{{T}_{o}}R\ln 2\]   
  done clear
  D)
       \[{{P}_{o}}{{T}_{o}}R\]
  done clear
  View Answer play_arrow

• question_answer41) During an adiabatic compression, 830 J of work is done on 2 moles of a diatomic ideal gas to reduce its volume by 50%. The change in its temperature is nearly: \[(R=8.3J{{K}^{-1}}mo{{l}^{-1}})\]                                [JEE ONLINE 11-04-2014]
  A)
   40 K    
  done clear
  B)
        33 K
  done clear
  C)
   20 K    
  done clear
  D)
                    14 K
  done clear
  View Answer play_arrow

• question_answer42) 

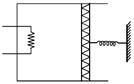
  An ideal monoatomic gas is confined in a cylinder by a spring loaded piston of cross section \[8.0\times {{10}^{-3}}{{m}^{2}}.\] Initially the gas is at 300 K and occupies a volume of \[2.4\times {{10}^{-3}}{{m}^{3}}\] and the spring is in its relaxed state as shown in figure. The gas is heated by a small heater until the piston moves out slowly by 0.1 m. The force constant of the spring is 8000 N/m and the atmospheric pressure is \[1.0\times {{10}^{5}}N/{{m}^{2}}.\] The cylinder and the piston are thermally insulated. The piston and the spring are massless and there is no friction between the piston and the cylinder. The final temperature of the gas will be:

  (Neglect the heat loss through the lead wires of the heater. The heat capacity of the heater coil is also negligible).

  [JEE ONLINE 11-04-2014]

  A)
   300 K  
  done clear
  B)
        800 K
  done clear
  C)
   500 K  
  done clear
  D)
        1000 K
  done clear
  View Answer play_arrow

• question_answer43) A Carnot engine absorbs 1000 J of heat energy from a reservoir at 127°C and rejects 600 J of heat energy during each cycle. The efficiency of engine and temperature of sink will be:                         [JEE ONLINE 12-04-2014]
  A)
   20% and – 43°C
  done clear
  B)
        40% and – 33°C
  done clear
  C)
   50% and – 20°C
  done clear
  D)
        70% and – 10°C
  done clear
  View Answer play_arrow

• question_answer44) A gas is compressed from a volume of \[2{{m}^{3}}\] to a volume of \[1{{m}^{3}}\] at a constant pressure of \[100N/{{m}^{2}}.\] Then it is heated at constant volume by supplying 150 J of energy. As a result, the internal energy of the gas:   [JEE ONLINE 19-04-2014]
  A)
   increases by 250 J
  done clear
  B)
        decreases by 250 J
  done clear
  C)
   increases by 50 J
  done clear
  D)
        decreases by 50 J
  done clear
  View Answer play_arrow

• question_answer45) 

  A solid body of constant heat capacity 1J/°C is being heated by keeping it in contact with reservoirs in two ways:

  (i) Sequentially keeping in contact with 2 reservoirs such that each reservoir supplies same amount of heat.

  (ii) Sequentially keeping in contact with 8 reservoirs such that each reservoir supplies same amount of heat.

  In both the cases body is brought from initial temperature 100°C to final temperature 200°C. Entropy change of the body in the two cases respectively is:                                                                  [JEE MAIN 2015]

  A)
   \[\ln \,2,\,2\ln \,2\]         
  done clear
  B)
        \[2\ln 2,\,\,8\ln \,2\]
  done clear
  C)
   \[\ln \,2,\,4\ln \,2\]         
  done clear
  D)
        \[\ln \,2,\,\ln \,2\]
  done clear
  View Answer play_arrow

• question_answer46) 

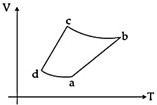
  An ideal gas goes through a reversible cycle \[a\to b\to c\to d\]has the V - T diagram shown below. Process \[d\to a\]and \[b\to c\] are adiabatic.                                                                            [JEE ONLINE 10-04-2015]

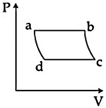
  The corresponding P - V diagram for the process is (all figures are schematic and not drawn to scale):

  A)
           
  done clear
  B)
   
  done clear
  C)
   
  done clear
  D)
        
  done clear
  View Answer play_arrow

• question_answer47) 

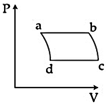
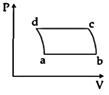
  'n' moles of an ideal gas undergoes a process \[A\to B\]as shown in the figure. The maximum temperature of the gas during the process will be:                                                             [JEE MAIN - I 3-4-2016]

  A)
  \[\frac{9{{P}_{0}}{{V}_{0}}}{nR}\]    
  done clear
  B)
       \[\frac{9{{P}_{0}}{{V}_{0}}}{4nR}\]
  done clear
  C)
  \[\frac{3{{P}_{0}}{{V}_{0}}}{2nR}\]  
  done clear
  D)
       \[\frac{9{{P}_{0}}{{V}_{0}}}{2nR}\]
  done clear
  View Answer play_arrow

• question_answer48) An ideal gas undergoes a quasi-static, reversible process in which its molar heat capacity C remains constant. If during this process the relation of pressure P and volume V is given by \[\text{P}{{\text{V}}^{\text{n}}}=\]constant, then n is given by (Here CP and CV are molar specific heat at constant pressure and constant volume, respectively):-                      [JEE MAIN - I 3-4-2016]
  A)
  \[n=\frac{C-{{C}_{v}}}{C-{{C}_{p}}}\]          
  done clear
  B)
       \[n=\frac{{{C}_{p}}}{{{C}_{v}}}\]
  done clear
  C)
  \[n=\frac{C-{{C}_{p}}}{C-{{C}_{v}}}\]          
  done clear
  D)
       \[n=\frac{{{C}_{p}}-C}{C-{{C}_{v}}}\]
  done clear
  View Answer play_arrow

• question_answer49) The ratio of work done by an ideal monatomic gas to the heat supplied to it in an isobaric process is:     [JEE ONLINE 09-04-2016]
  A)
  \[\frac{3}{2}\]              
  done clear
  B)
       \[\frac{2}{3}\]
  done clear
  C)
  \[\frac{3}{5}\]              
  done clear
  D)
       \[\frac{2}{5}\]
  done clear
  View Answer play_arrow

• question_answer50)             A carnot freezer takes heat from water at \[^{o}C\] inside it and rejects it to the room at a temperature of \[{{27}^{o}}C\]. The latent heat of ice is\[336\times {{10}^{3}}J\,k{{g}^{-1}}\]. If 5 kg of water at \[{{0}^{o}}C\]is converted into ice at \[{{0}^{o}}C\]by the freezer, then the energy consumed by the freezer is close to:                                                                                         [JEE ONLINE 10-04-2016]
  A)
   \[1.68\times {{10}^{6}}J\]      
  done clear
  B)
        \[1.71\times {{10}^{7}}J\]
  done clear
  C)
   \[1.51\times {{10}^{5}}J\]      
  done clear
  D)
        1\[1.67\times {{10}^{5}}J\]
  done clear
  View Answer play_arrow

• question_answer51) An engine operates by taking n moles of an ideal gas through the cycle ABCDA shown in figure. The thermal efficiency of the engine is - (Take \[{{C}_{v}}=1.4R,\]where R is gas constant)                                             [JEE Online 08-04-2017]
  A)
   0.24    
  done clear
  B)
        0.15

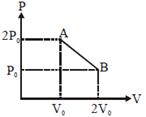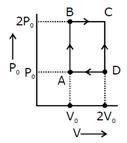
  done clear C)  0.32                 done clear D)       0.08 done clear View Answer play_arrow question_answer52) N moles of a diaotmic gas in a cylinder are at a temperature T. Heat is supplied to the cylinder such that the temperature remains constant but n moles of the diatomic gas get converted into monoatomic gas. What is the change in the total kinetic energy of the gas?                                                                                     [JEE Online 09-04-2017] A)  0                     done clear B)       \[\frac{5}{2}nRT\] done clear C)  \[\frac{3}{2}\,nRT\]       done clear D)       \[\frac{1}{2}\,nRT\] done clear View Answer play_arrow question_answer53) Two moles of an ideal monoatomic gas occupies a volume \[V\] at \[27{}^\circ C\]. The gas expands adiabatically to a volume\[\text{2 V}\]. Calculate  the final temperature of the gas and  change in its internal energy.                   [JEE Main Online 08-04-2018] A)  \[\text{(A) 189 K}\text{(B) -2}\text{.7 kj}\] done clear B)  \[\text{(A) 195 K}\text{(B) 2}\text{.7 kj}\] done clear C)  \[\text{(A) 189 K}\text{(B) 2}\text{.7 kj}\] done clear D)  \[\text{(A) 195 K}\text{(B) -2}\text{.7 kj}\] done clear View Answer play_arrow question_answer54) A Carnot's engine works as a refrigerator between \[250K\] and \[300K\]. It receives \[500cal\] heat from the reservoir at the lower temperature. The amount of work done in each cycle to operate the refrigerator is:    [JEE Online 15-04-2018] A)  \[420J\] done clear B)  \[2100J\] done clear C)  \[772J\] done clear D)       \[2520J\] done clear View Answer play_arrow question_answer55) One mode of an ideal monoatomic gas is compressed isothermally in a rigid vessel to double its pressure at room temperature, \[27{}^\circ C\]. The work done on the gas will be:                                    [JEE Online 15-04-2018] A)  \[300R\,\,In\,\,6\]           done clear B)       \[300R\] done clear C)  \[300R\,\,In\,\,7\]           done clear D)       \[300R\,\,In\,\,2\] done clear View Answer play_arrow question_answer56) Two Carnot engines A and B are operated in series. Engine A receives heat from a reservoir at \[600K\] and rejects heat to a reservoir heat to reservoir at temperature\[T\]. Engine\[B\] receives heat rejected by engine \[A\] and in turn rejects it to a reservoir at\[100K\]. If the efficiencies of the two engines A and B are represented by \[{{\eta }_{A}}\] and \[{{\eta }_{B}}\] respectively, then what is the value of \[\frac{{{\eta }_{A}}}{{{\eta }_{B}}}\] [JEE Online 15-04-2018 (II)] A)  \[\frac{12}{7}\]             done clear B)       \[\frac{12}{5}\] done clear C)  \[\frac{5}{12}\]             done clear D)       \[\frac{7}{12}\] done clear View Answer play_arrow 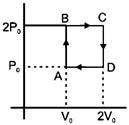question_answer57)  One mole of an ideal monoatomic gas is taken along the path ABCA as shown in the PV diagram. The maximum temperature attained by the gas along the path BC is given by                           [JEE Main Online 16-4-2018] A)  \[\frac{25}{8}\frac{{{P}_{0}}{{V}_{0}}}{R}\] done clear B)      \[\frac{25}{4}\frac{{{P}_{0}}{{V}_{0}}}{R}\] done clear C) \[\frac{25}{16}\frac{{{P}_{0}}{{V}_{0}}}{R}\] done clear D)       \[\frac{5}{8}\frac{{{P}_{0}}{{V}_{0}}}{R}\]  done clear View Answer play_arrow question_answer58)  A gas can be taken from A to B via two different processes ACB and ADB.                                                                                                             [JEE Main 09-Jan-2019 Morning]             When path ACB is used 60 J of heat flows into the system and 30 J of work is done by the system. If path ADB is used work done by the system is 10 J. The heat Flow into the system in path ADB is: A)        40 J     done clear B)  20 J done clear C)  100 J               done clear D)       80 J done clear View Answer play_arrow question_answer59) Two Carnot engines A and B are operated in series. The first one, A, receives heat at \[{{T}_{1}}\left( =600\text{ }K \right)\] and rejects to a reservoir at temperature Ta. The second engine B receives heat rejected by the first engine and, in turn, rejects to a heat reservoir at\[{{T}_{3}}(=400\text{ }K)\]. 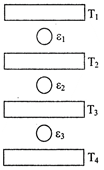Calculate the temperature \[{{T}_{2}}\] if the work outputs of the engines are equal: [JEE Main 09-Jan-2019 Evening] A)  500 K           done clear B)  300 K done clear C)  600 K               done clear D)       400 K done clear View Answer play_arrow question_answer60) Three Carnot engines operate in series between a heat source at a temperature \[{{T}_{1}}\] and a heat sink at temperature \[{{T}_{4}}\] (see figure). There are two other reservoirs at temperature\[{{T}_{2}}and\text{ }{{T}_{3}}\], as shown, with\[{{T}_{1}}>{{T}_{2}}>{{T}_{3}}>{{T}_{4}}\]. The three engines are equally efficient if -                                                                           [JEE Main 10-Jan-2019 Morning] A) \[{{T}_{2}}=\,\,{{({{T}_{1}}^{3}{{T}_{4}})}^{1/4}};\,\,=\,\,{{T}_{3}}\,\,=\,\,{{({{T}_{1}}{{T}_{4}}^{3})}^{1/4}}\] done clear B)    \[{{T}_{2}}=\,\,{{({{T}_{1}}{{T}_{4}})}^{1/2}};\,\,\,{{T}_{3}}\,\,=\,\,{{({{T}_{1}}^{2}{{T}_{4}})}^{1/3}}\] done clear C)    \[{{T}_{2}}=\,\,{{({{T}_{1}}{{T}_{4}}^{2})}^{1/3}};\,\,{{T}_{3}}\,\,=\,\,{{({{T}_{1}}^{2}{{T}_{4}})}^{1/3}}\] done clear D)    \[{{T}_{2}}=\,\,{{({{T}_{1}}^{2}{{T}_{4}})}^{1/3}};\,\,\,\,{{T}_{3}}\,\,=\,\,{{({{T}_{1}}{{T}_{4}}^{2})}^{1/3}}\] done clear View Answer play_arrow question_answer61) Half mole of an ideal monoatomic gas is heated at constant pressure of 1 atm from\[20{}^\circ C\text{ }to\text{ }90{}^\circ C\]. Work done by gas is close to - (Gas constant \[R=8.31\text{ }J\] /mol. K)                        [JEE Main 10-Jan-2019 Evening] A)  581 J               done clear B)  73 J   done clear C)  146 J   done clear D)       291 J done clear View Answer play_arrow question_answer62) A rigid diatomic ideal gas undergoes an adiabatic process at room temperature. The relation between temperature and volume for this process is \[T{{V}^{x}}=\]constant, then x is- [JEE Main 11-Jan-2019 Morning] A) \[\frac{2}{3}\]               done clear B)      \[\frac{3}{5}\] done clear C) \[\frac{2}{5}\]   done clear D)      \[\frac{5}{3}\] done clear View Answer play_arrow question_answer63) A gas mixture consists of 3 moles of oxygen and 5 moles of argon at temperature T. Considering only translational and rotational modes, the total internal energy of the system is-                 [JEE Main 11-Jan-2019 Morning] A)  20 RT   done clear B)       12 RT done clear C)  4 RT    done clear D)       15 RT done clear View Answer play_arrow question_answer64)  For the given cyclic process CAB as shown for a gas, the work done is-          [JEE Main 12-Jan-2019 Morning]        A)  10 J     done clear B)       1 J done clear C)  5 J                   done clear D)       30 J done clear View Answer play_arrow question_answer65) A vertical closed cylinder is separated into two parts by a frictionless piston of mass m and negligible thickness. The piston is free to move along the length of the cylinder. The length of the cylinder above the piston is\[{{l}_{1}}\]and that below the piston is\[{{l}_{2}},\]such that \[{{l}_{1}}>{{l}_{2}}.\]Each part of the cylinder contains n moles of an ideal gas at equal temperature T. If the piston is stationary, its mass m, will be given by (R is universal gas constant and g is the acceleration due to gravity) [JEE Main 12-Jan-2019 Evening] A) \[\frac{RT}{ng}\left[ \frac{{{l}_{1}}-3{{l}_{2}}}{{{l}_{1}}{{l}_{2}}} \right]\]    done clear B)      \[\frac{nRT}{g}\left[ \frac{1}{{{l}_{2}}}+\frac{1}{{{l}_{1}}} \right]\] done clear C) \[\frac{RT}{g}\left[ \frac{2{{l}_{1}}+{{l}_{2}}}{{{l}_{1}}{{l}_{2}}} \right]\]    done clear D)      \[\frac{nRT}{g}\left[ \frac{{{l}_{1}}-{{l}_{2}}}{{{l}_{1}}{{l}_{2}}} \right]\] done clear View Answer play_arrow question_answer66)  A parallel plate capacitor with plates of area 1 m2 each, are at a separation of 0.1m. If the electric field between the plates is \[100\,\,N\,\,{{C}^{-1}},\]the magnitude of charge on each plate is-                       [JEE Main 12-Jan-2019 Evening]  \[\left( \text{Take}\,{{\varepsilon }_{0}}=8.85\times {{10}^{-12}}\frac{{{C}^{2}}}{N{{m}^{2}}} \right)\] A)       \[8.85\times {{10}^{-10}}C\]     done clear B) \[7.85\times {{10}^{-10}}C\] done clear C) \[9.85\times {{10}^{-10}}C\] done clear D)      \[6.85\times {{10}^{-10}}C\] done clear View Answer play_arrow question_answer67) A thermally insulated vessel contains 150g of water at \[0{}^\circ C\]. Then the air from the vessel is pumped out adiabatically. A fraction of water turns into ice and the rest evaporates at \[0{}^\circ C\] itself. The mass of evaporated water will be closest to: (Latent heat of vaporization of water \[=2.10\times {{10}^{6}}Jk{{g}^{1}}\] and Latent heat of Fusion of water \[=3.36\times {{10}^{5}}Jk{{g}^{1}}\])                                                                                                                         [JEE Main 8-4-2019 Morning] A)  130 g done clear B)                   35 g done clear C)  20 g     done clear D)       150 g done clear View Answer play_arrow question_answer68)  The given diagram shows four processes i.e., isochoric, isobaric, isothermal and adiabatic. The correct assignment of the processes, in the same order is given by:- [JEE Main 8-4-2019 Afternoon] A)  d a c b done clear B)       a d c b done clear C)  a d b c done clear D)       d a b c done clear View Answer play_arrow question_answer69)  Following figure shows two processes A and B for a gas. If \[\Delta {{Q}_{A}}\]and \[\Delta {{Q}_{B}}\]are the amount of heat absorbed by the system in two cases, and \[\Delta {{U}_{A}}\]and \[\Delta {{U}_{B}}\]are changes in internal energies, respectively, then :          [JEE Main 9-4-2019 Morning] A) \[\Delta {{Q}_{A}}=\Delta {{Q}_{B}};\Delta {{U}_{A}}=\Delta {{U}_{B}}\] done clear B) \[\Delta {{Q}_{A}}>\Delta {{Q}_{B}};\Delta {{U}_{A}}=\Delta {{U}_{B}}\] done clear C) \[\Delta {{Q}_{A}}>\Delta {{Q}_{B}};\Delta {{U}_{A}}>\Delta {{U}_{B}}\] done clear D) \[\Delta {{Q}_{A}}<\Delta {{Q}_{B}};\Delta {{U}_{A}}<\Delta {{U}_{B}}\] done clear View Answer play_arrow question_answer70) n moles of an ideal gas with constant volume heat capcity \[{{C}_{V}}\]undergo an isobaric expansion by certain volume. The ratio of the work done in the process, to the heat supplied is:                         [JEE Main 10-4-2019 Morning] A) \[\frac{4nR}{{{C}_{V}}-nR}\]                done clear B) \[\frac{nR}{{{C}_{V}}-nR}\] done clear C) \[\frac{nR}{{{C}_{V}}+nR}\]    done clear D)      \[\frac{4nR}{{{C}_{V}}+nR}\] done clear View Answer play_arrow question_answer71) When heat Q is supplied to a diatomic gas of rigid molecules, at constant volume its temperature increases by \[\Delta T.\]The heat required to produce the same change in temperature, at a constant pressure is : [JEE Main 10-4-2019 Afternoon] A) \[\frac{7}{5}Q\] done clear B)      \[\frac{3}{2}Q\] done clear C) \[\frac{5}{3}Q\]             done clear D)      \[\frac{2}{3}Q\] done clear View Answer play_arrow question_answer72) One mole of an ideal gas passes through a process where pressure and volume obey the relation \[P={{P}_{0}}\left[ 1-\frac{1}{2}{{\left( \frac{{{V}_{0}}}{V} \right)}^{2}} \right].\] Here \[{{P}_{0}}\]and \[{{V}_{0}}\] are constants. Calculate the change in the temperature of the gas if its volume changes from \[{{V}_{0}}\] to \[2{{V}_{0}}\]. [JEE Main 10-4-2019 Afternoon] A) \[\frac{1}{2}\frac{{{P}_{0}}{{V}_{0}}}{R}\]    done clear B)      \[\frac{3}{4}\frac{{{P}_{0}}{{V}_{0}}}{R}\] done clear C) \[\frac{5}{4}\frac{{{P}_{0}}{{V}_{0}}}{R}\]    done clear D)      \[\frac{1}{4}\frac{{{P}_{0}}{{V}_{0}}}{R}\] done clear View Answer play_arrow question_answer73)  A sample of an ideal gas is taken through the cyclic process abca as shown in the figure. The change in the internal energy of the gas along the path ca is –180J. The gas absorbs 250 J of heat along the path ab and 60 J along the path bc. The work done by the gas along the path abc is :                                                                      [JEE Main Held on 12-4-2019 Morning] A)  100 J done clear B)                   120 J done clear C)  140 J   done clear D)       130 J done clear View Answer play_arrow question_answer74) A Carnot engine has an efficiency of 1/6. When the temperature of the sink is reduced by \[62{}^\text{o}C\], its efficiency is doubled. The temperatures of the source and the sink are, respectively                       [JEE Main 12-4-2019 Afternoon] A)  \[124{}^\text{o}C,62{}^\text{o}C\] done clear B)       \[37{}^\text{o}C,99{}^\text{o}C\] done clear C)  \[62{}^\text{o}C,124{}^\text{o}C\] done clear D)       \[99{}^\text{o}C,37{}^\text{o}C\] done clear View Answer play_arrow question_answer75) A diatomic gas with rigid molecules does 10 J of work when expanded at constant pressure. What would be the heat energy absorbed by the gas, in this process?                                  [JEE Main 12-4-2019 Afternoon] A)  35 J done clear B)      40 J done clear C)  25 J                 done clear D)       30 J done clear View Answer play_arrow question_answer76) A litre of dry air at STP expands adiabatically to a volume of 3 litres. If \[\gamma =1.40\], the work done by air is \[\left( {{3}^{1.4}}=4.6555 \right)\] [Take air to be an ideal gas]                                                 [JEE MAIN Held on 07-01-2020 Morning] A)  90.5 J  done clear B)       60.7 J done clear C)  48 J                 done clear D)       100.8 J done clear View Answer play_arrow question_answer77) A Carnot engine operates between two reservoirs of temperatures 900 K and 300 K. The engine performs 1200 J of work per cycle. The heat energy (in J) delivered by the engine to the low temperature reservoir, in a cycle, is_______. [JEE MAIN Held on 07-01-2020 Morning] View Answer play_arrow question_answer78) Two ideal Carnot engines operate in cascade (all heat given up by one engine is used by the other engine to produce work) between temperatures, \[{{T}_{1}}\]and \[{{T}_{2}}\]. The temperature of the hot reservoir of the first engine is \[{{T}_{1}}\] and the temperature of the cold reservoir of the second engine is\[{{T}_{2}}\]. \[T\] is temperature of the sink of first engine which is also the source for the second engine. How is \[T\] related to \[{{T}_{1}}\] and\[{{T}_{2}}\], if both the engines perform equal amount of work? [JEE MAIN Held on 07-01-2020 Evening] A)  \[T=\frac{{{T}_{1}}+{{T}_{2}}}{2}\] done clear B)       \[T=\sqrt{{{T}_{1}}{{T}_{2}}}\] done clear C)  \[T=\frac{2{{T}_{1}}{{T}_{2}}}{{{T}_{1}}+{{T}_{2}}}\]     done clear D)       \[T=0\] done clear View Answer play_arrow question_answer79) Under an adiabatic process, the volume of an ideal gas gets doubled. Consequently the mean collision time between the gas molecule changes from \[{{\tau }_{1}}\] to\[{{\tau }_{2}}\]. If \[\frac{{{C}_{P}}}{{{C}_{v}}}=\gamma \]for this gas then a good estimate for \[\frac{{{\tau }_{2}}}{{{\tau }_{1}}}\]is given by [JEE MAIN Held on 07-01-2020 Evening] A)  \[{{\left( \frac{1}{2} \right)}^{\frac{\gamma +1}{2}}}\] done clear B)       \[{{\left( \frac{1}{2} \right)}^{\gamma }}\] done clear C)  \[2\]                 done clear D)       None of these done clear View Answer play_arrow 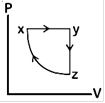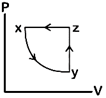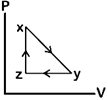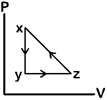question_answer80)  A thermodynamic cycle xyzx is shown on a V-T diagram The P-V diagram that best describes this cycle Is: (Diagrams are schematic and not to scale)                                                                                        [JEE MAIN Held On 08-01-2020 Morning] A)   done clear B)        done clear C)   done clear D)        done clear View Answer play_arrow question_answer81) A Carnot engine having an efficiency of \[\frac{1}{10}\] is being used as a refrigerator. 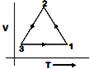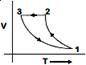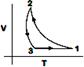If the work done on the refrigerator is 10 J, the amount of heat absorbed from the reservoir at lower temperature is :   [JEE MAIN Held on 08-01-2020 Evening] A)  90 J     done clear B)       1 J done clear C)  99 J                 done clear D)       100 J done clear View Answer play_arrow question_answer82)  Which of the following is an equivalent cyclic process corresponding to the thermodynamic cyclic given in the figure? where, \[1\to 2\] is adiabatic. (Graphs are schematic and are not to scale)                                                                      [JEE MAIN Held on 09-01-2020 Morning] A)   done clear B)        done clear C)   done clear D)        done clear View Answer play_arrow question_answer83) Starting at temperature \[300\text{ }K,\]one mole of an ideal diatomic gas \[(\gamma =1.4)\] is first compressed adiabatically from volume \[{{V}_{1}}\] to \[{{V}_{2}}=\frac{{{V}_{1}}}{16}.\]It is then allowed to expand isobarically to volume \[2{{V}_{2}}\]. If all the processes are the quasi-static then the final temperature of the gas (in \[{}^\circ K\]) is (to the nearest integer) _______. [JEE MAIN Held on 09-01-2020 Evening] View Answer play_arrow Study Package JEE PYQ-Thermodynamical Processes   Buy Now     Please Wait you are being redirected.... You need to login to perform this action. You will be redirected in 3 sec × Reset Password. Email : Send Password Cancel Add Mobile Enter Mobile Number Submit Logout Enter OTP OTP has been sent to your mobile number and is valid for one hour Enter One Time Password Submit Change Mobile: Submit Logout Mobile Number Verified Your mobile number is verified. Close Free Videos × For free video lectures fill in the form below and we will get back to you. * Email Address: * Mobile Number: Submit Cancel