(1)
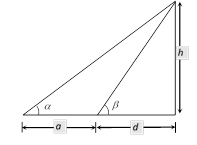 \[a=h\,(\cot \alpha -\cot \beta )=\frac{h\sin (\beta -\alpha )}{\sin \alpha .\sin \beta }\] \[\therefore \,h=a\sin \alpha \sin \beta \,co\text{sec }(\beta -\alpha )\] and \[d=h\cot \beta =a\sin \alpha .\cos \beta .\text{cosec}(\beta -\alpha )\]
\[a=h\,(\cot \alpha -\cot \beta )=\frac{h\sin (\beta -\alpha )}{\sin \alpha .\sin \beta }\] \[\therefore \,h=a\sin \alpha \sin \beta \,co\text{sec }(\beta -\alpha )\] and \[d=h\cot \beta =a\sin \alpha .\cos \beta .\text{cosec}(\beta -\alpha )\]
|
(2)
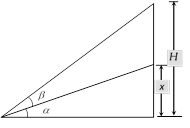 \[H=x\cot \alpha \tan (\alpha +\beta )\]
\[H=x\cot \alpha \tan (\alpha +\beta )\]
|
(3)
 \[a=h(\cot \alpha +\cot \beta ),\] where by \[h=a\sin \alpha .\sin \beta .\text{cosec}(\alpha +\beta )\] and \[d=h\cot \beta =a\sin \alpha .\cos \beta .\text{cosec }(\alpha +\beta )\]
\[a=h(\cot \alpha +\cot \beta ),\] where by \[h=a\sin \alpha .\sin \beta .\text{cosec}(\alpha +\beta )\] and \[d=h\cot \beta =a\sin \alpha .\cos \beta .\text{cosec }(\alpha +\beta )\]
|
||||||||||||
(4)
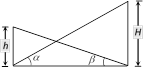 \[H=\frac{h\cot \beta }{\cot \alpha }\]
\[H=\frac{h\cot \beta }{\cot \alpha }\]
|
(5)
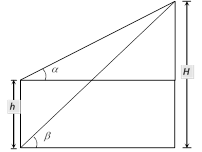 \[h=\frac{H\sin (\beta -\alpha )}{\cos \alpha \sin \beta }\] or \[H=\frac{h\cot \alpha }{\cot \alpha -\cot \beta }\]
\[h=\frac{H\sin (\beta -\alpha )}{\cos \alpha \sin \beta }\] or \[H=\frac{h\cot \alpha }{\cot \alpha -\cot \beta }\]
|
(6)
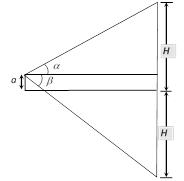 \[H=\frac{a\sin (\alpha +\beta )}{\sin (\beta -\alpha )}\]
\[H=\frac{a\sin (\alpha +\beta )}{\sin (\beta -\alpha )}\]
|
||||||||||||
(7)
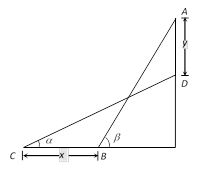 \[AB=CD\]. Then, \[x=y\tan \left( \frac{\alpha +\beta }{2} \right)\]
\[AB=CD\]. Then, \[x=y\tan \left( \frac{\alpha +\beta }{2} \right)\]
|
(8)
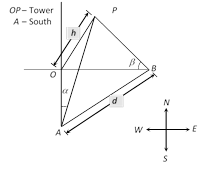 \[h=\frac{d}{\sqrt{{{\cot }^{2}}\beta +{{\cot }^{2}}\alpha }}\] \[h=\frac{d}{\sqrt{{{\cot }^{2}}\beta +{{\cot }^{2}}\alpha }}\]
|
(9)
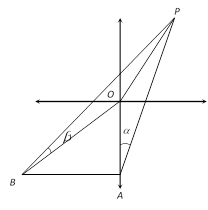 \[h=\frac{AB}{\sqrt{{{\cot }^{2}}\beta -{{\cot }^{2}}\alpha }}\]
\[h=\frac{AB}{\sqrt{{{\cot }^{2}}\beta -{{\cot }^{2}}\alpha }}\]
|
||||||||||||
(10)
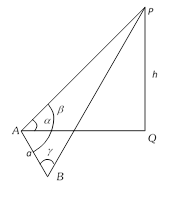 \[h=AP\sin \alpha \] \[=a\sin \alpha .\sin \gamma .c\text{osec}(\beta -\gamma )\] and If \[AQ=d\], then \[d=AP\text{ }\cos \alpha =a\text{ }\cos \alpha .\sin \gamma \text{ }.\text{ cosec }(\beta -\gamma )\]
\[h=AP\sin \alpha \] \[=a\sin \alpha .\sin \gamma .c\text{osec}(\beta -\gamma )\] and If \[AQ=d\], then \[d=AP\text{ }\cos \alpha =a\text{ }\cos \alpha .\sin \gamma \text{ }.\text{ cosec }(\beta -\gamma )\]
|
more...
(1) Angles in the same segment of a circle are equal i.e., \[\angle APB=\angle AQB=\angle ARB\].
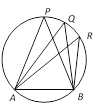 (2) Angles in the alternate segments of a circle are equal.
(2) Angles in the alternate segments of a circle are equal.


 (3) If the line joining two points A and B subtends the greatest angle \[\alpha \] at a point P then the circle, will touch the straight line \[XX'\]at the point P.
(3) If the line joining two points A and B subtends the greatest angle \[\alpha \] at a point P then the circle, will touch the straight line \[XX'\]at the point P.
 (4) The angle subtended by any chord at the centre is twice the angle subtended by the same on any point on the circumference of the circle.
(4) The angle subtended by any chord at the centre is twice the angle subtended by the same on any point on the circumference of the circle.

(1) Angle of elevation and depression: Let O and P be two points such that P is at higher level than O. Let PQ, OX be horizontal lines through P and O, respectively. If an observer (or eye) is at O and the object is at P, then \[\angle XOP\] is called the angle of elevation of P as seen from O. This angle is also called the angular height of P from O.
 If an observer (or eye) is at P and the object is at O, then \[\angle QPO\] is called the angle of depression of O as seen from P.
(2) Method of solving a problem of height and distance
(i) Draw the figure neatly showing all angles and distances as far as possible.
(ii) Always remember that if a line is perpendicular to a plane then it is perpendicular to every line in that plane.
(iii) In the problems of heights and distances we come across a right angled triangle in which one (acute) angle and a side is given. Then to find the remaining sides, use trigonometrical ratios in which known (given) side is used, i.e., use the formula.
(iv) In any triangle other than right angled triangle, we can use 'the sine rule'.
i.e., formula, \[\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C},\] or cosine formula i.e., \[\cos A=\frac{{{b}^{2}}+{{c}^{2}}-{{a}^{2}}}{2bc}\] etc.
(v) Find the length of a particular side from two different triangles containing that side common and then equate the two values thus obtained.
(3) Geometrical properties and formulae for a triangle
(i) In a triangle the internal bisector of an angle divides the opposite side in the ratio of the arms of the angle. \[\frac{BD}{DC}=\frac{c}{b}\].
If an observer (or eye) is at P and the object is at O, then \[\angle QPO\] is called the angle of depression of O as seen from P.
(2) Method of solving a problem of height and distance
(i) Draw the figure neatly showing all angles and distances as far as possible.
(ii) Always remember that if a line is perpendicular to a plane then it is perpendicular to every line in that plane.
(iii) In the problems of heights and distances we come across a right angled triangle in which one (acute) angle and a side is given. Then to find the remaining sides, use trigonometrical ratios in which known (given) side is used, i.e., use the formula.
(iv) In any triangle other than right angled triangle, we can use 'the sine rule'.
i.e., formula, \[\frac{a}{\sin A}=\frac{b}{\sin B}=\frac{c}{\sin C},\] or cosine formula i.e., \[\cos A=\frac{{{b}^{2}}+{{c}^{2}}-{{a}^{2}}}{2bc}\] etc.
(v) Find the length of a particular side from two different triangles containing that side common and then equate the two values thus obtained.
(3) Geometrical properties and formulae for a triangle
(i) In a triangle the internal bisector of an angle divides the opposite side in the ratio of the arms of the angle. \[\frac{BD}{DC}=\frac{c}{b}\].
 (ii) In an isosceles triangle the median is perpendicular to the base i.e., \[AD\,\bot \,BC\].
(iii) In similar triangles the corresponding sides are proportional.
(iv) The exterior angle is equal to sum of interior opposite angles.
(4) North-east : North-east means equally inclined to north and east, south-east means equally inclined to south and east. ENE means equally inclined to east and north-east.
(ii) In an isosceles triangle the median is perpendicular to the base i.e., \[AD\,\bot \,BC\].
(iii) In similar triangles the corresponding sides are proportional.
(iv) The exterior angle is equal to sum of interior opposite angles.
(4) North-east : North-east means equally inclined to north and east, south-east means equally inclined to south and east. ENE means equally inclined to east and north-east.
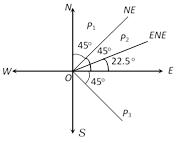 (5) Bearing : In the figure, if the observer and the object i.e., O and P be on the same level then bearing is defined. To measure the ?Bearing?, the four standard directions East, West, North and South are taken as the cardinal directions.
(5) Bearing : In the figure, if the observer and the object i.e., O and P be on the same level then bearing is defined. To measure the ?Bearing?, the four standard directions East, West, North and South are taken as the cardinal directions.
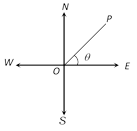 Angle between the line of observation i.e., OP and any one standard direction– east, west, more...
Angle between the line of observation i.e., OP and any one standard direction– east, west, more...
Different formulae will be used in different cases and sometimes the same problem may be solved in different ways by different formulae. We should, therefore, look for that formula which will suit the problem best.
(1) Solution of a right angled triangle
(2) Solution of a triangle in general
(1) Solution of a right angled triangle
(i) When two sides are given: Let the triangle be right angled at C. Then we can determine the remaining elements as given in the following table.
A regular polygon is a polygon which has all its sides equal and all its angles equal.
(1) Each interior angle of a regular polygon of n sides is \[\left( \frac{2n-4}{n} \right)\times \]right angles \[=\left[ \frac{2n-4}{n} \right]\times \frac{\pi }{2}\] radians.
(2) The circle passing through all the vertices of a regular polygon is called its circumscribed circle.
If \[a\] is the length of each side of a regular polygon of \[n\] sides, then the radius \[R\] of the circumscribed circle, is given by \[R=\frac{a}{2}\,.\,\text{cosec }\left( \frac{\pi }{n} \right)\]
(3) The circle which can be inscribed within the regular polygon so as to touch all its sides is called its inscribed circle.
Again if \[a\] is the length of each side of a regular polygon of \[n\] sides, then the radius \[r\] of the inscribed circle is given by \[r=\frac{a}{2}\,.\,\text{cot }\left( \frac{\pi }{n} \right)\]
 (4) The area of a regular polygon is given by \[\Delta =n\,\,\times \] area of triangle \[OAB\]
\[=\frac{1}{4}n{{a}^{2}}\cot \,\left( \frac{\pi }{n} \right),\] (in terms of side)
\[=n{{r}^{2}}\,.\,\tan \,\left( \frac{\pi }{n} \right),\] (in terms of in-radius)
\[=\frac{n}{2}\,.\,{{R}^{2}}\sin \,\left( \frac{2\pi }{n} \right),\] (in terms of circum-radius)
(4) The area of a regular polygon is given by \[\Delta =n\,\,\times \] area of triangle \[OAB\]
\[=\frac{1}{4}n{{a}^{2}}\cot \,\left( \frac{\pi }{n} \right),\] (in terms of side)
\[=n{{r}^{2}}\,.\,\tan \,\left( \frac{\pi }{n} \right),\] (in terms of in-radius)
\[=\frac{n}{2}\,.\,{{R}^{2}}\sin \,\left( \frac{2\pi }{n} \right),\] (in terms of circum-radius)
A quadrilateral PQRS is said to be cyclic quadrilateral if there exists a circle passing through all its four vertices P, Q, R and S.
 Let a cyclic quadrilateral be such that
\[PQ=a,\,QR=b,\,RS=c\] and \[SP=d\].
Then\[\angle Q+\angle S={{180}^{o}}\],\[\angle A+\angle C={{180}^{o}}\]
Let \[2s=a+b+c+d\]
Area of cyclic quadrilateral = \[\frac{1}{2}(ab+cd)\sin Q\]
Also, area of cyclic quadrilateral = \[\sqrt{(s-a)(s-b)(s-c)(s-d)}\], where \[2s=a+b+c+d\] and \[\cos Q=\frac{{{a}^{2}}+{{b}^{2}}-{{c}^{2}}-{{d}^{2}}}{2(ab+cd)}\].
(1) Circumradius of cyclic quadrilateral : Circum circle of quadrilateral PQRS is also the circumcircle of \[\Delta PQR\]. \[R=\frac{1}{4\Delta }\sqrt{(ac+bd)(ad+bc)(ab+cd)}=\frac{1}{4}\sqrt{\frac{(ac+bd)(ad+bc)(ab+cd)}{(s-a)(s-b)(s-c)(s-d)}}\].
(2) Ptolemy's theorem : In a cyclic quadrilateral PQRS, the product of diagonals is equal to the sum of the products of the length of the opposite sides i.e., According to Ptolemy's theorem, for a cyclic quadrilateral PQRS \[PR.QS=PQ.RS+RQ.PS.\]
Let a cyclic quadrilateral be such that
\[PQ=a,\,QR=b,\,RS=c\] and \[SP=d\].
Then\[\angle Q+\angle S={{180}^{o}}\],\[\angle A+\angle C={{180}^{o}}\]
Let \[2s=a+b+c+d\]
Area of cyclic quadrilateral = \[\frac{1}{2}(ab+cd)\sin Q\]
Also, area of cyclic quadrilateral = \[\sqrt{(s-a)(s-b)(s-c)(s-d)}\], where \[2s=a+b+c+d\] and \[\cos Q=\frac{{{a}^{2}}+{{b}^{2}}-{{c}^{2}}-{{d}^{2}}}{2(ab+cd)}\].
(1) Circumradius of cyclic quadrilateral : Circum circle of quadrilateral PQRS is also the circumcircle of \[\Delta PQR\]. \[R=\frac{1}{4\Delta }\sqrt{(ac+bd)(ad+bc)(ab+cd)}=\frac{1}{4}\sqrt{\frac{(ac+bd)(ad+bc)(ab+cd)}{(s-a)(s-b)(s-c)(s-d)}}\].
(2) Ptolemy's theorem : In a cyclic quadrilateral PQRS, the product of diagonals is equal to the sum of the products of the length of the opposite sides i.e., According to Ptolemy's theorem, for a cyclic quadrilateral PQRS \[PR.QS=PQ.RS+RQ.PS.\]

Let ABC be a triangle and I be the centre of incircle. Let \[{{I}_{1}}\], \[{{I}_{2}}\] and \[{{I}_{3}}\] be the centres of the escribed circles which are opposite to A, B, C respectively then \[{{I}_{1}}{{I}_{2}}{{I}_{3}}\] is called the Ex-central triangle of \[\Delta ABC\].
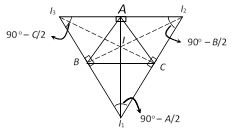 \[{{I}_{1}}{{I}_{2}}{{I}_{3}}\] is a triangle, thus the triangle ABC is the pedal triangle of its ex-central triangle \[{{I}_{1}}{{I}_{2}}{{I}_{3}}\]. The angles of ex-central triangle \[{{I}_{1}}{{I}_{2}}{{I}_{3}}\] are \[{{90}^{o}}-\frac{A}{2},\,\,{{90}^{o}}-\frac{B}{2},\,\,{{90}^{o}}-\frac{C}{2}\] and sides are \[{{I}_{1}}{{I}_{3}}=4R\cos \frac{B}{2};\,\,{{I}_{1}}{{I}_{2}}=4R\cos \frac{C}{2};\,\,{{I}_{2}}{{I}_{3}}=4R\cos \frac{A}{2}\].
Area and circum-radius of the ex-central triangle
Area of triangle
\[=\frac{1}{2}\] (Product of two sides) \[\times \] (sine of included angles)
\[\Delta =\frac{1}{2}\,\,\left( 4R\cos \frac{B}{2} \right)\,\,.\,\,\left( 4R\cos \frac{C}{2} \right)\times \sin \left( {{90}^{o}}-\frac{A}{2} \right)\]
\[\Delta =8{{R}^{2}}\cos \frac{A}{2}.\cos \frac{B}{2}.\cos \frac{C}{2}\]
Circum-radius \[=\frac{{{I}_{2}}{{I}_{3}}}{2\sin {{I}_{2}}{{I}_{1}}{{I}_{3}}}=\frac{4R\cos \frac{A}{2}}{2\sin \left( {{90}^{o}}-\frac{A}{2} \right)}=2R\].
\[{{I}_{1}}{{I}_{2}}{{I}_{3}}\] is a triangle, thus the triangle ABC is the pedal triangle of its ex-central triangle \[{{I}_{1}}{{I}_{2}}{{I}_{3}}\]. The angles of ex-central triangle \[{{I}_{1}}{{I}_{2}}{{I}_{3}}\] are \[{{90}^{o}}-\frac{A}{2},\,\,{{90}^{o}}-\frac{B}{2},\,\,{{90}^{o}}-\frac{C}{2}\] and sides are \[{{I}_{1}}{{I}_{3}}=4R\cos \frac{B}{2};\,\,{{I}_{1}}{{I}_{2}}=4R\cos \frac{C}{2};\,\,{{I}_{2}}{{I}_{3}}=4R\cos \frac{A}{2}\].
Area and circum-radius of the ex-central triangle
Area of triangle
\[=\frac{1}{2}\] (Product of two sides) \[\times \] (sine of included angles)
\[\Delta =\frac{1}{2}\,\,\left( 4R\cos \frac{B}{2} \right)\,\,.\,\,\left( 4R\cos \frac{C}{2} \right)\times \sin \left( {{90}^{o}}-\frac{A}{2} \right)\]
\[\Delta =8{{R}^{2}}\cos \frac{A}{2}.\cos \frac{B}{2}.\cos \frac{C}{2}\]
Circum-radius \[=\frac{{{I}_{2}}{{I}_{3}}}{2\sin {{I}_{2}}{{I}_{1}}{{I}_{3}}}=\frac{4R\cos \frac{A}{2}}{2\sin \left( {{90}^{o}}-\frac{A}{2} \right)}=2R\].
Let the perpendiculars AD, BE and CF from the vertices A, B and C on the opposite sides BC, CA and AB of \[\Delta ABC\] respectively, meet at O. Then O is the orthocentre of the \[\Delta ABC\]. The triangle DEF is called the pedal triangle of the \[\Delta ABC\].
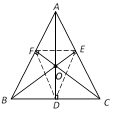 Othocentre of the triangle is the incentre of the pedal triangle.
If O is the orthocentre and DEF the pedal triangle of the \[\Delta ABC\], where AD, BE, CF are the perpendiculars drawn from A, B, C on the opposite sides BC, CA, AB respectively, then
(i) \[OA=2R\cos A,OB=2R\cos B\]and \[OC=2R\cos C\]
(ii) \[OD=2R\cos B\cos C,OE=2R\cos C\cos A\] and \[OF=2R\cos A\cos B\]
(1) Sides and angles of a pedal triangle: The angles of pedal triangle DEF are: \[180-2A,\,180-2B,\,180-2C\] and sides of pedal triangle are:
\[EF=a\cos A\] or \[R\sin 2A\]; \[FD=b\cos B\] or \[R\sin 2B\]; \[DE=c\cos C\] or \[R\sin 2C\]
Othocentre of the triangle is the incentre of the pedal triangle.
If O is the orthocentre and DEF the pedal triangle of the \[\Delta ABC\], where AD, BE, CF are the perpendiculars drawn from A, B, C on the opposite sides BC, CA, AB respectively, then
(i) \[OA=2R\cos A,OB=2R\cos B\]and \[OC=2R\cos C\]
(ii) \[OD=2R\cos B\cos C,OE=2R\cos C\cos A\] and \[OF=2R\cos A\cos B\]
(1) Sides and angles of a pedal triangle: The angles of pedal triangle DEF are: \[180-2A,\,180-2B,\,180-2C\] and sides of pedal triangle are:
\[EF=a\cos A\] or \[R\sin 2A\]; \[FD=b\cos B\] or \[R\sin 2B\]; \[DE=c\cos C\] or \[R\sin 2C\]
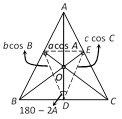 If given \[\Delta ABC\] is obtuse, then angles are represented by \[2A,\] \[2B\], \[2C-{{180}^{o}}\] and the sides are \[a\cos A,\,\,b\cos B,\,\,-\,\,c\cos C\].
(2) Area and circum-radius and in-radius of pedal triangle : Area of pedal triangle \[=\frac{1}{2}(\text{Product of the sides)}\times \] (sine of included angle)
\[\Delta =\frac{1}{2}{{R}^{2}}.\sin 2A.\sin 2B.\sin 2C\]
Circum-radius of pedal triangle\[=\frac{EF}{2\sin FDE}=\frac{R\sin 2A}{2\sin ({{180}^{o}}-2A)}=\frac{R}{2}\]
In-radius of pedal triangle \[=\frac{\text{area of }\Delta DEF}{\text{semi-perimeter of }\Delta DEF}\]
\[=\frac{\frac{1}{2}{{R}^{2}}\sin 2A.\sin 2B.\sin 2C}{2R\sin A.\sin B.\sin C}=2R\cos A.\cos B.\cos C\]
If given \[\Delta ABC\] is obtuse, then angles are represented by \[2A,\] \[2B\], \[2C-{{180}^{o}}\] and the sides are \[a\cos A,\,\,b\cos B,\,\,-\,\,c\cos C\].
(2) Area and circum-radius and in-radius of pedal triangle : Area of pedal triangle \[=\frac{1}{2}(\text{Product of the sides)}\times \] (sine of included angle)
\[\Delta =\frac{1}{2}{{R}^{2}}.\sin 2A.\sin 2B.\sin 2C\]
Circum-radius of pedal triangle\[=\frac{EF}{2\sin FDE}=\frac{R\sin 2A}{2\sin ({{180}^{o}}-2A)}=\frac{R}{2}\]
In-radius of pedal triangle \[=\frac{\text{area of }\Delta DEF}{\text{semi-perimeter of }\Delta DEF}\]
\[=\frac{\frac{1}{2}{{R}^{2}}\sin 2A.\sin 2B.\sin 2C}{2R\sin A.\sin B.\sin C}=2R\cos A.\cos B.\cos C\]
(1) Circumcircle of a triangle and its radius
(i) Circumcircle : The circle which passes through the angular points of a triangle is called its circumcircle. The centre of this circle is the point of intersection of perpendicular bisectors of the sides and is called the circumcentre. Its radius is always denoted by R. The circumcentre may lie within, outside or upon one of the sides of the triangle.
 (ii) Circum-radius : The circum-radius of a \[\Delta ABC\]is given by
(a) \[\frac{a}{2\sin A}=\frac{b}{2\sin B}=\frac{c}{2\sin C}=R\]
(b) \[R=\frac{abc}{4\Delta }\] \[[\Delta =\]area of \[\Delta ABC\]]
(2) Inscribed circle or in-circle of a triangle and its radius
(i) In-circle or inscribed circle : The circle which can be inscribed within a triangle so as to touch each of its sides is called its inscribed circle or in circle. The centre of this circle is the point of intersection of the bisectors of the angles of the triangle. The radius of this circle is always denoted by \[r\] and is equal to the length of the perpendicular from its centre to any one of the sides of triangle.
(ii) Circum-radius : The circum-radius of a \[\Delta ABC\]is given by
(a) \[\frac{a}{2\sin A}=\frac{b}{2\sin B}=\frac{c}{2\sin C}=R\]
(b) \[R=\frac{abc}{4\Delta }\] \[[\Delta =\]area of \[\Delta ABC\]]
(2) Inscribed circle or in-circle of a triangle and its radius
(i) In-circle or inscribed circle : The circle which can be inscribed within a triangle so as to touch each of its sides is called its inscribed circle or in circle. The centre of this circle is the point of intersection of the bisectors of the angles of the triangle. The radius of this circle is always denoted by \[r\] and is equal to the length of the perpendicular from its centre to any one of the sides of triangle.
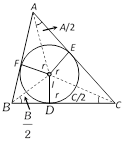 (ii) In-radius : The radius r of the inscribed circle of a triangle ABC is given by
(a) \[r=\frac{\Delta }{s}\]
(b) \[r=4R\sin \frac{A}{2}\sin \frac{B}{2}\sin \frac{C}{2}\]
(c) \[r=(s-a)\tan \frac{A}{2},\,\,r=(s-b)\tan \frac{B}{2},\,\,r=(s-c)\tan \frac{C}{2}\]
(d) \[r=\frac{a\sin \frac{B}{2}\sin \frac{C}{2}}{\cos \frac{A}{2}},r=\frac{b\sin \frac{A}{2}\sin \frac{C}{2}}{\cos \frac{B}{2}},r=\frac{c\sin \frac{B}{2}\sin \frac{A}{2}}{\cos \frac{C}{2}}\]
(e) \[\cos A+\cos B+\cos C=1+\frac{r}{R}\]
(3) Escribed circles of a triangle and their radii
(i) Escribed circle : The circle which touches the side BC and two sides AB and AC produced of a triangle ABC is called the escribed circle opposite to the angle A. Its radius is denoted by \[{{r}_{1}}\]. Similarly, \[{{r}_{2}}\] and \[{{r}_{3}}\] denote the radii of the escribed circles opposite to the angles B and C respectively.
The centres of the escribed circles are called the ex-centres. The centre of the escribed circle opposite to the angle A is the point of intersection of the external bisectors of angles B and C. The internal bisectors of angle A also passes through the same point. The centre is generally denoted by I1.
(ii) In-radius : The radius r of the inscribed circle of a triangle ABC is given by
(a) \[r=\frac{\Delta }{s}\]
(b) \[r=4R\sin \frac{A}{2}\sin \frac{B}{2}\sin \frac{C}{2}\]
(c) \[r=(s-a)\tan \frac{A}{2},\,\,r=(s-b)\tan \frac{B}{2},\,\,r=(s-c)\tan \frac{C}{2}\]
(d) \[r=\frac{a\sin \frac{B}{2}\sin \frac{C}{2}}{\cos \frac{A}{2}},r=\frac{b\sin \frac{A}{2}\sin \frac{C}{2}}{\cos \frac{B}{2}},r=\frac{c\sin \frac{B}{2}\sin \frac{A}{2}}{\cos \frac{C}{2}}\]
(e) \[\cos A+\cos B+\cos C=1+\frac{r}{R}\]
(3) Escribed circles of a triangle and their radii
(i) Escribed circle : The circle which touches the side BC and two sides AB and AC produced of a triangle ABC is called the escribed circle opposite to the angle A. Its radius is denoted by \[{{r}_{1}}\]. Similarly, \[{{r}_{2}}\] and \[{{r}_{3}}\] denote the radii of the escribed circles opposite to the angles B and C respectively.
The centres of the escribed circles are called the ex-centres. The centre of the escribed circle opposite to the angle A is the point of intersection of the external bisectors of angles B and C. The internal bisectors of angle A also passes through the same point. The centre is generally denoted by I1.
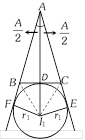 (ii) Radii of ex-circles
In any \[\Delta ABC\], we have
(a) \[{{r}_{1}}=\frac{\Delta }{s-a},{{r}_{2}}=\frac{\Delta }{s-b},{{r}_{3}}=\frac{\Delta }{s-c}\]
(b) \[{{r}_{1}}=s\tan \frac{A}{2},\,\,\,{{r}_{2}}=s\tan \frac{B}{2},\,\,{{r}_{3}}=s\tan \frac{C}{2}\]
(c) \[{{r}_{1}}=\frac{a\cos \frac{B}{2}\cos \frac{C}{2}}{\cos \frac{A}{2}},\,{{r}_{2}}=\frac{b\cos \frac{C}{2}\cos \frac{A}{2}}{\cos \frac{B}{2}},{{r}_{3}}=\frac{c\cos \frac{A}{2}\cos \frac{B}{2}}{\cos \frac{C}{2}}\]
(d) \[{{r}_{1}}+{{r}_{2}}+{{r}_{3}}-r=4R\]
(e) \[\frac{1}{{{r}_{1}}}+\frac{1}{{{r}_{2}}}+\frac{1}{{{r}_{3}}}=\frac{1}{r}\]
(f) \[\frac{1}{{{r}^{2}}}+\frac{1}{r_{1}^{2}}+\frac{1}{r_{2}^{2}}+\frac{1}{r_{3}^{2}}=\frac{{{a}^{2}}+{{b}^{2}}+{{c}^{2}}}{{{\Delta }^{2}}}\]
(g) \[\frac{1}{bc}+\frac{1}{ca}+\frac{1}{ab}=\frac{1}{2Rr}\]
(h) \[{{r}_{1}}{{r}_{2}}+{{r}_{2}}{{r}_{3}}+{{r}_{3}}{{r}_{1}}={{s}^{2}}\]
(i) \[\Delta more...
(ii) Radii of ex-circles
In any \[\Delta ABC\], we have
(a) \[{{r}_{1}}=\frac{\Delta }{s-a},{{r}_{2}}=\frac{\Delta }{s-b},{{r}_{3}}=\frac{\Delta }{s-c}\]
(b) \[{{r}_{1}}=s\tan \frac{A}{2},\,\,\,{{r}_{2}}=s\tan \frac{B}{2},\,\,{{r}_{3}}=s\tan \frac{C}{2}\]
(c) \[{{r}_{1}}=\frac{a\cos \frac{B}{2}\cos \frac{C}{2}}{\cos \frac{A}{2}},\,{{r}_{2}}=\frac{b\cos \frac{C}{2}\cos \frac{A}{2}}{\cos \frac{B}{2}},{{r}_{3}}=\frac{c\cos \frac{A}{2}\cos \frac{B}{2}}{\cos \frac{C}{2}}\]
(d) \[{{r}_{1}}+{{r}_{2}}+{{r}_{3}}-r=4R\]
(e) \[\frac{1}{{{r}_{1}}}+\frac{1}{{{r}_{2}}}+\frac{1}{{{r}_{3}}}=\frac{1}{r}\]
(f) \[\frac{1}{{{r}^{2}}}+\frac{1}{r_{1}^{2}}+\frac{1}{r_{2}^{2}}+\frac{1}{r_{3}^{2}}=\frac{{{a}^{2}}+{{b}^{2}}+{{c}^{2}}}{{{\Delta }^{2}}}\]
(g) \[\frac{1}{bc}+\frac{1}{ca}+\frac{1}{ab}=\frac{1}{2Rr}\]
(h) \[{{r}_{1}}{{r}_{2}}+{{r}_{2}}{{r}_{3}}+{{r}_{3}}{{r}_{1}}={{s}^{2}}\]
(i) \[\Delta more...
If 2s shows the perimeter of a triangle ABC then, i.e., \[2s=a+b+c,\] then
(1) Formulae for \[sin\frac{A}{2},sin\frac{B}{2},sin\frac{C}{2}\]
(i) \[\sin \frac{A}{2}=\sqrt{\frac{(s-b)(s-c)}{bc}}\]
(ii) \[\sin \frac{B}{2}=\sqrt{\frac{(s-a)(s-c)}{ca}}\]
(iii) \[\sin \frac{C}{2}=\sqrt{\frac{(s-a)(s-b)}{ab}}\]
(2) Formulae for \[cos\frac{A}{2},cos\frac{B}{2},cos\frac{C}{2}\]
(i) \[\cos \frac{A}{2}=\sqrt{\frac{s(s-a)}{bc}}\]
(ii) \[\cos \frac{B}{2}=\sqrt{\frac{s(s-b)}{ca}}\]
(iii) \[\cos \frac{C}{2}=\sqrt{\frac{s(s-c)}{ab}}\]
(3) Formulae for \[tan\frac{A}{2},tan\frac{B}{2},tan\frac{C}{2}\]
(i) \[\tan \frac{A}{2}=\sqrt{\frac{(s-b)(s-c)}{s(s-a)}}\]
(ii) \[\tan \frac{B}{2}=\sqrt{\frac{(s-c)(s-a)}{s(s-b)}}\]
(iii) \[\tan \frac{C}{2}=\sqrt{\frac{(s-a)(s-b)}{s(s-c)}}\]
Current Affairs CategoriesArchive
Trending Current Affairs
You need to login to perform this action. | |||||||||||||