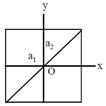
| Two springs, of force constants\[{{I}_{0}}=\frac{E}{R}=\frac{5}{5}=1\,A\]and\[\tau =\frac{L}{R}=\frac{10}{5}=2\,s\]are connected to a mass m as shown. The frequency of oscillation of the mass is\[t=2s\]. If\[I=(1-{{e}^{-1}})A\]both\[(\therefore -t/\tau =\frac{-2}{2}=-1)\]and\[J=\frac{i}{\pi {{a}^{2}}}\]are made four times their original values, the frequency of. oscillation becomes [AIEEE 2007] |
| |
| A ring is suspended from a point S on its rim as shown in the figure. When displaced from equilibrium, it oscillates with time period of 1 second. The radius of the ring is (take \[g={{\pi }^{2}}\]) [JEE ONLINE 19-05-2012] |
 |
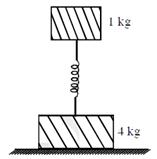
| Two bodies of masses 1 kg and 4 kg are connected to a vertical spring, as shown in the figure. The smaller mass executes simple harmonic motion of angular frequency 25rad/s, and amplitude 1.6 cm while the bigger mass remains stationary on the ground. The maximum force exerted by the system on the floor is (take \[g=10m{{s}^{-2}}\]) [JEE ONLINE 09-04-2014] |
 |
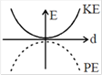
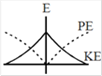

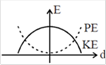
|
done
clear
View Answer play_arrow
question_answer45) The period of oscillation of a simple pendulum is \[T=2\pi \sqrt{\frac{L}{g}}.\]Measured value of L is 20.0 cm known to 1 mm accuracy and time for 100 oscillations of the pendulum is found to be 90 s using a wrist watch of 1s resolution. The accuracy in the determination of g is: [JEE MAIN 2015]
A)
1%
done
clear
B)
5%
done
clear
C)
2%
done
clear
D)
3%
done
clear
View Answer play_arrow
question_answer46) A simple harmonic oscillator of angular frequency \[2rad\,{{s}^{-1}}\]is acted upon by an external force \[F=\sin \,t\,N\]. If the oscillator is at rest in its equilibrium position at t = 0, its position at later times is proportional to: [JEE ONLINE 10-04-2015]
A)
\[\sin t+\frac{1}{2}\cos 2t\]
done
clear
B)
\[\sin t-\frac{1}{2}\sin 2t\]
done
clear
C)
\[\cot t-\frac{1}{2}\sin 2t\]
done
clear
D)
\[\sin t+\frac{1}{2}\sin 2t\]
done
clear
View Answer play_arrow
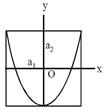
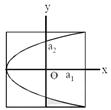
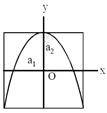
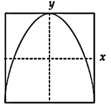
question_answer47) x and y displacements of a particle are given as x(t)=a \[\sin \omega t\] and \[y(t)=a\sin 2\omega t.\] Its trajectory will look like: [JEE ONLINE 10-04-2015]
A)

B)
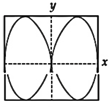
C)
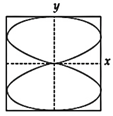
D)
question_answer48) A cylindrical block of wood (density \[=650\,kg\,{{m}^{-3}}\]), of base area \[30\text{ }c{{m}^{2}}\]and height 54 cm, floats in a liquid of density \[900\text{ }kg{{m}^{-}}^{3}.\]The block is depressed slightly and then released. The time period of the resulting oscillations of the block would be equal to that of a simple pendulum of length (nearly): [JEE MAIN 11-04-2015]
A)
65 cm
done
clear
B)
52 cm
done
clear
C)
39 cm
done
clear
D)
26 cm
done
clear
View Answer play_arrow
question_answer49) A pendulum with time period of 1s is losing energy due to damping. At certain time its energy is 45 J. If after completing 15 oscillations, its energy has become 15 J, its damping constant (in\[{{s}^{-1}}\]) is: [JEE MAIN 11-04-2015]
A)
\[\frac{1}{30}\ln 3\]
done
clear
B)
\[\frac{1}{15}\ln 3\]
done
clear
C)
2
done
clear
D)
\[\frac{1}{2}\]
done
clear
View Answer play_arrow
question_answer50) A particle performs simple harmonic motion with amplitude A. Its speed is trebled at the instant that it is at a distance\[\frac{2A}{3}\]from equilibrium position. [JEE MAIN - I 3-4-2016] The new amplitude of the motion is:-
A)
\[\frac{7A}{3}\]
done
clear
B)
\[\frac{A}{3}\sqrt{41}\]
done
clear
C)
3A
done
clear
D)
A 3
done
clear
View Answer play_arrow
question_answer51)
A)
\[\frac{T}{4}\]
done
clear
B)
\[\frac{5T}{6}\]
done
clear
C)
\[\frac{T}{3}\]
done
clear
D)
\[\frac{T}{6}\]
done
clear
View Answer play_arrow
question_answer52) In an engine the piston undergoes vertical simple harmonic motion with amplitude 7cm. A washer rests on top of the piston and moves with it. The motor speed is slowly increased. The frequency of the piston at which the washer longer stays in contact with the piston, is closed to: [JEE ONLINE 10-04-2016]
A)
0.7 Hz
done
clear
B)
1.2 Hz
done
clear
C)
1.9 Hz
done
clear
D)
0.1 Hz
done
clear
View Answer play_arrow
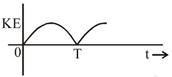
question_answer53) A particle is executing simple harmonic motion with a time period T. AT time t = 0, it is at its position of equilibrium. The kinetic energy-time graph of the particle will look like [JEE Main 2017]
A)
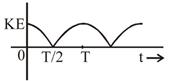
B)
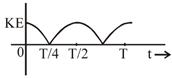
C)
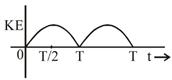
D)
question_answer54) A 1 kg block attached to a spring vibrates with a frequency of 1 Hz on a frictionless horizontal table. Two springs identical to the original spring are attached in parallel to an 8 kg block placed on the same table. So, the frequency of vibration of the 8 kg block is [JEE Online 08-04-2017]
A)
2 Hz
done
clear
B)
\[\frac{1}{4}Hz\]
done
clear
C)
\[\frac{1}{2\sqrt{2}}Hz\]
done
clear
D)
\[\frac{1}{2}Hz\]
done
clear
View Answer play_arrow
question_answer55)
A)
\[500m/{{s}^{2}}\]
done
clear
B)
\[750\sqrt{2}m/{{s}^{2}}\]
done
clear
C)
\[750m/{{s}^{2}}\]
done
clear
D)
\[500\sqrt{2}m/{{s}^{2}}\]
done
clear
View Answer play_arrow
question_answer56) A block of mass 0.1 kg is connected to an elastic spring of spring constant \[640\,\,N{{m}^{-1}}\] and oscillates in a damping medium of damping constant \[{{10}^{-2}}kg\,{{s}^{-1}}\]. The system dissipates its energy gradually. The time taken for its mechanical energy of vibration to drop to half of its initial value, is closest to [JEE Online 09-04-2017]
A)
2 s
done
clear
B)
5 s
done
clear
C)
7 s
done
clear
D)
3.5 s
done
clear
View Answer play_arrow
question_answer57) In an experiment to determine the period of a simple pendulum of length 1m, it is attached to different spherical bobs of radii \[{{r}_{1}}\] and \[{{r}_{2}}\]. The two spherical bobs have uniform mass distribution. If the relative difference in the periods, is found to be \[5\times {{10}^{-4}}s,\] the difference in radii, \[|{{r}_{1}}-{{r}_{2}}|\] is best given by [JEE Online 09-04-2017]
A)
0.01 cm
done
clear
B)
(2) 0.1 cm
done
clear
C)
0.5 cm
done
clear
D)
1 cm
done
clear
View Answer play_arrow
question_answer58) A silver atom in a solid oscillates in simple harmonic motion in some direction with a frequency of \[{{10}^{12}}/\sec \]. What is the force constant of the bonds connecting one atom with the other? (Mole wt. of silver = 108 and Avagadro number\[\text{=6}\text{.02}\times \text{1}{{\text{0}}^{\text{23}}}\text{ gm mol}{{\text{e}}^{\text{-1}}}\]). [JEE Main Online 08-04-2018]
A)
\[2.2\,\,N/m\]
done
clear
B)
\[5.5\,\,N/m\]
done
clear
C)
\[6.4\,\,N/m\]
done
clear
D)
\[7.1\,\,N/m\]
done
clear
View Answer play_arrow
question_answer59)
A)
\[\text{Parameters: A=B, a=2b;}\delta \text{=}\frac{\pi }{2};Curve:Circle\]
done
clear
B)
\[\text{Parameters: A=B, a=b;}\delta \text{=}\frac{\pi }{2};Curve:line\]
done
clear
C)
\[\text{Parameters: A}\ne \text{B, a=b; }\delta \text{=}\frac{\pi }{2};Curve:\text{Ellipse}\]
done
clear
D)
\[\text{Parameters:}A\ne B,a=b;\delta =0;\text{Curve : Parabola}\]
done
clear
View Answer play_arrow
question_answer60) An oscillator of mass M is at rest in its equilibrium position in a potential \[V=\frac{1}{2}k{{(x-X)}^{2}}.\]A particle of mass comes from right with speed u and collides completely in elastically with and sticks to it. This process repeats every time the oscillator crosses its equilibrium position. The amplitude of oscillations after collisions is:\[(M=10,m=5,u=1,k=1).\] [JEE Main Online 16-4-2018]
A)
\[\frac{1}{2}\]
done
clear
B)
\[\frac{1}{\sqrt{3}}\]
done
clear
C)
\[\frac{2}{3}\]
done
clear
D)
\[\sqrt{\frac{3}{5}}\]
done
clear
View Answer play_arrow
question_answer61) A rod of mass M and length 2L is suspended at its middle by a wire. It exhibits torsional oscillations; If two masses each of m are attached at distance L/2 from its centre on both sides, it reduces the oscillation frequency by \[20%\]. The value of ratio m/M is close to: [JEE Main 09-Jan-2019 Evening]
A)
0.37
done
clear
B)
0.57
done
clear
C)
0.77
done
clear
D)
0.17
done
clear
View Answer play_arrow
question_answer62) A particle is executing simple harmonic motion (SHM) of amplitude A, along the x-axis, about\[x=0\]. When its potential Energy (PE) equals kinetic energy (KE), the position of the particle will be: [JEE Main 09-Jan-2019 Evening]
A)
\[\frac{A}{2\sqrt{2}}\]
done
clear
B)
\[\frac{A}{\sqrt{2}}\]
done
clear
C)
\[\frac{A}{2}\]
done
clear
D)
A
done
clear
View Answer play_arrow
question_answer63) A cylindrical plastic bottle of negligible mass is filled with 310 ml of water and left floating in a pond with still water. If pressed downward slightly and released, it starts performing simple harmonic motion at angular frequency co. If the radius of the bottle is 2.5 cm then co is close to - (density of water\[={{10}^{3}}kg/{{m}^{3}}\]). [JEE Main 10-Jan-2019 Evening]
A)
\[2.50\text{ }rad\text{ }{{s}^{-}}^{1}\]
done
clear
B)
\[3.75\text{ }rad\text{ }{{s}^{-1}}\]
done
clear
C)
\[5.00\text{ }rad\text{ }{{s}^{-1}}\]
done
clear
D)
None of these
done
clear
View Answer play_arrow
question_answer64) A particle executes simple harmonic motion with an amplitude of 5 cm. When the particle is at 4 cm from the mean position, the magnitude of its velocity in SI units is equal to that of its acceleration. Then, its periodic time in seconds is- [JEE Main 10-Jan-2019 Evening]
A)
\[\frac{4\pi }{3}\]
done
clear
B)
\[\frac{3\,}{8}\pi \]
done
clear
C)
\[\frac{7\,}{3}\pi \]
done
clear
D)
\[\frac{8\,\pi }{3}\]
done
clear
View Answer play_arrow
question_answer65) A particle undergoing simple harmonic motion has time dependent displacement given by \[x(t)=A\sin \frac{\pi t}{90}.\] The ratio of kinetic to potential energy of this particle at t = 210 s will be- [JEE Main 11-Jan-2019 Morning]
A)
\[\frac{1}{9}\]
done
clear
B)
2
done
clear
C)
1
done
clear
D)
None of these
done
clear
View Answer play_arrow
question_answer66) A pendulum is executing simple harmonic motion and its maximum kinetic energy is \[{{K}_{1}}\]. If the length of the pendulum is doubled and it performs simple harmonic motion with the same amplitude as in the first case. its maximum kinetic energy is \[{{K}_{2}}\]. Then [JEE Main 11-Jan-2019 Evening]
A)
\[{{K}_{2}}={{K}_{1}}\]
done
clear
B)
\[{{K}_{2}}=\frac{{{K}_{1}}}{2}\]
done
clear
C)
\[{{K}_{2}}=2{{K}_{1}}\]
done
clear
D)
\[{{K}_{2}}=\frac{{{K}_{1}}}{4}\]
done
clear
View Answer play_arrow
question_answer67) The mass and the diameter of a planet are three times the respective values for the Earth. The period of oscillation of a simple pendulum on the Earth is 2 s. The period of oscillation of the same pendulum on the planet would be- [JEE Main 11-Jan-2019 Evening]
A)
\[2\sqrt{3}s\]
done
clear
B)
\[\frac{3}{2}s\]
done
clear
C)
\[\frac{2}{\sqrt{3}}s\]
done
clear
D)
\[\frac{\sqrt{3}}{2}s\]
done
clear
View Answer play_arrow
question_answer68) A simple pendulum of length 1 m is oscillating with an angular frequency 10 rad/s. The support of the pendulum starts oscillating up and down with a small angular frequency of 1 rad/s and an amplitude of \[{{10}^{-2}}\]m. The relative change in the angular frequency of the pendulum is best given by- [JEE Main 11-Jan-2019 Evening]
A)
\[{{10}^{-5}}rad/s\]
done
clear
B)
\[{{10}^{-1}}rad/s\]
done
clear
C)
\[1rad/s\]
done
clear
D)
\[{{10}^{-3}}rad/s\]
done
clear
View Answer play_arrow
question_answer69) A simple pendulum, made of a string of length I and a bob of mass m, is released from a small angle \[{{\theta }_{0}}.\]It strikes a block of mass M, kept on a horizontal surface at its lowest point of oscillations, elastically. It bounces back and goes up to an angle \[{{\theta}_{1}}.\]Then M is given by- [JEE Main 12-Jan-2019 Morning]
A)
\[\frac{m}{2}\left( \frac{{{\theta }_{0}}-{{\theta }_{1}}}{{{\theta }_{0}}+{{\theta }_{1}}} \right)\]
done
clear
B)
\[m\left( \frac{{{\theta }_{0}}+{{\theta }_{1}}}{{{\theta }_{0}}-{{\theta }_{1}}} \right)\]
done
clear
C)
\[\frac{m}{2}\left( \frac{{{\theta }_{0}}+{{\theta }_{1}}}{{{\theta }_{0}}-{{\theta }_{1}}} \right)\]
done
clear
D)
\[m\left( \frac{{{\theta }_{0}}-{{\theta }_{1}}}{{{\theta }_{0}}+{{\theta }_{1}}} \right)\]
done
clear
View Answer play_arrow
question_answer70)
A)
\[5cm,\frac{3}{2}s\]
done
clear
B)
\[10cm,\frac{2}{3}s\]
done
clear
C)
\[5cm,\frac{2}{3}s\]
done
clear
D)
\[10cm,\frac{3}{2}s\]
done
clear
View Answer play_arrow
question_answer71) A damped harmonic oscillator has a frequency of 5 oscillations per second. The amplitude drops to half its value for every 10 oscillations. The time it will take to drop to \[\frac{1}{1000}\]of the original amplitude is close to: [JEE Main 8-4-2019 Afternoon]
A)
100 s
done
clear
B)
20 s
done
clear
C)
10 s
done
clear
D)
50 s
done
clear
View Answer play_arrow
question_answer72) In a simple pendulum experiment for determination of acceleration due to gravity (g), time taken for 20 oscillations is measured by using a watch of 1 second least count. The mean value of time taken comes out to be 30 s. The length of pendulum is measured by using a meter scale of least count 1 mm and the value obtained is 55.0 cm. The percentage error in the determination of g is close to:- [JEE Main 8-4-2019 Afternoon]
A)
0.7%
done
clear
B)
0.2%
done
clear
C)
3.5%
done
clear
D)
6.8%
done
clear
View Answer play_arrow
question_answer73) A simple pendulum oscillating in air has period T. The bob of the pendulum is completely immersed in a non-viscous liquid. The density of the liquid is \[\frac{1}{16}\]th of the material of the bob. If the bob is inside liquid all the time, its period of oscillation in this liquid is : [JEE Main 9-4-2019 Morning]
A)
\[4T\sqrt{\frac{1}{15}}\]
done
clear
B)
\[2T\sqrt{\frac{1}{10}}\]
done
clear
C)
\[4T\sqrt{\frac{1}{14}}\]
done
clear
D)
\[2T\sqrt{\frac{1}{14}}\]
done
clear
View Answer play_arrow
question_answer74) A string 2.0 m long and fixed at its ends is driven by a 240 Hz vibrator. The string vibrates in its third harmonic mode. The speed of the wave and its fundamental frequency is :- [JEE Main 9-4-2019 Afternoon]
A)
320m/s, 120 Hz
done
clear
B)
180m/s, 80 Hz
done
clear
C)
180m/s, 120 Hz
done
clear
D)
320m/s, 80 Hz
done
clear
View Answer play_arrow
question_answer75) The displacement of a damped harmonic oscillator is given by \[x(t)={{e}^{-01.1t}}\cos (10\pi t+\phi ).\]Here t is in seconds. The time taken for its amplitude of vibration to drop to half of its initial value is close to: [JEE Main 10-4-2019 Morning]
A)
13 s
done
clear
B)
7 s
done
clear
C)
27 s
done
clear
D)
4 s
done
clear
View Answer play_arrow
question_answer76) A simple pendulum is being used to determine the value of gravitational acceleration g at a certain place. The length of the pendulum is 25.0 cm and a stopwatch with 1 s resolution measures the time taken for 40 oscillation to be 50 s. The accuracy in g is [JEE MAIN Held on 08-01-2020 Evening]
A)
3.40%
done
clear
B)
2.40%
done
clear
C)
5.40%
done
clear
D)
4.40%
done
clear
View Answer play_arrow
Study Package
You need to login to perform this action. |