 Ascending Order of numbers:
Arranging numbers from the least number to the largest number is called Ascending Order.
Ex: 13, 67, 132, 168 are in ascending order
Descending Order of numbers:
Arranging numbers from the largest number to the least number is called Descending Order.
Ex: 168, 132, 67, 13 are in descending order.
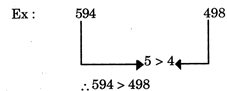
Symbols used for comparison of two numbers:
Ascending Order of numbers:
Arranging numbers from the least number to the largest number is called Ascending Order.
Ex: 13, 67, 132, 168 are in ascending order
Descending Order of numbers:
Arranging numbers from the largest number to the least number is called Descending Order.
Ex: 168, 132, 67, 13 are in descending order.
Symbols used for comparison of two numbers:
 Rule 3:
If the left most digits are also the same, we go to next digit from left and compare.
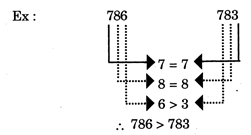
Rule 3:
If the left most digits are also the same, we go to next digit from left and compare.
 Successor of a numeral:
Successor of a particular numeral comes just after that numeral. So, we can find out the successor of a numeral by adding 1 to the given numeral.
Ex : The successor of 99 is 99 + 1 or 100.
Predecessor of a numeral:
Predecessor of a particular numeral comes just before that numeral. So, we can find out the
predecessor of a numeral by subtracting 1 from more...
Successor of a numeral:
Successor of a particular numeral comes just after that numeral. So, we can find out the successor of a numeral by adding 1 to the given numeral.
Ex : The successor of 99 is 99 + 1 or 100.
Predecessor of a numeral:
Predecessor of a particular numeral comes just before that numeral. So, we can find out the
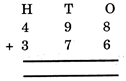
predecessor of a numeral by subtracting 1 from more... 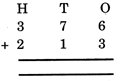 Step-2: Add the ONES first, then the TENs and finally the HUNDREDS.
Step-2: Add the ONES first, then the TENs and finally the HUNDREDS.
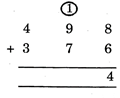 Step - 3: Add tens
1 + 9 + 7 = 17
17 tens = I hundred + 7 tens
1 hundred is carried to hundreds column.
7 is written in tens place.
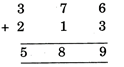
Step - 3: Add tens
1 + 9 + 7 = 17
17 tens = I hundred + 7 tens
1 hundred is carried to hundreds column.
7 is written in tens place.
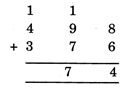 Step - 4: Add Hundreds
1+4+3=8
8 is written in Hundreds place.
Step - 4: Add Hundreds
1+4+3=8
8 is written in Hundreds place.
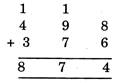 Subtraction:
Subtraction:
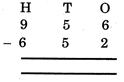 Step-2: Subtract ONES first, then the TENS and finally the HUNDREDS.
Step-2: Subtract ONES first, then the TENS and finally the HUNDREDS.
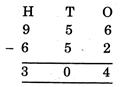
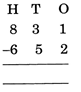 Step - 2: Subtract ONES
1 – 2 = ?
We can't subtract 2 from 1. more...
Step - 2: Subtract ONES
1 – 2 = ?
We can't subtract 2 from 1. more... 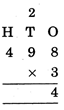 Step - 2: Multiply 9 tens by 3.
9 tens \[\times \] 3 = 27 tens
= 20 tens + 7 tens
= 2 hundreds + 7 tens
Carry 2 hundreds to the hundreds place.
Add the carried 2 tens to 7 tens.
So 7 tens + 2 tens = 9 tens,
write 9 in the tens place
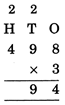
Step - 2: Multiply 9 tens by 3.
9 tens \[\times \] 3 = 27 tens
= 20 tens + 7 tens
= 2 hundreds + 7 tens
Carry 2 hundreds to the hundreds place.
Add the carried 2 tens to 7 tens.
So 7 tens + 2 tens = 9 tens,
write 9 in the tens place
 Step - 3: Multiply 4 hundreds by 3.
hundreds x 3 = 12 hundreds.
Add the carried 2 hundreds to 12 hundreds.
So, 12 hundreds + 2 hundreds = 14 hundreds,
write 14 in hundreds place
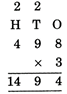
Step - 3: Multiply 4 hundreds by 3.
hundreds x 3 = 12 hundreds.
Add the carried 2 hundreds to 12 hundreds.
So, 12 hundreds + 2 hundreds = 14 hundreds,
write 14 in hundreds place
 Multiplication by TENS and HUNDREDS:
5 \[\times \] 10 = 5 \[\times \]1 ten = 5 tens = 50
7\[\times \] 30 = 7 \[\times \] 3 tens = 21 tens = 210
15 x 60 = 15 \[\times \] 6 tens = 90 tens = 900
8\[\times \]100=8\[\times \] 1 hundred = 8 hundreds = 800
9 \[\times \] 400 = 9 \[\times \] 4 hundreds = 36 hundreds = 3600
Multiplication by 2-digit numbers:
Ex: Multiply 36 by 25
Multiplication by TENS and HUNDREDS:
5 \[\times \] 10 = 5 \[\times \]1 ten = 5 tens = 50
7\[\times \] 30 = 7 \[\times \] 3 tens = 21 tens = 210
15 x 60 = 15 \[\times \] 6 tens = 90 tens = 900
8\[\times \]100=8\[\times \] 1 hundred = 8 hundreds = 800
9 \[\times \] 400 = 9 \[\times \] 4 hundreds = 36 hundreds = 3600
Multiplication by 2-digit numbers:
Ex: Multiply 36 by 25

 we have, 12-3 =9 apples left.
Step - 2: Again give 1 apple to each boy.
we have, 12-3 =9 apples left.
Step - 2: Again give 1 apple to each boy.
 we have, 9-3=6 apples left.
Step - 3: Give 1 more apple to each boy
we have, 9-3=6 apples left.
Step - 3: Give 1 more apple to each boy
 We have, 6-3=3 apples left.
Step - 4: Again give 1 more apple to each boy.
We have, 6-3=3 apples left.
Step - 4: Again give 1 more apple to each boy.
 We have, 3-3=0. Each boy has got 4 apples and no apple is left.
We observed that 12 apples have been distributed among 3 boys. Each boy got 4 apples.
Thus 12- 3=4
Grouping:
Ex: 9 balls are to be distributed among some boys. Each boy should get 3 balls. How many boys are there?
Step -1: Give 3 balls to one boy
We have, 3-3=0. Each boy has got 4 apples and no apple is left.
We observed that 12 apples have been distributed among 3 boys. Each boy got 4 apples.
Thus 12- 3=4
Grouping:
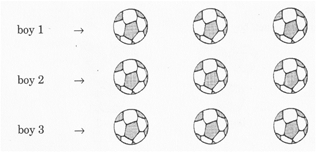
Ex: 9 balls are to be distributed among some boys. Each boy should get 3 balls. How many boys are there?
Step -1: Give 3 balls to one boy
 We have, 9 - 3=6 balls left.
Step - 2: Give 3 balls to the next boy.
We have, 6 - 3=3 balls left
Step - 3: Give 3 balls to the next boy.
We have, 3 - 3 =0
No balls left. We observed that, Three boys got the balls.
We have, 9 - 3=6 balls left.
Step - 2: Give 3 balls to the next boy.
We have, 6 - 3=3 balls left
Step - 3: Give 3 balls to the next boy.
We have, 3 - 3 =0
No balls left. We observed that, Three boys got the balls.
 Thus, 9 – 3 = 3
Multiplication and Division facts:
Thus, 9 – 3 = 3
Multiplication and Division facts:
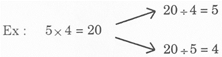 Basic terms used in division:
Basic terms used in division:

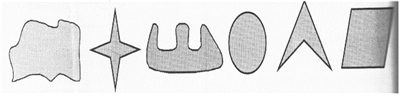
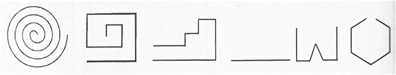
 Closed and Open figures:
Closed and Open figures:
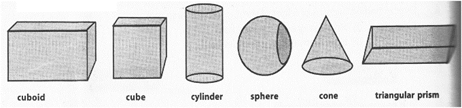
 Description of some basic shapes:
Description of some basic shapes:
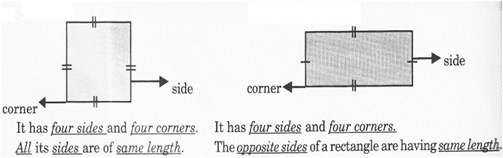 It has four sides and four corners.
All its sides are of same length.
It has four sides and four corners.
The opposite sides of a rectangle are having same length.
It has four sides and four corners.
All its sides are of same length.
It has four sides and four corners.
The opposite sides of a rectangle are having same length.
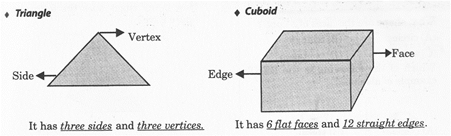
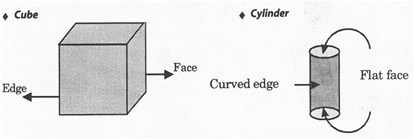 It has 6 flat faces and 12 straight edges.
It has 3 faces\[\to \] 1 curved face and 2 flat faces..
It has 2 cured edges.
It has 6 flat faces and 12 straight edges.
It has 3 faces\[\to \] 1 curved face and 2 flat faces..
It has 2 cured edges.
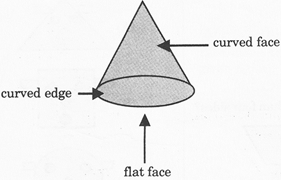 It has 2 faces \[\to \] 1 curved face and 1 flat face.
It has 1curued edge.
It has 2 faces \[\to \] 1 curved face and 1 flat face.
It has 1curued edge.
You need to login to perform this action.
You will be redirected in
3 sec
