| Quantity | Sign | Condition |
| \[\Delta Q\] | + | When heat is supplied to a system |
| ? | When heat is drawn from the system | |
| \[\Delta W\] | + | When work done by the gas (expansion) |
| ? | When work done on the gas (compression) | |
| \[\Delta U\] | + | With temperature rise, internal energy increases |
| ? | With temperature fall, internal energy decreases |
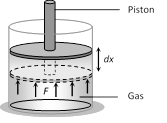 When the piston is pushed outward an infinitesimal distance dx, the work done by the gas \[dW=F.dx=P(A\,dx)=P\,dV\]
For a finite change in volume from \[{{V}_{i}}\] to \[{{V}_{f}}\]
Total amount of work done \[W=\int_{\,Vi}^{\,{{V}_{f}}}{P\,dV}=P({{V}_{f}}-{{V}_{i}})\]
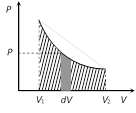
(i) If we draw indicator diagram, the area bounded by PV-graph and volume axis represents the work done
Work = Area \[=P({{V}_{2}}{{V}_{1}})\]
When the piston is pushed outward an infinitesimal distance dx, the work done by the gas \[dW=F.dx=P(A\,dx)=P\,dV\]
For a finite change in volume from \[{{V}_{i}}\] to \[{{V}_{f}}\]
Total amount of work done \[W=\int_{\,Vi}^{\,{{V}_{f}}}{P\,dV}=P({{V}_{f}}-{{V}_{i}})\]
(i) If we draw indicator diagram, the area bounded by PV-graph and volume axis represents the work done
Work = Area \[=P({{V}_{2}}{{V}_{1}})\]
 Work \[=\int_{\,{{V}_{1}}}^{{{V}_{2}}}{PdV}=P({{V}_{2}}-{{V}_{1}})\]
Work \[=\int_{\,{{V}_{1}}}^{{{V}_{2}}}{PdV}=P({{V}_{2}}-{{V}_{1}})\]
 Work = 0
Work = 0
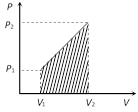
 Work = Area of the shown trapezium \[=\frac{1}{2}({{P}_{1}}+{{P}_{2}})\,({{V}_{2}}-{{V}_{1}})\]
Work = Area of the shown trapezium \[=\frac{1}{2}({{P}_{1}}+{{P}_{2}})\,({{V}_{2}}-{{V}_{1}})\]
 (ii) From \[\Delta W=P\Delta V=P({{V}_{f}}-{{V}_{i}})\]
If system expands against some external force then
\[{{V}_{f}}>{{V}_{i}}\] \[\Rightarrow \] \[\Delta W=\] positive
If system contracts because of external force then \[{{V}_{f}}
(ii) From \[\Delta W=P\Delta V=P({{V}_{f}}-{{V}_{i}})\]
If system expands against some external force then
\[{{V}_{f}}>{{V}_{i}}\] \[\Rightarrow \] \[\Delta W=\] positive
If system contracts because of external force then \[{{V}_{f}} 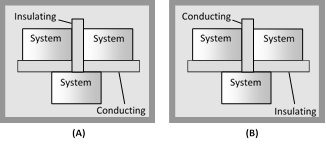 (1) The zeroth law leads to the concept of temperature. All bodies in thermal equilibrium must have a common property which has the same value for all of them. This property is called the temperature.
(2) The zeroth law came to light long after the first and seconds laws of thermodynamics had been discovered and numbered. It is so named because it logically precedes the first and second laws of thermodynamics.
(1) The zeroth law leads to the concept of temperature. All bodies in thermal equilibrium must have a common property which has the same value for all of them. This property is called the temperature.
(2) The zeroth law came to light long after the first and seconds laws of thermodynamics had been discovered and numbered. It is so named because it logically precedes the first and second laws of thermodynamics.  (iv) Thermodynamic system may be of three types
(a) Open system : It exchange both energy and matter with the surrounding.
(b) Closed system : It exchange only energy (not matter) with the surroundings. (c) Isolated system : It exchange neither energy nor matter with the surrounding.
(iv) Thermodynamic system may be of three types
(a) Open system : It exchange both energy and matter with the surrounding.
(b) Closed system : It exchange only energy (not matter) with the surroundings. (c) Isolated system : It exchange neither energy nor matter with the surrounding. 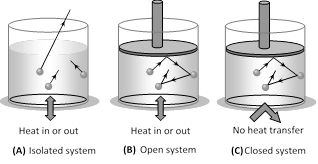 (2) Thermodynamic variables and equation of state : A thermodynamic system can be described by specifying its pressure, volume, temperature, internal energy and the number of moles. These parameters are called thermodynamic variables. The relation between the thermodynamic variables (P, V, T) of the system is called equation of state.
For \[\mu \] moles of an ideal gas, equation of state is \[PV=\mu RT\] and for 1 mole of an it ideal gas is PV = RT
(3) Thermodynamic equilibrium : In steady state thermodynamic variables are independent of time and the system is said to be in the state of thermodynamic equilibrium. For a system to be in thermodynamic equilibrium, the following conditions must be fulfilled.
(i) Mechanical equilibrium : There is no unbalanced force between the system and its surroundings.
(ii) Thermal equilibrium : There is a uniform temperature in all parts of the system and is same as that of surrounding.
(iii) Chemical equilibrium : There is a uniform chemical composition through out the system and the surrounding.
(4) Thermodynamic process : The process of change of state of a system involves change of thermodynamic variables such as pressure P, volume V and temperature T of the system. The process is known as thermodynamic process. Some important processes are
(i) Isothermal process : Temperature remain constant
(ii) Adiabatic process : No transfer of heat
(iii) Isobaric process : Pressure remains constant
(iv) Isochoric (isovolumic process) : Volume remains constant
(v) Cyclic and non-cyclic process : Incyclic process Initial and final states are same while in non-cyclic process these states are different.
(vi) Reversible and irreversible process :
(5) Indicator diagram : Whenever the state of a gas (P, V, T) is changed, we say the gaseous system is undergone a thermodynamic process. The graphical representation of the change in state of a gas by a thermodynamic process is called indicator diagram. Indicator diagram is plotted generally in pressure and volume of gas.
(2) Thermodynamic variables and equation of state : A thermodynamic system can be described by specifying its pressure, volume, temperature, internal energy and the number of moles. These parameters are called thermodynamic variables. The relation between the thermodynamic variables (P, V, T) of the system is called equation of state.
For \[\mu \] moles of an ideal gas, equation of state is \[PV=\mu RT\] and for 1 mole of an it ideal gas is PV = RT
(3) Thermodynamic equilibrium : In steady state thermodynamic variables are independent of time and the system is said to be in the state of thermodynamic equilibrium. For a system to be in thermodynamic equilibrium, the following conditions must be fulfilled.
(i) Mechanical equilibrium : There is no unbalanced force between the system and its surroundings.
(ii) Thermal equilibrium : There is a uniform temperature in all parts of the system and is same as that of surrounding.
(iii) Chemical equilibrium : There is a uniform chemical composition through out the system and the surrounding.
(4) Thermodynamic process : The process of change of state of a system involves change of thermodynamic variables such as pressure P, volume V and temperature T of the system. The process is known as thermodynamic process. Some important processes are
(i) Isothermal process : Temperature remain constant
(ii) Adiabatic process : No transfer of heat
(iii) Isobaric process : Pressure remains constant
(iv) Isochoric (isovolumic process) : Volume remains constant
(v) Cyclic and non-cyclic process : Incyclic process Initial and final states are same while in non-cyclic process these states are different.
(vi) Reversible and irreversible process :
(5) Indicator diagram : Whenever the state of a gas (P, V, T) is changed, we say the gaseous system is undergone a thermodynamic process. The graphical representation of the change in state of a gas by a thermodynamic process is called indicator diagram. Indicator diagram is plotted generally in pressure and volume of gas.  Thermodynamics is a branch of science which deals with exchange of heat energy between bodies and conversion of the heat energy into mechanical energy and vice-versa.
Thermodynamics is a branch of science which deals with exchange of heat energy between bodies and conversion of the heat energy into mechanical energy and vice-versa. You need to login to perform this action.
You will be redirected in
3 sec
